Highlights
-
CP violations and branching ratios for Bc+→ D(s)+π+π−(K+K−) from interference of the vector mesons in Perturbative QCD
2025, 49(9): 093108. doi: 10.1088/1674-1137/add523
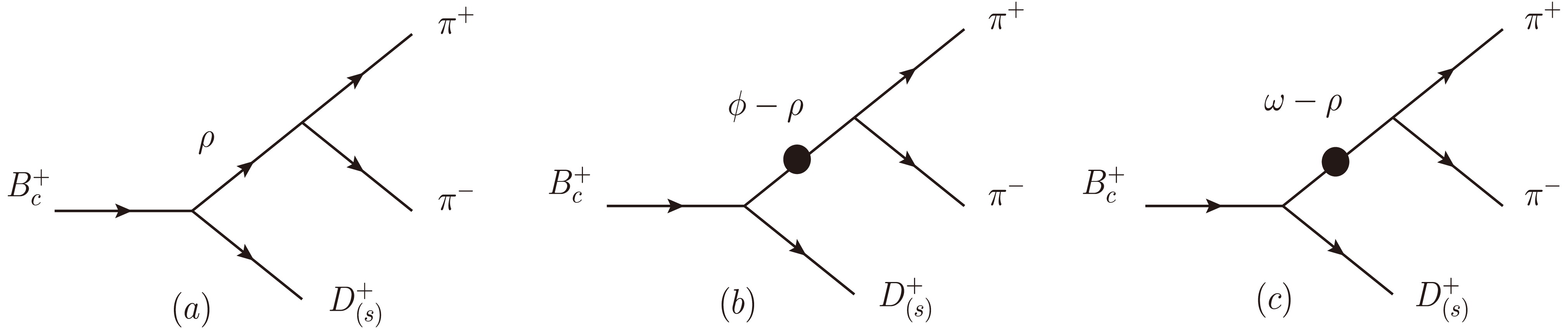 Within the framework of the perturbative QCD approach utilizing
Within the framework of the perturbative QCD approach utilizing$k_T$ factorization, we investigated the CP violation and branching ratios in the decay processes of$B_{c}^{+}\to D_{(s)} ^{+}V(V\rightarrow\pi^{+}\pi^{-})$ and$B_{c}^{+}\to D_{(s)}^{+}V(V\rightarrow K^{+}K^{-})$ , where V denotes the three vector mesons$\rho^0$ , ω, and ϕ. During the$V\to \pi^+\pi^-$ and$V\to K^+K^-$ decay processes, we incorporated the$\rho^{0}-\omega-\phi$ mixing mechanism to describe the amplitudes of these quasi-two-body decays. Within the interference region of the three vector particles, we observed distinct changes in both CP violations and branching ratios. Furthermore, our study presents evidence for local CP violations and branching ratios that warrants further experimental investigation. -
Predictions of unknown masses using a feedforward neural network
2025, 49(9): 094111. doi: 10.1088/1674-1137/add10a
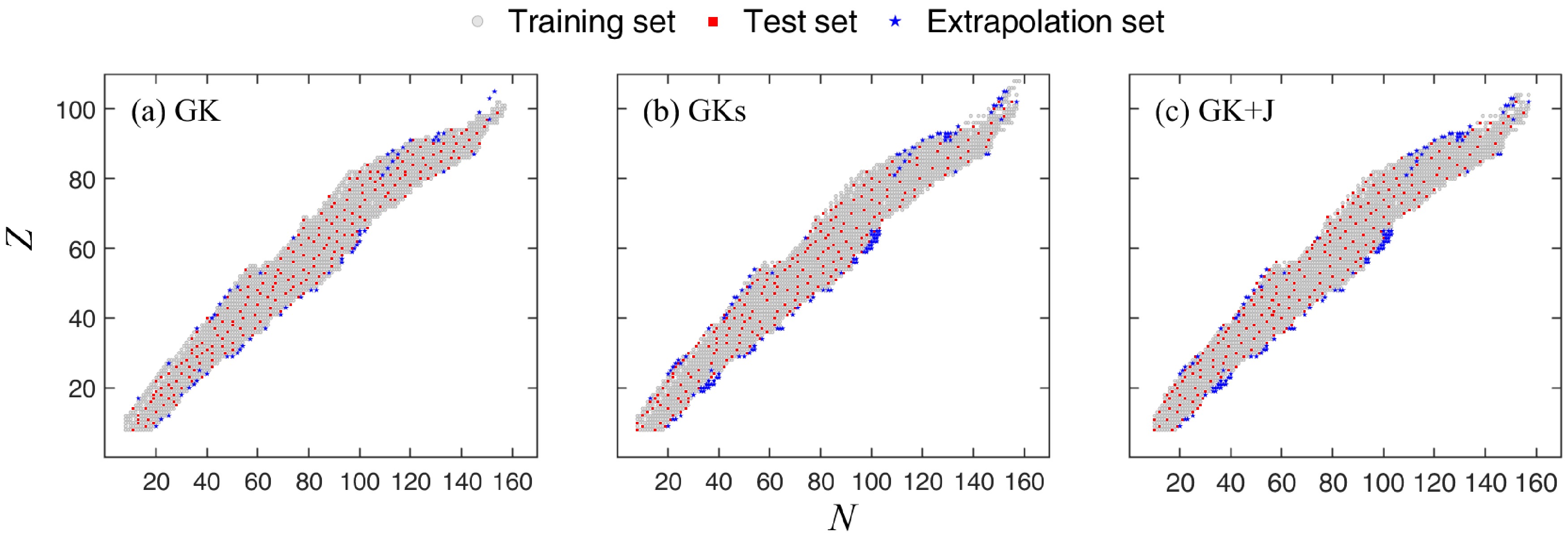 In this study, a feedforward neural network (FNN) approach is employed to optimize three local mass models (GK, GKs, and GK+J). We find that adding physical quantities related to the pairing effect in the input layer can effectively improve the prediction accuracy of local models. For the known masses in AME2012, the FNN reduces the root-mean-square deviation between theory and experiment for the three mass models by 11 keV, 32 keV, and 623 keV. Among them, the improvement effect of the light mass region with mass number between 16 and 60 is better than that of medium and heavy mass regions. The approach also has good optimization results when extrapolating AME2012 to AME2020 and the latest measured masses after AME2020. Based on the improved mass data, the separation energies for single- and two-proton (neutron) emissions and α-decay energies are obtained, which agree well with the experiment.
In this study, a feedforward neural network (FNN) approach is employed to optimize three local mass models (GK, GKs, and GK+J). We find that adding physical quantities related to the pairing effect in the input layer can effectively improve the prediction accuracy of local models. For the known masses in AME2012, the FNN reduces the root-mean-square deviation between theory and experiment for the three mass models by 11 keV, 32 keV, and 623 keV. Among them, the improvement effect of the light mass region with mass number between 16 and 60 is better than that of medium and heavy mass regions. The approach also has good optimization results when extrapolating AME2012 to AME2020 and the latest measured masses after AME2020. Based on the improved mass data, the separation energies for single- and two-proton (neutron) emissions and α-decay energies are obtained, which agree well with the experiment. -
A three-body form factor at sub-leading power in the high-energy limit: planar contributions
2025, 49(9): 093102. doi: 10.1088/1674-1137/addcc7
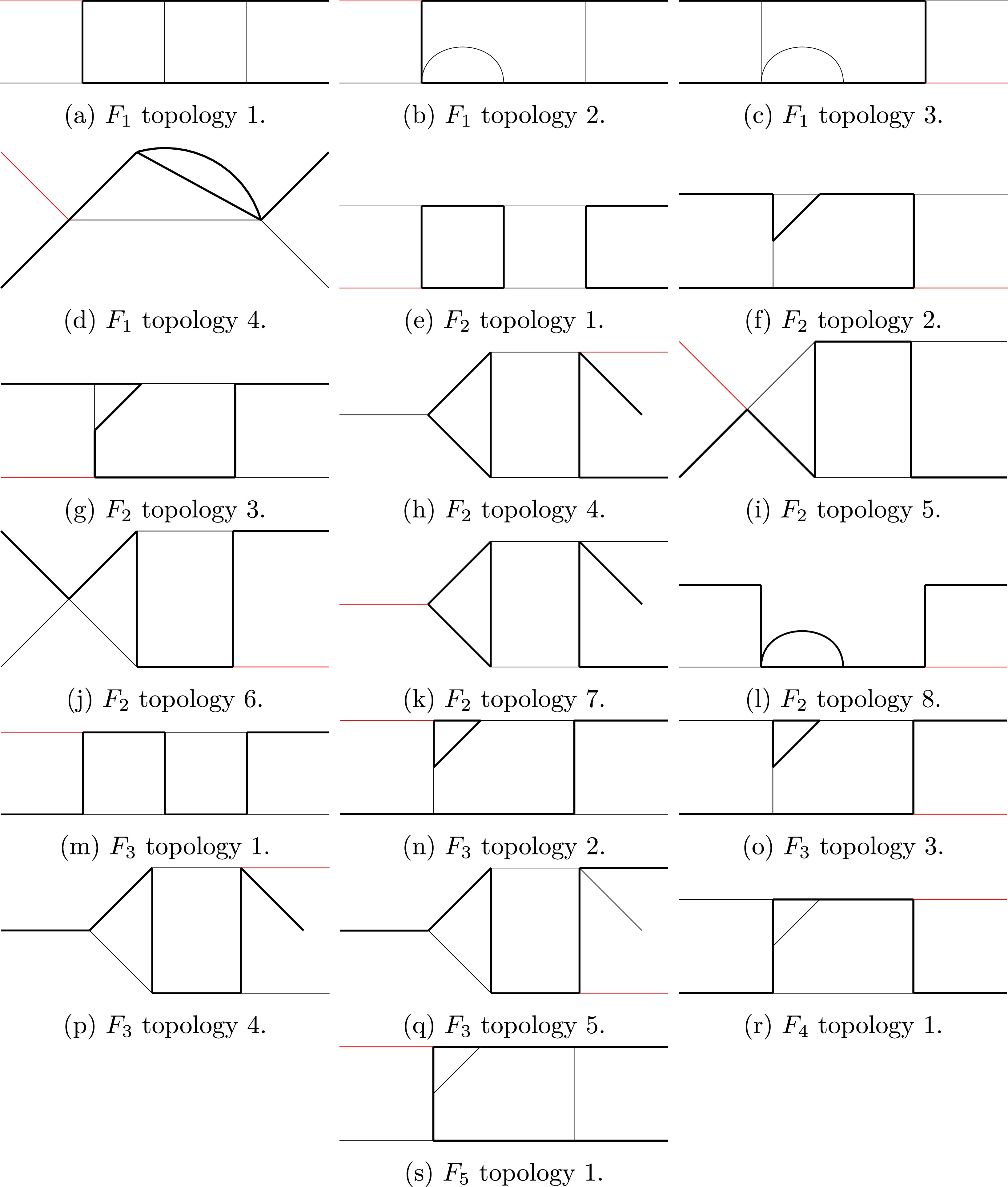 We analyzed two-loop planar contributions to a three-body form factor at next-to-leading power in the high-energy limit, where the masses of the external particles are much smaller than their energies. Calculations were performed by exploiting differential equations for the expansion coefficients, both to facilitate linear relations among them and to derive their analytic expressions. The results are expressed in terms of generalized polylogarithms involving a few simple symbol letters. Our method can readily be applied to calculations of non-planar contributions as well. Our results provide crucial information for establishing sub-leading factorization theorems for massive scattering amplitudes in the high-energy limit.
We analyzed two-loop planar contributions to a three-body form factor at next-to-leading power in the high-energy limit, where the masses of the external particles are much smaller than their energies. Calculations were performed by exploiting differential equations for the expansion coefficients, both to facilitate linear relations among them and to derive their analytic expressions. The results are expressed in terms of generalized polylogarithms involving a few simple symbol letters. Our method can readily be applied to calculations of non-planar contributions as well. Our results provide crucial information for establishing sub-leading factorization theorems for massive scattering amplitudes in the high-energy limit.
Just Accepted
More >
-
Unveiling the Inner Structure of the Pion’s First Excited State
Published: 2025-09-09, doi: 10.1088/1674-1137/adfc34
-
Correlation between Zero-Sound Modes and Nuclear Equation of State Stiffness Detected by Light Vector Boson
Published: 2025-09-08, doi: 10.1088/1674-1137/adfa81
-
Radiation properties of the accretion disk around a quantum-corrected black hole
Published: 2025-09-07, doi: 10.1088/1674-1137/adfa83
Recent
More >
-
Refinement of an analytical capture cross section formula
2025, 49(12): 124106-124106-8. doi: 10.1088/1674-1137/adfe53Show AbstractAn analytical formula with high accuracy is proposed for a systematic description of the capture cross sections at near-barrier energies from light to superheavy reaction systems. Based on the empirical barrier distribution method, three key input quantities are refined by introducing nuclear surface correction to the Coulomb parameter z for calculating the barrier height, incorporating the reaction Q-value and shell correction into the barrier distribution width calculations, and considering the deep inelastic scattering effects of superheavy systems on the barrier radius. With these refinements, the accuracy of not only the calculated barrier height but also the predicted capture cross sections is substantially improved. The average deviation (in logarithmic scale) between the predicted cross sections and the experimental data for 426 reaction systems with
$ 35 < Z_1 Z_2 < 2600 $ is sharply reduced from 3.485 to 0.113. -
Polarized neutron beams from polarized deuterium-tritium fusion with applications to magnetic field imaging in high-energy-density plasmas
2025, 49(12): 124102-124102-10. doi: 10.1088/1674-1137/adec4fShow AbstractWhen the spins of deuteron and triton are aligned in parallel, the fusion cross-section increases by approximately 50%. The emitted neutrons are anisotropic and polarized in specific directions. The polarized neutron beams can be used to measure strong magnetic fields in high-energy-density plasmas, offering a potential alternative to the well-established proton imaging technique. In contrast to protons, neutrons are not deflected by electromagnetic fields and are not sensitive to electric fields, thus reducing the complexity of magnetic field reconstruction. Three-dimensional spin transport hydrodynamics simulations are employed to investigate the polarized neutron beams generated from spin-polarized deuterium-tritium target implosions. Synthetic polarized neutron images of magnetic fields are generated from Monte Carlo simulations. Based on a comparison of the results of finite-size sources and an ideal point source, a method to compensate the finite-source-size blurring effect is proposed to reduce the error in magnetic field reconstruction.
-
$ D^*_{(s)}\to P $ form factors and their applications to semi-leptonic and non-leptonic weak decays2025, 49(12): 123104-123104-14. doi: 10.1088/1674-1137/adfb5dShow AbstractSimilar to other heavy flavor mesons, the weak decays of$ D^*_{(s)} $ mesons can provide a platform to verify the standard model, explore new physics, and understand the mechanisms of weak interactions. At present, the theoretical and experimental studies on$ D^*_{(s)} $ mesons are relatively limited. In addition to the dominant electromagnetic decays, the$ D^*_{(s)} $ weak decays should be feasible to explore the$ D^*_{(s)} $ mesons. In this study, we used the covariant light-front quark model to study the form factors of the transitions$ D^*_{(s)}\to \pi, K, \eta_{q, s} $ , and then calculated the branching ratios of the semi-leptonic decays$ D^*_{(s)}\to P\ell^{+}\nu_{\ell} $ and the non-leptonic decays$ D^*_{(s)}\to PP, PV $ with$ P=\pi, K, \eta^{(\prime)}, $ $ V=\rho, K^*, \phi $ , and$ \ell=e, \mu $ . The channels$ D_{s}^{*+}\to\eta \ell^{+}\nu_{\ell} $ and$ D^{*+}_{s}\to \eta\rho^{+} $ possess the largest branching ratios, which can reach anorder of $ 10^{-6} $ among these decays, and are most likely to be accessible in experiments at future high-luminosity colliders. Furthermore, we predict and discuss the longitudinal polarization fraction$ f_{\rm L} $ and the forward-backward asymmetry$ A_{\rm FB} $ for the considered semi-leptonic$ D^*_{(s)} $ decays.
Archive
ISSN 1674-1137 CN 11-5641/O4
Original research articles, Ietters and reviews Covering theory and experiments in the fieids of
- Particle physics
- Nuclear physics
- Particle and nuclear astrophysics
- Cosmology
Author benefits
- A SCOAP3 participating journal - free Open Access publication for qualifying articles
- Average 24 days to first decision
- Fast-track publication for selected articles
- Subscriptions at over 3000 institutions worldwide
- Free English editing on all accepted articles
News
- Chinese Physics C Outstanding Reviewer Award 2023
- Impact factor of Chinese Physics C is 3.6 in 2022
- 2022 CPC Outstanding Reviewer Awards
- The 2023 Chinese New Year-Office closure
- 《Chinese Physics C》BEST PAPER AWARDS 2022
Cover Story
- Cover Story (Issue 9, 2025): Precise measurement of χc0 resonance parameters and branching fractions of χc0,c2→π+π-/ K+K-
- Cover Story (Issue 8, 2025) A Novel Perspective on Spacetime Perturbations: Bridging Riemannian and Teleparallel Frameworks
- Cover Story (Issue 7, 2025) Evidence of the negative parity linear chain states in 16C
- Cover Story (Issue 1, 2025) Comments on Prediction of Energy Resolution inthe JUNO Experiment
- Cover Story (Issue 12, 2024) | Doubly heavy meson puzzle: precise prediction of the mass spectra and hadronic decay with coupled channel effects to hunt for beauty-charm family