R-Symmetric NMSSM

In the context of supersymmetry (SUSY), the minimal supersymmetric standard model (MSSM) is not favored by the observed Higgs mass [1, 2] if naturalness is considered. The naturalness problem can be relieved by the addition of a standard model (SM) singlet, which directly couples to two Higgs doublets in the MSSM. Compared to the MSSM, in this singlet extended model known as the next-to-minimal supersymmetric standard model (NMSSM), the observed Higgs mass [3–8] is easily explained in terms of new tree-level contributions instead of large loop corrections. Apart from the Higgs mass issue, the conventional NMSSM has distinctive features, such as a relaxed constraint on neutralino dark matter from dark matter direct detection [9–11], and even enables us to address baryon asymmetry by means of strong first-order phase transition [12–14].

In fact, without any violation of the success of Higgs mass naturalness, the conventional NMSSM can be modified in several well-motivated ways, the new phenomenological outcomes of which may be more favored by current experiments. Recently, Ref. [15] considered the NMSSM extended by gauged $ U(1)_{B-L} $ symmetry, where gluinos and neutralinos are still Majorana fermions. As in earlier studies [16, 17], in this study, we focus on the NMSSM extended by an unbroken $ U(1)_R $ global symmetry, referred to as the RNMSSM, where gluinos and neutralinos are instead Dirac fermions. Because of $ U(1)_R $ symmetry, we show that the Kahler potential and superpotential in the RNMSSM change as follows:

where $ K_{{\rm{MSSM}}} $ and $ W_{{\rm{MSSM}}} $ denote the Kahler potential and superpotential in the MSSM, respectively, N is the singlet, and $ H_{u} $and $ H_d $ are two Higgs doublets. Accordingly, we also expect the soft terms in $ \mathcal{L}_{{\rm{soft}}} $ to be altered.

The main goal of this study is to uncover the explicit forms of $ \delta K $ and $ \delta W $ from the perspective of both simplicity and viability, where "simplicity" means that we seek a minimal extension on the matter content, whereas "viability" requires that the RNMSSM be consistent with current experimental bounds. In Sec. II, we highlight the R charge assignments to accommodate $ U(1)_R $ symmetry. In Sec. III, we first infer the structure of the minimal RNMSSM and then discuss the main features of the supersymmetric mass spectrum and phenomenological implications to gauginos, charginos, neutralinos, and sfermions. Finally, we conclude with Sec. IV.

To be consistent with $ U(1)_R $ symmetry, the R charges of several matter fields should be assigned as follows:

● The R charges of Higgs doublets must be zero to ensure an unbroken R symmetry after electroweak symmetry breaking. This R charge assignment makes our model different from that in Ref. [16], where R symmetry is broken by the vacuum expectation values (vevs) of the Higgs doublets.

● The R charge of N should be two from the Yukawa interaction $ W\supset NH_{u}H_{d} $ in Eq. (2), and the vev of this scalar field should also be zero, that is, $ \left<N\right>=0 $.

● The R charges of SM gauge superfields $ W^{i}_{\alpha} $ ($ i=1-3 $) must be one^①, which ensures that all of the SM gauge fields are neutral under $ U(1)_R $.

From the R charge assignments above, one can infer the R charges of all matter superfields from Eq. (2), which are explicitly shown in Table 1. They imply that none of the SM fields carry the R charge.

$ {\rm{Field}} $	$({S U}(3)_{c}, {S U}(2)_{L})_{U(1)_{Y}}$	${U}(1)_{R}$
Q	$ (3,2)_{1/6} $	$ 1 $
$ \bar{u} $	$ (\bar{3},1)_{-2/3} $	$ 1 $
$ \bar{d} $	$ (\bar{3},1)_{1/3} $	$ 1 $
L	$ (1,2)_{-1/2} $	$ 1 $
$ \bar{e} $	$ (1,1)_{1} $	$ 1 $
$ H_{u} $	$ (1,2)_{1/2} $	$ 0 $
$ H_{d} $	$ (1,2)_{-1/2} $	$ 0 $
N	$ (1,1)_{0} $	$ 2 $
$ \sigma_{1} $	$ (1, 1)_{0} $	$ 0 $
$ \sigma_{3} $	$ (1, 3)_{0} $	$ 0 $
T	$ (1,3)_{0} $	$ 2 $
$ \sigma_{8} $	$ (8,1)_{0} $	$ 0 $

Table 1.  Matter content and R charge assignments in the minimal RNMSSM, where $ \sigma_1 $, $ \sigma_{3} $, T, and $ \sigma_8 $ refer to an $S U(2)_L$ singlet, two $S U(2)_L$ triplets, and an $S U(3)_c$ octet states, respectively. The triplets (octet) make the charginos and neutralinos (gluino) Dirac fermions. See Sec. III for details.

In this section, we discuss the phenomenological features in the minimal RNMSSM.

With unbroken R symmetry, gauginos such as binos $ \tilde{B} $, winos $ \tilde{W} $, and gluinos $ \tilde{g} $ must be Dirac fermions [18, 19] instead of Majorana fermions, which couple to the fermion components $ \tilde{\sigma}_{i} $ in the chiral adjoint superfields $ \sigma_i $ as

From Eq. (3), the R charges of these chiral superfields are found to be zero, as shown in Table 1. The input values of the gaugino masses $ m_i $ in Eq. (3) are read from soft SUSY-breaking operators such as

where X represents the SUSY-breaking sector^②, and M denotes the scale at which the SUSY-breaking effect is mediated to the RNMSSM. Eq. (4) is consistent with the SM gauge symmetries and R symmetry shown in Table 1, where the first and second yield the one-loop Dirac gaugino masses $ m_{i}\sim F/M $ and the two-loop scalar soft mass squared $ m^{2}_{\sigma_{i}}\sim F^{2}/M^{2} $ at the input scale M, respectively.

Similar to a Majorana analogy, the Dirac gluino can be directly produced at hadron colliders. Because the production cross section of the Dirac gluino not only depends on the gluino mass $ m_3 $ but also squark mass parameters, we are unable to constrain $ m_3 $ in model-independent ways. For discussions on the constraints from current LHC and future collider experiments, see Refs. [23–25, 26], respectively.

Without the $S U(2)_L$ triplet T shown in Table 1, the determinant of the charged chargino mass matrix vanishes owing to the unbroken R symmetry. This mass issue can be resolved by an extension of the matter content. The simplest way to achieve this is via the addition of the triplet T [16], which couples to the Higgs doublets as

Equation (5) suggests that T has an R charge of $ +2 $. With the quantum numbers of T, one can infer its soft masses from the SUSY-breaking operators,

where the first and second terms give rise to the triplet scalar mass squared $ m^{2}_{T}\sim F^{2}/M^{2} $ and a new Dirac mass parameter $ m'_{2} $ similar to $ m_2 $ in Eq. (3), respectively.

To explicitly write the Dirac chargino and neutralino mass matrices, it is convenient to divide the triplet fields $ \sigma_{3} $ in Eq. (3) and T in Eq. (5) into the neutral fields $ \sigma^{0}_{3} $ and $ T^{0} $ and the charged fields $ \sigma^{\pm}_{3} $ and $ T^{\pm} $, respectively. With the new superpotential term in Eq. (5) taken into account, the chargino and neutralino mass matrices are now given as

respectively, where $ \theta_W $ is the weak mixing angle, the vevs of the two Higgs doublets satisfy $\upsilon^{2}_{u}+\upsilon^{2}_{d}=\upsilon^{2}= (174\; {\rm{GeV}})^{2}$ with $ \tan\beta=\upsilon_{u}/\upsilon_{d} $, and we simply assume the singlet vevs

Although nonzero $ \left<\sigma^{0}_{1}\right> $ and $ \left<\sigma^{0}_{3}\right> $ are allowed by R symmetry, they make the analysis of electroweak symmetry breaking more complicated than with the values we choose.

In the Dirac chargino mass spectrum given by Eq. (7), the lightest mass $ m_{\chi^{\pm}_{1}} $ is sensitive to the triplet T in the sense that it vanishes as T disappears, that is, $ y_{T}\rightarrow 0 $ and $ m'_{2}\rightarrow 0 $. This must be avoided because $ m_{\chi^{\pm}_{1}} $ must be larger than $ \sim 103.5 $ GeV from the previous LEP-2 limit [27, 28]. Improved bounds on $ m_{\chi^{\pm}_{1}} $ inferred from current LHC data [29, 30] are sensitive to the $ \chi^{\pm}_{1} $ decay pattern because many choices exist owing to the wide range of the lightest neutralino mass $ m_{\chi^{0}_{1}} $ in Eq. (8). In the case of $ \chi^{\pm}_{1}\rightarrow \chi^{0}_{1}+W^{\pm} $, $ m_{\chi^{\pm}_{1}} $ is excluded up to $ 600-700 $ GeV for massless $ \chi^{0}_{1} $ [29]. If the mass difference between $ m_{\chi^{\pm}_{1}} $ and $ m_{\chi^{0}_{1}} $ is small, $ \chi^{\pm}_{1} $ decay is dominated by either $\chi^{\pm}_{1}\rightarrow \chi^{0}_{1}W^{*}\rightarrow \chi^{0}_{1} f\bar{f}'$ or $ \chi^{\pm}_{1}\rightarrow W\tilde{G} $, where f and $ f' $ refer to SM fermions. In the former situation, the chargino mass bound is greatly relaxed, whereas in the latter case, $ m_{\chi^{\pm}_{1}} $ must be larger than $ \sim 200 $ GeV [31] for a light gravitino with a mass of the order of $ \sim 10 $ eV as the lightest supersymmetric particle (LSP). To summarize, because $ m_{\chi^{\pm}_{1}} $ in our model is less than $ \sim 200 $ GeV owing to a moderate value of $ y_T $, the small mass splitting between $ m_{\chi^{\pm}_{1}} $ and $ m_{\chi^{0}_{1}} $, with $ \chi^{0}_{1} $ as the LSP, is favored in light of current collider limits.

To illustrate the viability of $ m_{\chi^{0}_{1}}< m_{\chi^{\pm}_{1}} <m_{\chi^{0}_{1}}+m_{W} $ in Eqs. (7) and (8), we show in Fig. 1 the samples that yield the required mass spectrum with the parameter ranges shown in Table 2 in comparison with regions excluded by the LEP-2 limit (in gray) and disfavored by current LHC data (in red). We show the explicit dependences of the mass spectrum on the model parameters $ \tan\beta $ (left), $ y_T $ (middle), and $ m_1 $ (right). This indicates that small $ m_{\chi^{0}_{1}} $ of the order of less than $ \sim 40 $ GeV favors both large $ y_{T}\geq 0.9 $ and small $ \tan\beta \leq 5 $, whereas moderate $ m_{\chi^{0}_{1}} $ of the order of $ \sim 80-100 $ GeV has no specific preferences. In particular, the right plot in Fig. 1 implies that bino-like neutralino dark matter (with small $ \mid m_1\mid $) can be achieved in the case of either small or moderate $ m_{\chi^{0}_{1}} $.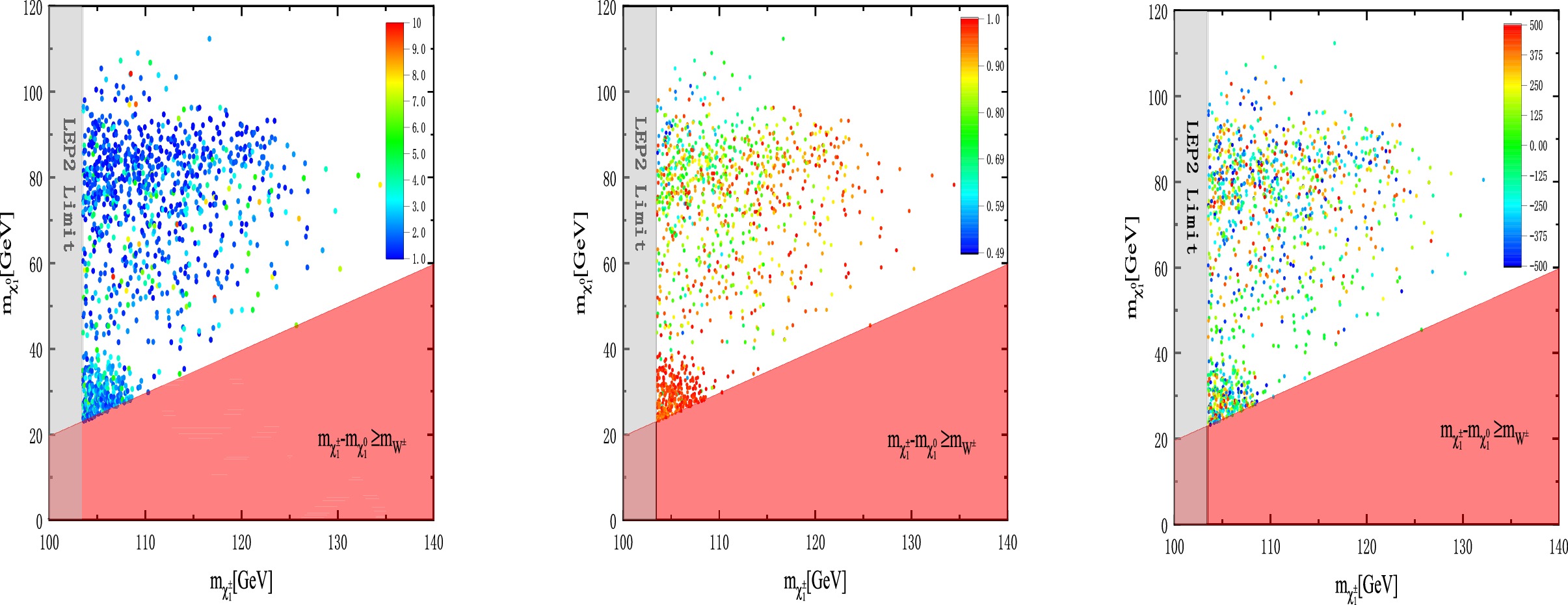

Figure 1.  (color online) Mass spectrum of $ m_{\chi^{\pm}_{1}} $ and $ m_{\chi^{0}_{1}} $ with $ m_{\chi^{0}_{1}}< m_{\chi^{\pm}_{1}} <m_{\chi^{0}_{1}}+m_{W} $ in the parameter ranges shown in Table 2, where the dependences of the mass spectrum on $ \tan\beta $, $ y_T $, and $ m_1 $ are highlighted in the left, middle, and right plots, respectively. Regions excluded by the LEP-2 limit (in gray) and disfavored by current LHC data (in red) are also shown.

${\rm{Parameter} }$	${\rm{Range} }$
$ m_1 $	$ [-490.8, 490.3] $
$ m_2 $	$ [-137.6, 157.1] $
$ m'_2 $	$ [-430, 409] $
$ \tan\beta $	$ (1, 10] $
λ	$ [0.5, 0.7] $
$ y_T $	$ [0.49, 1.0] $

Table 2.  Parameter ranges in the Dirac chargino-neutralino sector, which are consistent with the LEP-2 bound on $ m_{\chi^{\pm}_{1}} $ and favored by current LHC data. Masses are in units of GeV.

Now, we turn to the subject of Dirac neutralino dark matter $ \chi^{0}_1 $. Because $ \chi^{0}_1 $ in Eq. (8) can be either bino- or singlino-like, we discuss them separately. If $ \chi^{0}_1 $ is singlino-like, the annihilation cross section of a $ \chi^{0}_1 $ pair is mainly through an exchange of Higgs and heavier neutralino states in Eq. (8). Because $ m_{\chi^{0}_{1}} $ in Fig. 1 is less than the Higgs mass $ m_h $, the latter annihilation channel is kinetically forbidden. Such Higgs-portal dark matter [32] is excluded by a combination of invisible Higgs decay in the mass region $ m_{\chi^{0}_{1}}<m_{h}/2 $ and spin-independent direct detection limits in the mass region $ m_{\chi^{0}_{1}}>m_{h}/2 $, except a narrow resonant region $ m_{\chi^{0}_{1}}\approx m_{h}/2 $.

Unlike a Majorana neutralino or Higgs-portal dark matter as above, the annihilation cross sections of a bino-like $ \chi^{0}_1 $ (namely the $ \tilde{B}^0-\tilde{\sigma}_{1}^{0} $ state) pair into SM fermions is no longer suppressed by the SM fermion masses [33–36], which makes the lightest Dirac neutralino a natural realization of leptophilic dark matter [37]. In the situation with squark masses larger than slepton masses, the annihilation of the $ \chi^{0}_1 $ pair is dominated by lepton final states [36]

where $ g' $ is the $ U(1)_Y $ gauge coupling constant, $ \upsilon_{{\rm{rel}}} $ is the dark matter relative velocity, $ \ell=\{\tau,\mu, e\} $, and $ m_{\tilde{l}_{L}} $ and $ m_{\tilde{l}_{R}} $ refer to the left- and right-hand slepton masses, respectively. Note that R symmetry forbids mixing effects between the left- and right-hand sleptons.

Figure 2 shows the contour (in black) of the bino-like $ \chi^{0}_{1} $ projected onto the two-parameter plane of $ m_{\chi^{0}_{1}} $ and the right-hand smuon and stau mass $ m_{\tilde{\ell}_{R}} $ with $ \ell=\{\mu,\tau\} $, which satisfies the dark matter annihilation cross section $\sigma\upsilon_{{\rm{rel}}}\approx 3\times 10^{-26}\;{\rm{cm}}^{3}{\rm{s}}^{-1}$ from the observed thermal dark matter relic density. We take the selectron masses $ m_{\tilde{e}_{L}}=m_{\tilde{e}_{R}}=300 $ GeV, consistent with the AMS limit on $ \sigma_{e^{+}e^{-}}\upsilon_{{\rm{rel}}} $ [38] in the mass range $ m_{\chi^{0}_{1}}\leq 100 $ GeV, and adopt the left-hand smuon and stau masses $ m_{\tilde{\mu}_{L}}=m_{\tilde{\tau}_{L}}=400 $ GeV in light of LEP [39] and current LHC [30, 40] bounds.^③ This figure shows that in comparison with the LHC bounds and the AMS limits on $ \sigma_{\mu^{+}\mu^{-}}\upsilon_{{\rm{rel}}} $ and $ \sigma_{\tau^{+}\tau^{-}}\upsilon_{{\rm{rel}}} $ [38, 42], $ m_{\chi^{0}_{1}} $ between $ 70 $ GeV and $ 100 $ GeV survives with $ m_{\tilde{\ell}_{R}}\geq 250 $ GeV.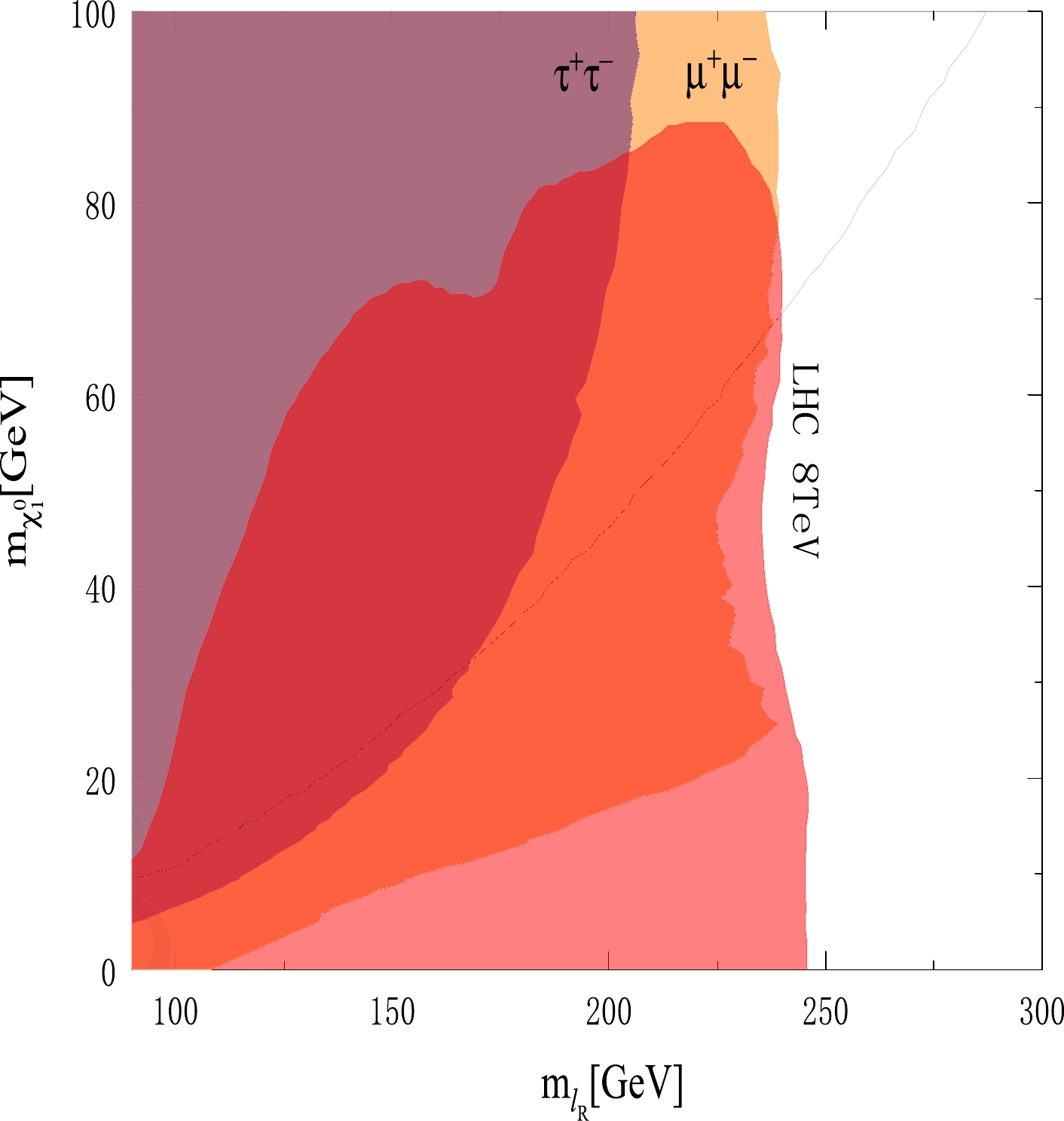

Figure 2.  (color online) Contour (in black) of bino-like neutralino dark matter is projected onto the two-parameter plane of $ m_{\chi^{0}_1} $ and the right-hand slepton masses $ m_{\tilde{\ell}_{R}} $ with $ \ell=\{\mu,\tau\} $ for selectron masses $ m_{\tilde{e}_{L}}=m_{\tilde{e}_{R}}=300 $ GeV and left-hand smuon and stau masses $ m_{\tilde{\mu}_{L}}=m_{\tilde{\tau}_{L}}=400 $ GeV, in comparison with regions excluded by the 8-TeV LHC limit (in red), AMS limits on $ \sigma_{e^{+}e^{-}}\upsilon_{{\rm{rel}}} $ and $ \sigma_{\mu^{+}\mu^{-}}\upsilon_{{\rm{rel}}} $ (in orange), and Fermi-LAT limit on $ \sigma_{\tau^{+}\tau^{-}}\upsilon_{{\rm{rel}}} $ (in purple).

Compared to the R-symmetric MSSM (RMSSM) [43–47] or conventional NMSSM, the scalar mass spectrum of the Higgs sector in the minimal RNMSSM is different. Let us begin with relevant soft masses in our model.

where the first three terms arise from the following soft SUSY-breaking operators:

In Eq. (12), two-loop $ m^{2}_{N}\sim m^{2}_{H_{u}}\sim m^{2}_{H_{d}} \sim F^{2}/M^{2} $ are produced at the input scale M. Combining the gauge interactions in Eq. (1), the F terms in Eq. (2), and the soft masses in Eq. (11) gives rise to the scalar mass spectrum in this sector as shown below.

First, the mass squared matrices for CP-even scalars with R charges of $ 0 $ and $ 2 $ are given by

and

respectively, where the soft masses $ m^{2}_{H_{u}} $ and $ m^{2}_{H_{d}} $ are eliminated by the b term because of the conditions of electroweak symmetry breaking.

Second, the mass squared matrices for the CP-odd scalars are

which contain a massless Goldstone mode, and

Third, the mass squared matrix for CP-charged scalars under the basis $ (H^{+}_{u}, H^{-*}_{d}) $ is given by

with $ m^{2}_{T^{\pm}}\approx m^{2}_{T}+m'^{2}_{2}+y^{2}_{T}\upsilon^{2} $, where $ g_{2} $ is the $S U(2)_L$ gauge coupling constant. From Eq. (17), one obtains a massless Goldstone mode and a massive charged state $ H^{\pm} $. Compared to the NMSSM, the new $ y_T $ term in Eq. (17) is useful in uplifting the charged Higgs scalar mass.

The scalars in Eqs. (13)–(16) can be classified into two sets, one set similar to type-II Higgs doublets in the MSSM and the other set controlled by free parameters, such as $ m_N $ and $ m_{T} $. In the former set, the mass relations are altered; at the tree level, these rely on four input parameters, b, λ, $ y_T $, and $ \tan\beta $. One can replace the dimensional parameter b by imposing the constraint $ m_{h_{1}}=125 $ GeV and then uncover other masses such as $ m_{h_{2}} $, $ m_A $, and $ m_{H^{\pm}} $ by adjusting the three dimensionless parameters.

To date, the most stringent constraint on these scalars arose from precision measurements of $ B\rightarrow X_{s}\gamma $ [48–50]. Figure 3 shows the masses $ m_{h_{2}} $ (hollow) and $ m_A $ (solid) as functions of the CP-charged scalar mass $ m_{H^{\pm}} $ for the parameter ranges shown in Table 3, which satisfy the observed Higgs mass and excess the conservative mass bound $ m_{H^{\pm}}\geq 480 $ GeV [49] simultaneously.^④ The explicit dependences of the mass spectrum on $ \tan\beta $, $ y_T $, and b are shown in the left, middle, and right plots, respectively. The lower mass bound implies that both $ m_{h_{2}} $ and $ m_{A} $ must exceed $ \sim 450 $ GeV. For future prospects regarding the detection of these scalars at the LHC, see, for example, Ref. [51]. Note that Eq. (13) is a tree-level estimate for the observed Higgs mass $ m_{h_{1}} $. It is valid when the loop correction is small, which is a natural assumption in our case.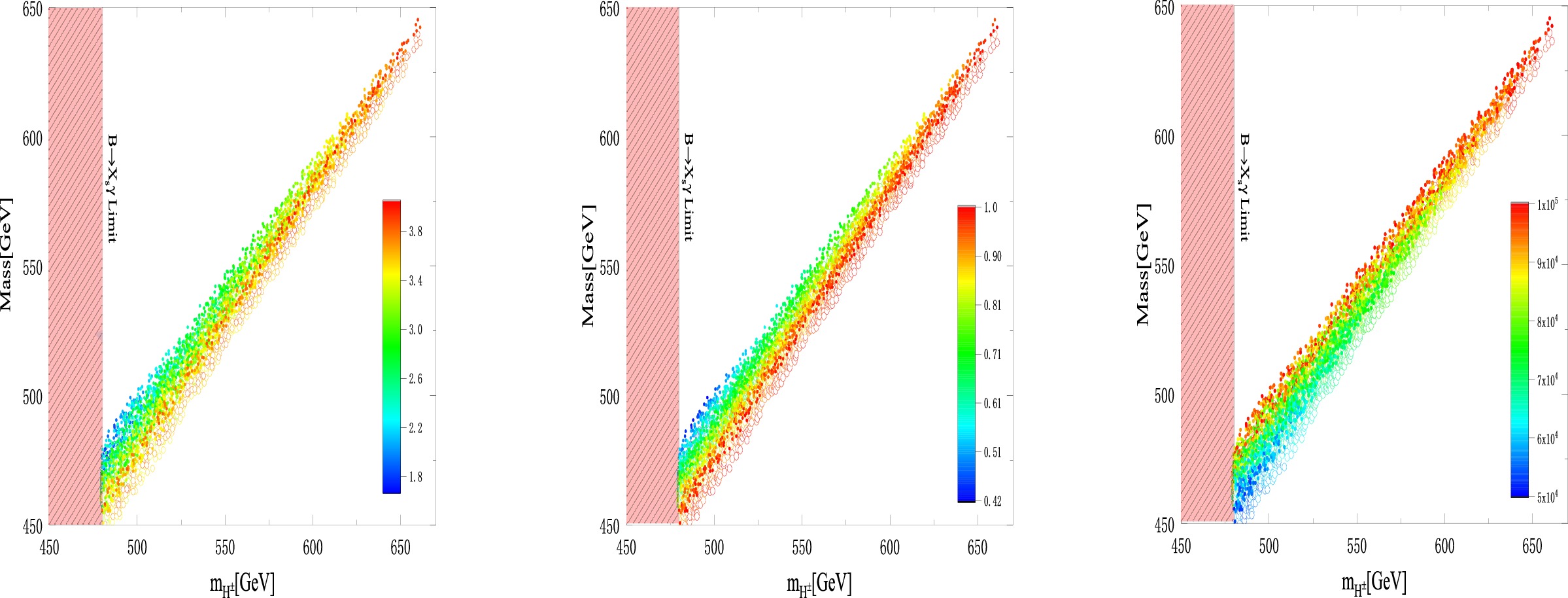

Figure 3.  (color online) Masses $ m_{h_{2}} $ (hollow) and $ m_A $ (solid) as functions of the CP-charged scalar mass $ m_{H^{\pm}} $, with $ m_{h_{1}}=125 $ GeV in the parameter ranges shown in Table 3, where the explicit dependences of the mass spectrum on $ \tan\beta $, $ y_T $, and b are shown in the left, middle, and right plots, respectively.

${\rm{Parameter} }$	${\rm{Range} }$
b	$[5\times 10^{4}, 10^{5}] \; {\rm{GeV} }^{2}$
$ \tan\beta $	$ [1.6, 4.0] $
λ	$ [0.5, 0.7] $
$ y_{T} $	$ [0.42, 1.0] $

Table 3.  Adopted parameter ranges for the numerical analysis in Fig. 3.

Let us now estimate the fine tuning related to the natural argument of Higgs mass. We take the measure of the fine tuning as $ \Delta=\max\{\Delta_P\} $, with

where the soft mass squared $ P=\{m^{2}_{i}, b, \cdots\} $. Among the aforementioned soft masses, the dimensional b in Table 3 contributes to $ \Delta_{b}\sim \tan\beta(b/ 4m^{2}_{Z}) \leq 12.5 $.

Apart from Majorana gaugino masses, R symmetry also prohibits holomorphic soft masses, such as A terms related to sfermions. It only allows scalar soft masses

which arise from soft SUSY-breaking operators such as

From Eq. (20), the two-loop $ m^{2}_{\tilde{f}}\sim F^{2}/M^{2} $ is generated at the scale M.

The absence of A terms earns us a few advantages. The vanishing A term associated with the top quark suggests that the observed Higgs mass cannot be explained without a violation of naturalness, which is true for the typical RMSSM but does not stand in the context of the RNMSSM. This is because, similar to the NMSSM, there is a large tree-level correction to the Higgs mass in this model. Moreover, flavor violations, which are too large to violate stringent experimental bounds on the MSSM with large top-related A terms, are naturally small in the RNMSSM.

In this study, we propose a new variant of the NMSSM by imposing unbroken R symmetry. Compared to well-known SUSY models such as the MSSM, NMSSM, and RMSSM, the RNMSSM introduces distinctive phenomenological features, as shown in Table 4. These features make it a high-motivation scenario that deserves investigation. We identify the minimal version of the RNMSSM from the principles of both simplicity and viability. Our example, which contains an SM singlet, two $S U(2)_L$ triplets, and an $S U(3)_c$ octet beyond the matter content of the NMSSM, indicates the following:

Model	Higgs naturalness problem	µ problem	Flavor violation	Neutralino dark matter
$ {\rm{MSSM}} $	$ {\rm{yes}} $	$ {\rm{yes}} $	$ {\rm{yes}} $	$ {\rm{Majorana}} $
$ {\rm{NMSSM}} $	$ {\rm{no}} $	$ {\rm{no}} $	$ {\rm{yes}} $	$ {\rm{Majorana}} $
$ {\rm{RMSSM}} $	$ {\rm{yes}} $	$ {\rm{no}} $	$ {\rm{no}} $	$ {\rm{Dirac}} $
$ {\rm{RNMSSM}} $	$ {\rm{no}} $	$ {\rm{no}} $	$ {\rm{no}} $	$ {\rm{Dirac}} $

Table 4.  Summary of the main phenomenological features in the RNMSSM compared to those of the MSSM, NMSSM, and RMSSM.

● The μ problem is resolved by identifying the neutralinos as Dirac fermions. In this model, two $ SU(2)_L $ triplets are introduced to illustrate the viability of the idea.

● The lightest neutralino mass is typically less than $ \sim 100 $ GeV, which can realize bino-like dark matter with the main annihilations dominated by $ \tau^{+}\tau^{-} $ and $ \mu^{+}\mu^{-} $. Much of the surviving parameter space in Fig. 2 can be probed in future LHC experiments via $ pp\rightarrow $ multi – leptons + missing energy, dark matter direct detection, such as LZ [52], or dark matter indirect detection, such as Planck [53].

● The Higgs mass naturalness is resolved in terms of both the singlet and triplet, which simultaneously contribute to the tree-level Higgs mass. Meanwhile, they help the charged Higgs mass exceed the lower bound value from the stringent $ B\rightarrow X_{s}\gamma $ limit.

● Owing to the absence of A terms, flavor violations are naturally small.

Finally, although not addressed here, there is no obstacle to finding an explicit SUSY-breaking sector^⑤ that yields the soft Lagrangian $ \mathcal{L}_{{\rm{soft}}} $, as we show above.