-
Ladder operators can provide us deep insights into the characteristics of a system. In quantum mechanics, ladder operators relate families of solutions of the Schrödinger equation without requiring detailed knowledge of the solutions. When investigating the massive Klein-Gordon equation in curved spacetime, Cardoso et al. constructed generalized ladder operators for massive Klein-Gordon scalar fields in spacetimes with the conformal symmetry and showed that these operators can map a solution of the massive Klein-Gordon equation into that with different mass squared values [1, 2]. Thus, these operators are called mass ladder operators. In Ref. [3], Mück used a scalar curvature term to replace the mass squared term and built generalized ladder operators for the Klein-Gordon equation with a scalar curvature term. More recently, Katagiri and Kimura used ladder operators to investigate the quasinormal modes (QNMs) of a massive Klein-Gordon field in Bañados-Teitelboim-Zanelli (BTZ) spacetime, demonstrating that ladder operators can change the indices of QNM overtones under different boundary conditions [4].
The aforementioned studies on ladder operators focused only on Einstein gravity. It is of significant interest to generalize the investigation of ladder operators to Einstein-bumblebee gravity as it is a simple and effective theory involving Lorentz violation [5]. In the bumblebee model, Lorentz violation results from the dynamics of the bumblebee field, i.e., a single vector or axial-vector field
$ B_\mu $ . In 2018, Casana and Cavalcante obtained an exact Schwarzschild-like black hole solution for this Einstein-bumblebee gravity and investigated some classical tests, including the advance of the perihelion, bending of light, and Shapiro's time-delay [6]. In 2020, Ding et al. obtained an exact Kerr-like solution and investigated the shadow of this Kerr-like black hole [7]. For a high dimensional spacetime, the authors of Ref. [8] obtained an exact AdS-like black hole solution and investigated the thermodynamics and phase transitions of this high dimensional Schwarzschild-AdS-like black hole. For three-dimensional spacetime, Ding et al. obtained an exact rotating BTZ-like black hole solution in the Einstein-bumblebee gravity theory and the central charges of the dual conformal field theory (CFT) on the boundary using the thermodynamic method [9]. Subsequently, Chen et al. investigated the quasinormal modes of a scalar perturbation around this rotating BTZ-like black hole in Einstein-bumblebee gravity and observed that the parameter breaking Lorentz symmetry imprints only in the imaginary parts of the quasinormal frequencies [10]. Hence, Einstein-bumblebee gravity has been explored extensively on various aspects, including exact solutions [11−19], geodesics and gravitational lensing [20−24], black hole shadows [25−29], quasinormal modes and stability [30−37], and energy extraction [38].In this study, we construct the mass ladder operators for the static BTZ-like black hole obtained in Ref. [9] in Einstein-bumblebee gravity, and we probe the QNM frequencies of the mapped modes using mass ladder operators for the scalar perturbation under Dirichlet and Neumann boundary conditions. We observe that the mass ladder operators, which depend on the Lorentz symmetry breaking parameter s in Einstein-bumblebee gravity, can map a solution of the massive Klein-Gordon equation for the BTZ-like black hole into that with different mass squared values and change indices of QNM overtones. Particularly, we find that a threshold value
$ s_c $ exists for all the modes, i.e., the quasinormal frequency has an imaginary part only if the parameter$ s<s_c $ .The remainder of this article is organized as follows. In Sec. II, we first review the static BTZ-like black hole in Einstein-bumblebee gravity; we then calculate the QNMs and frequencies for a massive perturbation of the static BTZ-like black hole under the Dirichlet and Neumann boundary conditions. In Sec. III, we construct the mass ladder operators in the static BTZ-like spacetime, discuss the relationship between the mass ladder operators and QNMs, and analyze the effect of the overtone number n and Lorentz symmetry breaking parameter s on the mapped modes. Finally, in Sec. IV, we summarize our study.
-
Let us begin by briefly reviewing the static BTZ-like spacetime in Einstein-bumblebee gravity obtained in [9]. The action in this theoretical model with a negative cosmological constant
$ \Lambda = -\dfrac{1}{l^2} $ is given by [39−41]$ \begin{aligned}[b] {\cal{S}} =& \int {\rm d}^3x\sqrt{-g}\Big[\frac{R-2\Lambda}{2\kappa}+\frac{\varrho}{2\kappa} B^{\mu}B^{\nu}R_{\mu\nu}-\frac{1}{4}B^{\mu\nu}B_{\mu\nu} \\& -V(B_\mu B^{\mu}\pm b^2)+{\cal{L}}_M\Big]. \end{aligned}$

(1) Here, R describes the Ricci scalar, and κ is a constant that has a relationship with the three-dimensional Newton's constant G, given by
$ \kappa = 8\pi G $ .$ B_{\mu\nu} = \partial_{\mu}B_{\nu}-\partial_{\nu}B_{\mu} $ is the strength of the bumblebee field$ B_\mu $ , and$ \varrho $ is a coupling constant with a dimension of$ M^{-1} $ in this model. In addition, we note that the potential V is minimum at$ B^{\mu}B_{\mu}\pm b^2 = 0 $ , where b is a real positive constant and the signs$ \pm $ determine whether the field$ b_\mu $ is timelike or spacelike. This minimum yields a nonzero vacuum value$ \langle B_{\mu}\rangle = b_\mu $ with$ b_\mu b^\mu = \mp b^2 $ , which results in the breaking of$ U(1) $ symmetry. Note that the nonzero vector background$ b_\mu $ results in the violation of Lorentz symmetry [5, 39−41].From the action (1), we can obtain the static BTZ-like black hole solution in Einstein-bumblebee gravity [9]:
$ {\rm d}s^{2} = -f(r){\rm d}t^{2}+\frac{1+s}{f(r)} {\rm d}r^{2}+r^{2} {\rm d}\varphi^{2}, $

(2) with
$ f(r) = \frac{r^2}{l^2}-M, $

(3) where M denotes the mass of the black hole, and
$ s = \xi b^2 $ represents the spontaneous breaking of Lorentz symmetry owing to the Einstein-bumblebee vector field with the form$ b_{\mu} = (0,b\xi, 0, 0) $ . Considering the determinant of the metric$ g = -(1+s)r^2 $ , we find that the metric becomes degenerate at$ s = -1 $ . In the following analysis, we consider the constraint$ s>-1 $ and compare the results with those for$ s = 0 $ , i.e., without Lorentz symmetry breaking. The Hawking temperature of this BTZ-like black hole is$ T_H = \frac{r_{H}}{8\pi l^2\sqrt{1+s}}, $

(4) with the horizon
$ r_{H} = \sqrt{M}l $ . For convenience, we will scale$ l = 1 $ in the numerical calculation.In the static BTZ-like spacetime, the massive scalar field evolves according to
$ \left(\nabla_{\mu}\nabla^{\mu}-\mu^2\right)\Psi = 0, $

(5) where μ is the mass of the scalar field. Assuming that the massive scalar perturbation Ψ has the form
$ \Psi = {\rm e}^{-{\rm i}\omega t+{\rm i}m\varphi}\psi(r), $

(6) we can obtain the radial equation
$ \begin{aligned}[b] & \frac{{\rm d}^2\psi(r)}{{\rm d}r^2}+\bigg[\frac{1}{r}+\frac{f'(r)}{f(r)}\bigg]\frac{{\rm d}\psi(r)}{{\rm d}r}\\&+(1+s) \bigg[\frac{\omega^2}{f(r)^2} -\frac{m^2}{r^2f(r)}-\frac{\mu^2}{f(r)}\bigg]\psi(r) = 0, \end{aligned}$

(7) which can be solved in terms of the hypergeometric functions [42]. We introduce a variable
$z = {(r^2-r_H^2)}/{r^2}$ ; thus, the radial Eq. (7) can be rewritten as$ \begin{aligned}[b] &z(1-z)\frac{{\rm d}^2\psi(z)}{{\rm d}z^2}+(1-z)\frac{{\rm d}\psi(z)}{{\rm d}z}\\&+\left(\frac{A}{z}-\frac{B}{1-z}-C\right)\psi(z) = 0, \end{aligned}$

(8) where A, B, and C have the forms
$ A = \frac{\omega^2l^4(1+s)}{4r_H^2},\quad B = \frac{\mu^2l^2(1+s)}{4},\quad C = \frac{m^2l^2(1+s)}{4r_H^2}. $

(9) Rewriting the radial function
$ \psi(z) $ as the form$ \psi(z) = z^{{\rm{i}}\tfrac{l^2\sqrt{1+s}}{2r_H}\omega} (1-z)^{\tfrac{1+\sqrt{1+\mu^2l^2(1+s)}}{2}}F(z), $

(10) we find that the function
$ F(z) $ satisfies the standard hypergeometric equation$ z(1-z)\frac{{\rm d}^2F(z)}{{\rm d}z^2}+[c-(1+a+b)z]\frac{{\rm d}F(z)}{{\rm d}z}+ab F(z) = 0, $

(11) with
$ a = \frac{1+\sqrt{1+\mu^2l^2(1+s)}}{2}-{\rm i}\frac{l\sqrt{1+s}}{2r_H}(\omega l-m), $

(12) $ b = \frac{1+\sqrt{1+\mu^2l^2(1+s)}}{2}-{\rm i}\frac{l\sqrt{1+s}}{2r_H}(\omega l+m), $

(13) $ c = 1-{\rm i}\frac{l^2\sqrt{1+s}}{r_H}\omega, $

(14) where the scalar field mass must obey
$ \mu^2\geqslant\mu^2_{\rm BF} $ with the Breitenlohner-Freedman (BF) bound$ \mu_{\rm BF}^2 = -\dfrac{1}{l^2(1+s)} $ . Thus, the general solution of the radial Eq. (8) can be given by a linear combination of hypergeometric functions F, i.e.,$ \begin{aligned}[b] \psi(z) = &z^{{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\omega} (1-z)^{\tfrac{1+\sqrt{1+\mu^2l^2(1+s)}}{2}} \Bigg[A F(a,b,c;z)\\&+B z^{{\rm i}\tfrac{\omega l^2 \sqrt{1+s}}{r_H}}F(a-c+1,b-c+1,2-c;z)\Bigg], \end{aligned}$

(15) where A and B are two constants of integration. By imposing the ingoing-wave condition at the black hole horizon, we obtain
$ B = 0 $ ; thus, the solution (15) has a more simple form:$ \psi(z) = A z^{{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\omega} (1-z)^{\tfrac{1+\sqrt{1+\mu^2l^2(1+s)}}{2}} F(a,b,c;z). $

(16) To probe the asymptotic behavior of
$ \psi(z) $ at infinity as$ r\rightarrow\infty $ (i.e.,$ z\rightarrow 1 $ ), we can expand the hypergeometric functions at$ 1-z $ [42], i.e.,$ \begin{aligned}[b] {l} F(a,b,c;z) =& \dfrac{\Gamma(c)\Gamma(c-a-b)}{\Gamma(c-a)\Gamma(c-b)}F(a, b, a+b-c+1; 1-z)\\& +(1-z)^{c-a-b}\dfrac{\Gamma(c)\Gamma(a+b-c)}{\Gamma(a)\Gamma(b)}\\&F(c-a, c-b, c-a-b+1; 1-z). \end{aligned} $

(17) Thus, the general solution (16) can be expressed as
$ \psi(r) = A_{\rm{I}}\psi^{(D)}(r)+A_{\rm{II}}\psi^{(N)}(r), $

(18) where the constants
$ A_{\rm{I}} $ and$ A_{\rm{II}} $ are$ A_{\rm{I}} = A\dfrac{\Gamma(c)\Gamma(c-a-b)}{\Gamma(c-a)\Gamma(c-b)},\quad\quad\quad A_{\rm{II}} = A\dfrac{\Gamma(c)\Gamma(a+b-c)}{\Gamma(a)\Gamma(b)}. $

(19) The functions
$ \psi^{(D)}(r) $ and$ \psi^{(N)}(r) $ denote the forms of solution (16) that satisfy the Dirichlet and Neumann boundary conditions, respectively, at infinity [4]. The forms of$ \psi^{(D)}(r) $ and$ \psi^{(N)}(r) $ are$ \begin{aligned}[b]\psi^{(D)}(r) =& \left(1-\dfrac{r_H^2}{r^2}\right)^{{\rm i} \tfrac{l^2\sqrt{1+s}}{2r_H}\omega}\left(\dfrac{r_H}{r}\right)^{1+\sqrt{1+\mu^2l^2(1+s)}}\\& \times F\left(a, b, a+b-c+1; \dfrac{r_H^2}{r^2}\right),\end{aligned} $

(20) $ \begin{aligned}[b]\psi^{(N)}(r) =& \left(1-\dfrac{r_H^2}{r^2}\right)^{{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\omega}\left(\dfrac{r_H}{r}\right)^{1-\sqrt{1+\mu^2l^2(1+s)}} \\ & \times F\left(c-a, c-b, c-a-b+1; \dfrac{r_H^2}{r^2}\right).\end{aligned} $

(21) With the Dirichlet boundary condition, we find
$ a = -n $ or$ b = -n $ and then obtain the quasinormal frequencies for the massive scalar perturbation$ \omega^{(D)} = \pm\frac{m}{l}-{\rm i}\frac{2r_H}{l^2\sqrt{1+s}}\bigg[n+\frac{1}{2}+\frac{1}{2}\sqrt{1+\mu^2l^2(1+s)}\bigg]. $

(22) The signs
$ + $ and$ - $ denote the left-moving and right-moving modes, respectively. As s increases, the absolute value of the imaginary part of$ \omega^{(D)} $ decreases for different n values [10], which is also shown in Fig. 1(a). Moreover, we also note that all the imaginary parts of$ \omega^{(D)} $ are negative, which means that the black hole is stable under the scalar perturbation with the Dirichlet boundary condition. Additionally, the corresponding wave-function$ \Psi^{(D)} $ at infinity has the asymptotic behavior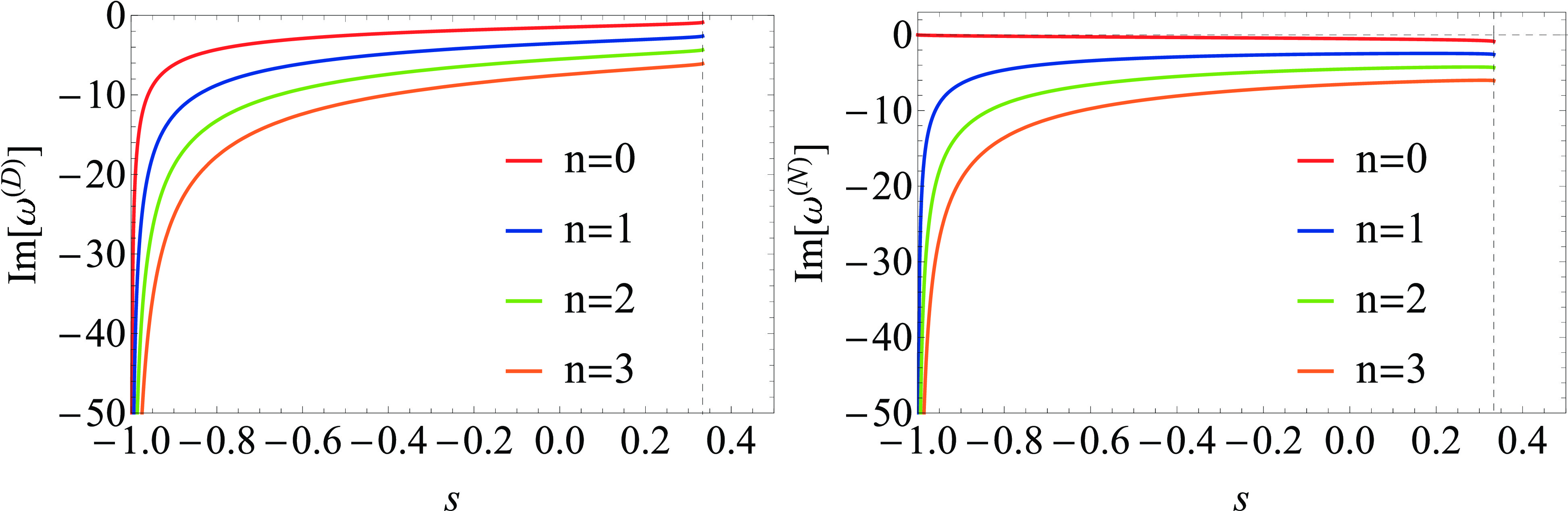
Figure 1. (color online) Relationship between the imaginary part of the QNM frequencies and the Lorentz symmetry breaking parameter s with different overtone numbers n under different boundary conditions, where
$ r_H = 1 $ and$ \mu^2 = -0.75 $ .$ \begin{aligned}[b] \Psi^{(D)}|_{r\rightarrow\infty} =&A_{\rm{I}} \left(\dfrac{r_H}{r}\right)^{1+\sqrt{1+\mu^2l^2(1+s)}} \\& \times \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\omega^{(D)}t+im\varphi}. \end{aligned}$

(23) Similarly, for the Neumann boundary condition, we obtain
$ c-a = -n $ or$ c-b = -n $ ; thus, the quasinormal frequencies for the scalar field are$ \omega^{(N)} = \pm \frac{m}{l}-{\rm i}\frac{2r_H}{l^2\sqrt{1+s}}\bigg[n+\frac{1}{2}-\frac{1}{2}\sqrt{1+\mu^2l^2(1+s)}\bigg]. $

(24) As shown in Fig. 1(b), similar to the case of the Dirichlet boundary condition, the imaginary part of
$ \omega^{(N)} $ increases for different n values as s increases under the Neumann boundary condition, except for the fundamental mode$ n = 0 $ , i.e., the scalar field without nodes, where the imaginary part of$ \omega^{(N)} $ decreases slowly as s increases. Note that the asymptotic behavior of the wave-function$ \Psi^{(N)} $ at infinity becomes$ \begin{aligned}[b] \Psi^{(N)}|_{r\rightarrow\infty} =& A_{\rm{II}} \left(\frac{r_H}{r}\right)^{1-\sqrt{1+\mu^2l^2(1+s)}} \\& \times \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\omega^{(N)}t+{\rm i}m\varphi}.\end{aligned} $

(25) -
For the d-dimensional spacetime
$ (M,g_{\mu\nu}) $ with the symmetry under the conformal transformation$g_{\mu\nu}\rightarrow g'_{\mu\nu} = e^{2Q}g_{\mu\nu}u\nu}$ and$ Q = \frac{1}{d}\nabla_\sigma\zeta^\sigma $ , a conformal Killing vector (CKV)$ \zeta^\mu $ exists that satisfies the conformal Killing equation$ \nabla_{\mu}\zeta_\nu+\nabla_\nu\zeta_\mu = \frac{2}{d}\nabla_\sigma\zeta^\sigma g_{\mu\nu}. $

(26) Because the closed conformal Killing vector (CCKV)
$ \zeta^{\mu} $ satisfies an extra condition$ \nabla_{[\mu}\zeta_{\nu]} = 0, $

(27) it satisfies the reduced Killing equation
$ \nabla_{\mu}\zeta_\nu = \frac{1}{d}\nabla_\sigma\zeta^\sigma g_{\mu\nu}. $

(28) Recent investigations show that a CCKV
$ \zeta^{\mu} $ is an eigenvector of Ricci tensor with a constant eigenvalue χ [1, 2], i.e.,$ R^\mu_{\enspace\nu}\zeta^{\nu} = \chi(d-1)\zeta^{\mu}. $

(29) We can introduce a one-parameter family of operators
$ D_{k}: = {\cal{L}}_{\zeta}-\frac{k}{d}\nabla_{\mu}\zeta^\mu, $

(30) where
$ {\cal{L}}_{\zeta} $ denotes the Lie derivative with respect to$ \zeta^\mu $ , and k is a parameter. The commutation relation between the operator$ D_{k} $ and the d'Alembertian is given by$ \begin{aligned}[b] [\nabla_\mu \nabla^\mu,D_k] =& \chi(2k+d-2)D_k\\&+2Q(\nabla_\mu \nabla^\mu+\chi k(k+d-1)).\end{aligned} $

(31) Acting the commutator on a scalar field ϕ, we can obtain
$ \begin{aligned}[b]&D_{k-2}\left[\nabla_\mu \nabla^\mu+\chi k(k+d-1)\right]\phi\\ =\;& \left[\nabla_\mu \nabla^\mu+\chi (k-1)(k+d-2)\right]D_k\phi. \end{aligned}$

(32) The operator
$ D_k $ maps a scalar field ϕ with mass squared$ \mu^2 = -\chi k(k+d-1) $ to another scalar field$ D_k\phi $ with a new mass squared$ \mu'^2 = -\chi(k-1)(k+d-2) $ ; therefore,$ D_k $ can be considered a mass ladder operator, which shifts parameter k to$ k-1 $ in the mass squared term. Thus, this type of operator is called the mass lowering operator$ D_{k_-} $ . Similarly, we can introduce the mass raising operator$ D_{k_+} $ , which shifts parameter k to$ k+1 $ in the mass squared term.For the BTZ-like spacetime in Einstein-bumblebee gravity (2), we obtain four independent CCKVs
$ \zeta_{0} = {\rm e}^{\tfrac{r_H}{l^2\sqrt{1+s}}t}\left(\frac{1}{\sqrt{r^2-{r_H}^2}}\partial_t-\frac{r\sqrt{r^2-{r_H}^2}}{r_H l^2 \sqrt{1+s}}\partial_r\right), $

(33) $ \zeta_{1} = {\rm e}^{-\tfrac{r_H}{l^2\sqrt{1+s}}t}\left(\frac{1}{\sqrt{r^2-{r_H}^2}}\partial_t+\frac{r\sqrt{r^2-{r_H}^2}}{r_H l^2 \sqrt{1+s}}\partial_r\right), $

(34) $ \zeta_{2} = {\rm e}^{\tfrac{r_H}{l\sqrt{1+s}}\varphi}\left(\frac{r^2-{r_H}^2}{r_H l\sqrt{1+s}}\partial_r+\frac{1}{r}\partial_\varphi\right), $

(35) $ \zeta_{3} = {\rm e}^{-\tfrac{r_H}{l\sqrt{1+s}}\varphi}\left(-\frac{r^2-{r_H}^2}{r_H l\sqrt{1+s}}\partial_r+\frac{1}{r}\partial_\varphi\right), $

(36) and the corresponding four mass ladder operators
$ \begin{aligned}[b] D_{0,k} =\;& {\rm e}^{\tfrac{r_H}{l^2\sqrt{1+s}}t}\Bigg(\frac{1}{\sqrt{r^2-{r_H}^2}}\partial_t-\frac{r\sqrt{r^2-{r_H}^2}}{r_Hl^2 \sqrt{1+s} }\partial_r+k\frac{\sqrt{r^2-{r_H}^2}}{r_H l^2 \sqrt{1+s}}\Bigg), \end{aligned}$

(37) $ \begin{aligned}[b]D_{1,k} =& {\rm e}^{-\tfrac{r_H}{l^2\sqrt{1+s}}t}\Bigg(\frac{1}{\sqrt{r^2-{r_H}^2}}\partial_t\\&+\frac{r\sqrt{r^2-{r_H}^2}}{r_H l^2\sqrt{1+s} }\partial_r-k\frac{\sqrt{r^2-{r_H}^2}}{r_H l^2 \sqrt{1+s}}\Bigg),\end{aligned} $

(38) $ D_{2,k} = {\rm e}^{\tfrac{r_H}{l\sqrt{1+s}}\varphi}\Bigg(\frac{r^2-{r_H}^2}{r_H l\sqrt{1+s}}\partial_r+ \frac{1}{r}\partial_\varphi-k\frac{r}{r_H l\sqrt{1+s}}\Bigg), $

(39) $ D_{3,k} = {\rm e}^{-\tfrac{r_H}{l\sqrt{1+s}}\varphi}\left(-\frac{r^2-{r_H}^2}{r_H l\sqrt{1+s}}\partial_r+\frac{1}{r}\partial_\varphi+k\frac{r}{r_H l\sqrt{1+s}}\right). $

(40) The mass ladder operators depend on s in Einstein-bumblebee gravity. In an AdS
$ _d $ -like spacetime, the BF-bound$ \mu^2_{BF} $ for a scalar field can be expressed as [1, 4]$ \mu^2_{\rm BF} = \frac{(d-1)^2}{4}\chi,\quad \chi<0. $

(41) For the BTZ-like black hole spacetime in Einstein-bumblebee gravity (2), we find that χ is exactly equal to the BF-bound
$ \mu^2_{BF} = -\dfrac{1}{l^2(1+s)} $ , and then the commutation relation (31) with the d'Alembertian becomes$ \left[\nabla_\mu\nabla^\mu, D_{i,k}\right] = -\frac{2k+1}{l^2(1+s)}D_{i,k} +\frac{2}{3}(\nabla_\mu \zeta_i^\mu)\left[\nabla_\mu\nabla^\mu -\frac{k (k+2)}{l^2(1+s)}\right]. $

(42) This means that Eq. (32) can be further simplified as
$ D_{i,k-2}\left[\nabla_\mu\nabla^\mu-\frac{k(k+2)}{l^2(1+s)}\right]\Psi = \left[\nabla_\mu\nabla^\mu -\frac{(k-1)(k+1)}{l^2(1+s)}\right]D_{i,k}\Psi. $

(43) As in [1, 2],
$ D_{i,k} $ maps a solution Ψ to the Klein-Gordon equation with the mass squared$\mu^2 = \dfrac{k (k+2)}{l^2(1+s)}$ into another solution$ D_{i,k}\Psi $ with the mass squared$ \mu^2 = \dfrac{(k-1)(k+1)}{l^2(1+s)} $ , namely, it shifts k to$ k-1 $ in the mass squared term.To require that the characteristic exponents with the mass
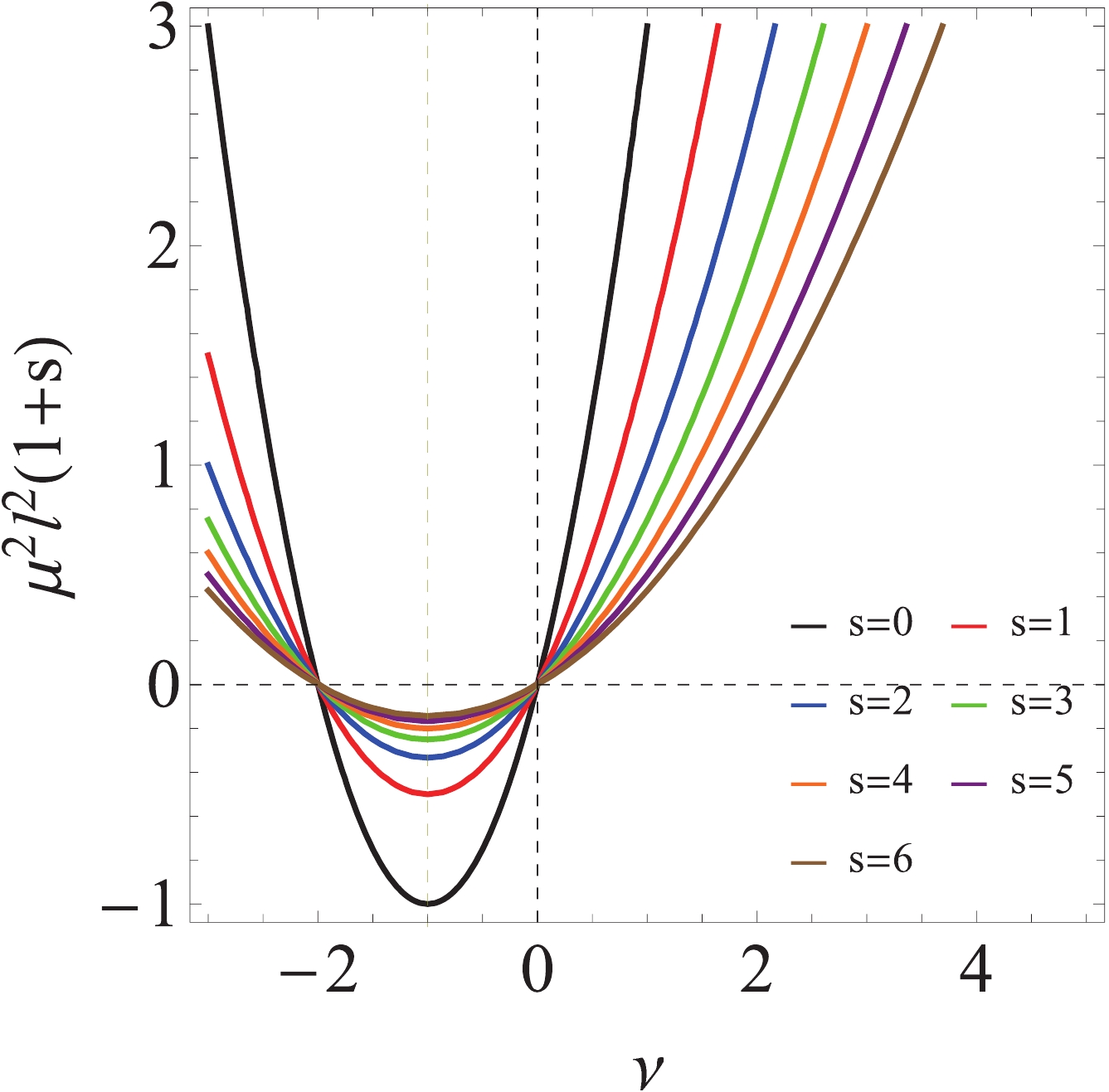
$ \Delta_{\pm} = \dfrac{1}{2}[1\pm\sqrt{1+\mu^{2}l^{2}(1+s)}] $ in (23) and (25) are real, we focus only on the mass squared$ \mu^2\geqslant\mu^2_{\rm BF} $ . As in [4], we can introduce a parameter ν, which obeys$ \mu^2 l^2(1+s) = \nu(\nu+2). $

(44) This means that
$ \nu = -1\pm\sqrt{1+\mu^2l^2(1+s)} $ . The relationship between$ \mu^2l^2(1+s) $ and ν for different values of s is presented in Fig. 2, which shows that the function$ \mu^2l^2(1+s) $ is symmetric about$ \nu = -1 $ . Thus, without loss of generality, we focus only on the right part of the parabola where$ \nu = -1+\sqrt{1+\mu^2l^2(1+s)} $ with$ \nu\in[-1,\infty) $ . For a given parameter ν, two values of k satisfy$k(k+2) = \nu(\nu+2)$ , i.e.,$ k_+ = -2-\nu,\;\; k_- = \nu. $

(45) The corresponding mass ladder operators
$ D_{i,k_+} $ and$ D_{i,k_-} $ shift ν to$ \nu+1 $ and$ \nu-1 $ , respectively.Now, we can discuss the mapped solutions resulting from the mass ladder operators acting on the original solution Ψ. Because the operators
$ D_{2,k\pm} $ and$ D_{3,k\pm} $ are non-global operators, they are not globally smooth in the φ direction. Thus, they change the quantum number m of the scalar field. However, the operators$ D_{0,k\pm} $ and$ D_{1,k\pm} $ can directly affect the frequencies of the scalar perturbation. Therefore, as in [4], we focus only on the effects of$ D_{0,k\pm} $ and$ D_{1,k\pm} $ on the general solution of the scalar field under different boundary conditions.The general solution (16) of the scalar field can be rewritten as
$ \Psi = A\left(1-\frac{r^2_H}{r^2}\right)^{-{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\omega} \left(\frac{r_H}{r}\right)^{2+\nu} F\left(a,b,c;1-\frac{r^2_H}{r^2}\right){\rm e}^{-{\rm i}\omega t+{\rm i}m\varphi}. $

(46) The asymptotic behavior of the general solution near the horizon is
$ \begin{aligned}[b]\Psi|_{r\simeq r_H} =& 2^{-{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\omega}A\left(\frac{r-r_H}{r}\right)^{-{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\omega}\\ & \times \left[1+{\cal{O}}(r-r_H)\right]{\rm e}^{-{\rm i}\omega t+{\rm i}m\varphi}. \end{aligned}$

(47) Under the Dirichlet or Neumann boundary conditions at infinity, the above general solution has the asymptotic behavior
$ \Psi|_{r\simeq \infty} = A_{\rm{I}}\left(\frac{r_H}{r}\right)^{2+\nu} \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\omega t+{\rm i}m\varphi} \; \; \; \; { ({\rm{DBC}})}, $

(48) $ \Psi|_{r\simeq \infty} = A_{{\rm{II}}}\left(\frac{r_H}{r}\right)^{-\nu}\left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\omega t+{\rm i}m\varphi}\; \; \; \; {\rm{(NBC)}}. $

(49) Acting the mass ladder operators
$ D_{0,k\pm} $ and$ D_{1,k\pm} $ on the exact solution (46), we can obtain the asymptotic behaviors of the mapped solutions near the horizon:$ \begin{aligned}[b] D_{0,k\pm}\Psi|_{r\simeq r_H} =&C_{0,k\pm}\left(\frac{r-r_H}{r}\right)^{-{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\left(\omega+{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)}\\ &\left[1+{\cal{O}}(r-r_H)\right]{\rm e}^{-{\rm i}\left(\omega+{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)t+{\rm i}m\varphi}, \end{aligned}$

(50) $ \begin{aligned}[b] D_{1,k\pm}\Psi|_{r\simeq r_H} =& C_{1,k\pm}\left(\frac{r-r_H}{r}\right)^{-{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\left(\omega-{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)}\\&\left[1+{\cal{O}}(r-r_H)\right]{\rm e}^{-{\rm i}\left(\omega-{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)t+{\rm i}m\varphi},\end{aligned} $

(51) where
$ C_{0,k\pm} $ and$ C_{1,k\pm} $ are two constants:$ \begin{aligned} C_{0,k\pm} = &\dfrac{{\rm i}A}{r_Hl^4(1+s)\left(\omega +{\rm i}\dfrac{r_H}{l^2\sqrt{1+s}}\right)}2^{-1-{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\left(\omega+{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)} \\ &\times\left[r_H^2(k_{\pm}(2+k_{\pm})-\nu(2+\nu))+l^4(1+s)\left(\omega+\tfrac{m}{l}-{\rm i}k_{\pm}\frac{r_H}{l^2\sqrt{1+s}}\right)\left(\omega-\frac{m}{l}- {\rm i}k_{\pm}\frac{r_H}{l^2\sqrt{1+s}}\right)\right], \end{aligned} $ 
(52) $ C_{1,k\pm} = -2^{1-{\rm i}\tfrac{l^2\sqrt{1+s}}{2r_H}\left(\omega-{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)}\frac{{\rm i}\omega A}{r_H}. $

(53) Under the Dirichlet boundary condition, the asymptotic behaviors of the mapped solutions acted by the mass ladder operators
$ D_{0,k\pm} $ and$ D_{1,k\pm} $ at infinity can be expressed as$ \begin{aligned}[b]D_{0,k+}\Psi^{(D)}|_{r\simeq\infty} =& C_{0,k+}^{(D)}\left(\frac{r_H}{r}\right)^{3+\nu} \\ & \times \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\left(\omega^{(D)}+{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}} \right)t+{\rm i}m\varphi}, \end{aligned}$

(54) $ \begin{aligned}[b] D_{1,k+}\Psi^{(D)}|_{r\simeq\infty} =& C_{1,k+}^{(D)}\left(\frac{r_H}{r}\right)^{3+\nu}\\ & \times \left[1+{\cal{O}} (1/r^2)\right]{\rm e}^{-{\rm i}\left(\omega^{(D)}-{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)t+{\rm i}m\varphi},\end{aligned} $

(55) $ \begin{aligned}[b]D_{0,k-}\Psi^{(D)}|_{r\simeq\infty} =& C_{0,k-}^{(D)}\left(\frac{r_H}{r}\right)^{1+\nu}\\&\times \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\left(\omega^{(D)}+{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)t+{\rm i}m\varphi}, \end{aligned}$

(56) $ \begin{aligned}[b] D_{1,k-}\Psi^{(D)}|_{r\simeq\infty} =& C_{1,k-}^{(D)}\left(\frac{r_H}{r}\right)^{1+\nu}\\&\times \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\left(\omega^{(D)}-{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)t+{\rm i}m\varphi}, \end{aligned}$

(57) where
$ \begin{aligned}[b] C^{(D)}_{0,k+} =& -\frac{A_{\rm{I}}}{(2+\nu)}\frac{l^2\sqrt{1+s}}{2r_H^2}\left[\omega^{(D)}-\frac{m}{l}+{\rm i}\frac{r_H}{l^2\sqrt{1+s}}(2+\nu)\right]\\&\times \left[\omega^{(D)}+\frac{m}{l}+{\rm i}\frac{r_H}{l^2\sqrt{1+s}}(2+\nu)\right], \end{aligned}$

(58) $ \begin{aligned}[b]C^{(D)}_{1,k+} =& \frac{A_{\rm{I}}}{(2+\nu)}\frac{l^2\sqrt{1+s}}{2r_H^2}\left[\omega^{(D)}-\frac{m}{l}-{\rm i}\frac{r_H}{l^2\sqrt{1+s}}(2+\nu)\right]\\&\times \left[\omega^{(D)}+\frac{m}{l}-{\rm i}\frac{r_H}{l^2\sqrt{1+s}}(2+\nu)\right], \end{aligned}$

(59) $ C^{(D)}_{0,k-} = \frac{2(1+\nu)}{l^2\sqrt{1+s}}A_{\rm{I}},\quad\quad C^{(D)}_{1,k-} = -\frac{2(1+\nu)}{l^2\sqrt{1+s}}A_{\rm{I}}. $

(60) Combining with Eqs. (50), (51), and (54)−(57), we find that
$ D_{0,k\pm} $ and$ D_{1,k\pm} $ shift ω to$\omega+-\dfrac{r_H}{l^2\sqrt{1+s}}$ and$\omega--\dfrac{r_H}{l^2\sqrt{1+s}}$ , respectively, while keeping m unchanged. Thus, the quasinormal frequencies of the mapped solutions of the scalar field by the mass ladder operators$ D_{0,k\pm} $ and$ D_{1,k\pm} $ are$ \omega^{(D)}_{0,1} = \omega^{(D)}\pm -\frac{r_H}{l^2\sqrt{1+s}}, $

(61) with
$ \omega^{(D)} = \pm\frac{m}{l}-{\rm i}\frac{r_H}{l^2\sqrt{1+s}}(2n+\nu+2). $

(62) In particular, when the mass ladder operator
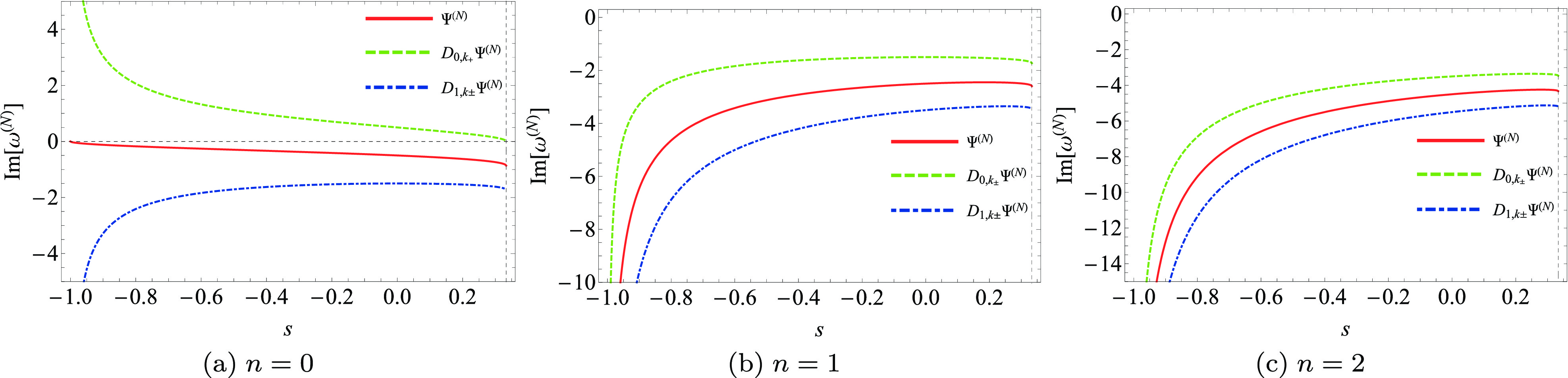
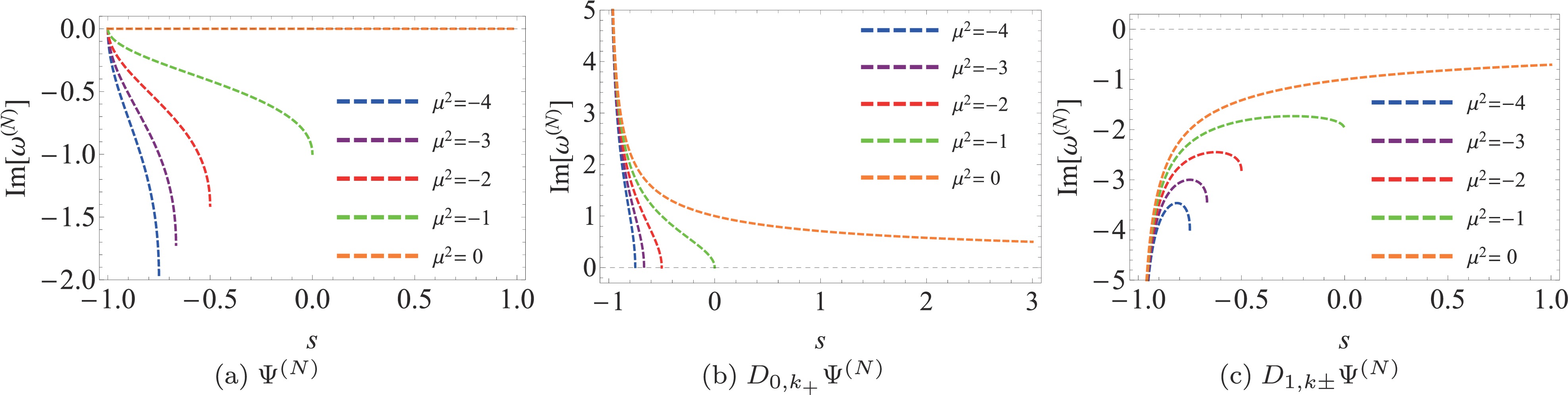
$ D_{0,k+} $ acts on the fundamental mode, the mapped mode vanishes rather than becomes a “negative overtone mode” owing to the restriction imposed by (58) as in [4]. Figure 3 shows the change of quasinormal frequencies of the mapped modes$ D_{0,k\pm}\Psi^{(D)} $ and$ D_{1,k\pm}\Psi^{(D)} $ with the parameter s, which is similar to that of the original modes$ \Psi^{(D)} $ . Notice that, for all the modes with$ \mu^2 = -0.75 $ and$ l = 1 $ , a threshold value$ s_{c} = \dfrac{1}{3} $ exists, corresponding to a vertical dashed line in each panel, which means that all the modes stop increasing their imaginary parts when they reach this vertical line. The threshold value$ s_{c} = -\left(1+\dfrac{1}{\mu^2 l^2}\right) $ depends only on the mass of the scalar field and increases with the mass squared term$ \mu^2 $ , as shown in Fig. 4 for$ \mu^2\leqslant0 $ .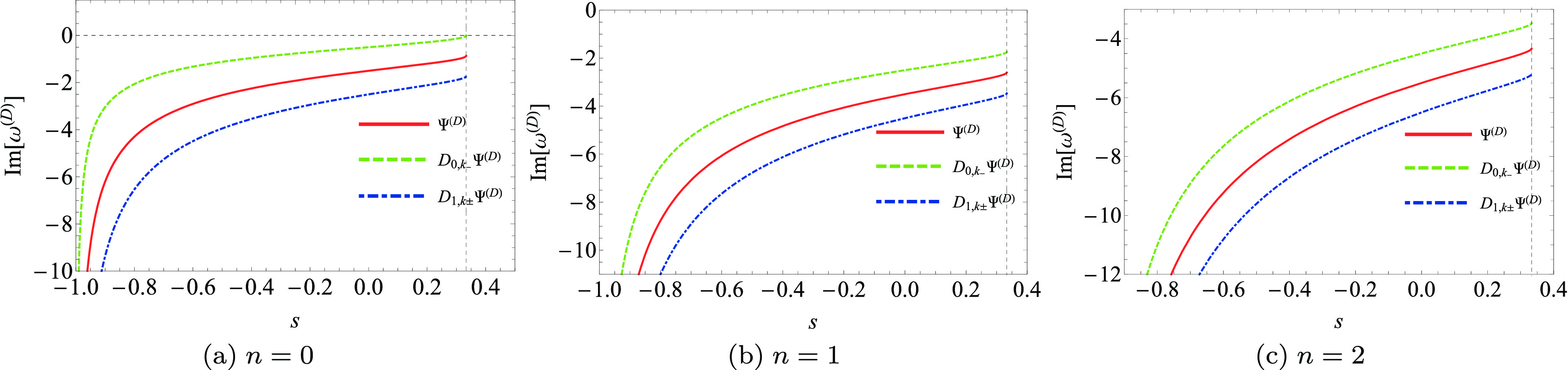
Figure 3. (color online) Relationship between the imaginary part
$ {\rm{Im}} $ $ [\omega^{(D)}] $ and s for$ \Psi^{(D)} $ ,$ D_{0,k\pm}\Psi^{(D)} $ , and$ D_{1,k\pm}\Psi^{(D)} $ for different values of n, where$ r_H = 1 $ and$ \mu^2 = -0.75 $ .
Figure 4. (color online) Relationship between
$ {\rm{Im}} $ $ [\omega^{(D)}] $ and s for$ \Psi^{(D)} $ ,$ D_{0,k_-}\Psi^{(D)} $ , and$ D_{1,k\pm}\Psi^{(D)} $ with different masses$ \mu^2 $ , where$ n = 0 $ and$ r_H = 1 $ .Under the Neumann boundary condition, we can obtain the asymptotic behaviors of the mapped solutions by mass ladder operators
$ D_{0,k\pm} $ and$ D_{0,k\pm} $ at infinity:$ \begin{aligned}[b] D_{0,k+}\Psi^{(N)}|_{r\simeq\infty} =& C_{0,k+}^{(N)}\left(\frac{r_H}{r}\right)^{-1-\nu}\\&\times \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\left(\omega^{(N)}+{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)t+{\rm i}m\varphi}, \end{aligned}$

(63) $ \begin{aligned}[b] D_{1,k+}\Psi^{(N)}|_{r\simeq\infty} =& C_{1,k+}^{(N)}\left(\frac{r_H}{r}\right)^{-1-\nu}\\&\times \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\left(\omega^{(N)}-{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)t+{\rm i}m\varphi}, \end{aligned}$

(64) $ \begin{aligned}[b] D_{0,k-}\Psi^{(N)}|_{r\simeq\infty} =& C_{0,k-}^{(N)}\left(\frac{r_H}{r}\right)^{1-\nu}\\&\times \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\left(\omega^{(N)}+{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)t+{\rm i}m\varphi},\end{aligned} $

(65) $\begin{aligned}[b] D_{1,k-}\Psi^{(N)}|_{r\simeq\infty} =& C_{1,k-}^{(N)}\left(\frac{r_H}{r}\right)^{1-\nu}\\&\times \left[1+{\cal{O}}(1/r^2)\right]{\rm e}^{-{\rm i}\left(\omega^{(N)}-{\rm i}\tfrac{r_H}{l^2\sqrt{1+s}}\right)t+{\rm i}m\varphi},\end{aligned} $

(66) where
$ C^{(N)}_{0,k+} = -\frac{2(1+\nu)}{l^2\sqrt{1+s}}A_{\rm{II}},\quad\quad C^{(N)}_{1,k+} = \frac{2(1+\nu)}{l^2\sqrt{1+s}}A_{\rm{II}}, $

(67) $ \begin{aligned}[b]C^{(N)}_{0,k-} =& \frac{A_{\rm{II}}}{\nu}\frac{l^2\sqrt{1+s}}{2r_H^2}\left[\omega^{(N)} -\frac{m}{l}-{\rm i}\frac{r_H}{l^2\sqrt{1+s}}\nu\right]\\&\times \left[\omega^{(N)} +\frac{m}{l}-{\rm i}\frac{r_H}{l^2\sqrt{1+s}}\nu\right],\end{aligned} $

(68) $ \begin{aligned}[b]C^{(N)}_{1,k-} =& -\frac{A_{\rm{II}}}{\nu}\frac{l^2\sqrt{1+s}}{2r_H^2}\left[\omega^{(N)} -\frac{m}{l}+{\rm i}\frac{r_H}{l^2\sqrt{1+s}}\nu\right]\\ &\times \left[\omega^{(N)} +\frac{m}{l}+{\rm i}\frac{r_H}{l^2\sqrt{1+s}}\nu\right]. \end{aligned}$

(69) Combining with Eqs. (50), (51), (63)−(66), we find that
$ D_{0,k\pm} $ and$ D_{1,k\pm} $ shift ω to$\omega+{\rm i}\dfrac{r_H}{l^2\sqrt{1+s}}$ and$\omega- {\rm i}\dfrac{r_H}{l^2\sqrt{1+s}}$ , respectiveley, while keeping m unchanged. Thus, the quasinormal frequencies of the mapped solutions of the scalar field by the mass ladder operators$ D_{0,k\pm} $ and$ D_{1,k\pm} $ are$ \omega^{(N)}_{0,1} = \omega^{(N)}\pm {\rm i}\frac{r_H}{l^2\sqrt{1+s}}, $

(70) with
$ \omega^{(N)} = \pm\frac{m}{l}-{\rm i}\frac{r_H}{l^2\sqrt{1+s}}(2n-\nu). $

(71) Similarly, under the Neumann boundary condition, no ''negative overtones'' are generated from the fundamental modes for
$ D_{0,k_-} $ as in the case with the Dirichlet boundary condition. For the fundamental mode with$ \mu^2<0 $ , the mass ladder operators do not change the BF-bound$\mu^2_{\rm BF}$ . Furthermore, as in the Dirichlet boundary condition, we find that the same threshold value$s_c = {1}/{3}$ also exists for the mapped and original modes. The threshold value$ s_c $ for all the mapped modes with the same mass squared term is a constant. Thus, the mass ladder operators maintain the threshold value$ s_c $ for the mapped modes.Moreover, we observe that, for the
$n=0 $ case in Fig. 5, the modes$ D_{0,k_+}\Psi^{(N)} $ and$\Psi^{(N)} $ decrease with the increase in s, which differ with the other modes. Therefore, we focus on the$n=0 $ case as shown in Fig. 6, which indicates that the threshold value$s_c $ increases with the increase in$\mu^2 $ . Note that the imaginary parts of the quasinormal frequencies of the mapped modes caused by$ D_{0,k_+}\Psi^{(N)} $ are all positive, which indicates that all the fundamental modes of$ D_{0,k_+}\Psi^{(N)} $ are unstable. -
In this study, we investigated mass ladder operators constructed from the conformal symmetry of the static BTZ-like black hole in Einstein-bumblebee gravity. Additionally, we probed the QNM frequencies of the mapped modes using mass ladder operators for the scalar perturbation under the Dirichlet and Neumann boundary conditions. We found that the mass ladder operators, which can change not only the mass squared but also the QNM frequencies, depend on the Lorentz symmetry breaking parameter s in Einstein-bumblebee gravity. We observed that the imaginary parts of the frequencies shifting by mass ladder operators increase with an increase in s. The changes of quasinormal frequencies of the mapped modes with s are similar to those of the original modes under two boundary conditions. However, under the Neumann boundary condition, the imaginary parts of the quasinormal frequencies of the mapped modes caused by the mass ladder operator
$ D_{0,k_+} $ are all positive, which implies that all the corresponding fundamental modes are unstable. Moreover, mass ladder operators do not change the BF-bound$ \mu^2_{BF} $ as in the usual BTZ black hole, and the threshold value of the Lorentz symmetry breaking parameter$ s_{c} $ , where the modes stop increasing their imaginary parts, increases with an increase in mass squared$ \mu^2 $ . These results can aid us in further understanding the conformal symmetry and Lorentz symmetry breaking of the static BTZ-like black hole in Einstein-bumblebee gravity.
Mass ladder operators and quasinormal modes of the static BTZ-like black hole in Einstein-bumblebee gravity
- Received Date: 2024-07-26
- Available Online: 2025-01-15
Abstract: We investigate mass ladder operators for the static BTZ-like black hole in Einstein-bumblebee gravity and probe the quasinormal frequencies of the mapped modes using mass ladder operators for a scalar perturbation under Dirichlet and Neumann boundary conditions. We find that the mass ladder operators depend on the Lorentz symmetry breaking parameter, and the imaginary parts of the frequencies shifted by the mass ladder operators increase with the increase in the Lorentz symmetry breaking parameter under the two boundary conditions. Note that, under the Neumann boundary condition, the mapped modes caused by the mass ladder operator





 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF














 DownLoad:
DownLoad: