-
The heavy ion collision (HIC) experiments at the relativistic heavy ion collider (RHIC) and large hadron collider (LHC) are hypothesized to create approximately the most perfect fluid, namely quark gluon plasma (QGP) [1−4]. This provides a novel approach for studying the physics of quantum chromodynamics (QCD) at a strongly coupled regime. Given that the properties of a strongly coupled system cannot be reliably calculated directly by perturbative techniques, one has to resort to some nonperturbative approaches to address the associated challenges.
AdS/CFT correspondence [5−7] is a promising approach to deal with these problems in QCD in a strongly coupled scenario which cannot be handled properly by perturbative methods [8, 9]. Concerning the gravity sector, it has been suggested that an external quark on the gauge theory side is related to a string which has a single endpoint at the boundary and extends down to the horizon of an AdS black hole [10, 11]. Moreover, the diffusion of heavy quarks in a strongly coupled plasma can be understood as the fluctuation correlations of the trailing string. The study of the stochastic nature of a heavy quark in a holography was proposed in [12, 13]. Subsequently, the stochastic motion was formulated as a Langevin process [14, 15]. Given that the heavy quarks in HIC experiments are relativistic in many cases, the relativistic Langevin equation was studied in [16] as well as in non-conformal frameworks in [17, 18], aiming at the multiple scales of QCD.
Methods in AdS/CFT relate a 4-dimensional
$ \mathcal{N} = 4 $ super Yang-Mills (SYM) theory to a type-IIB string theory on the$ AdS_5\times S^5 $ , and string theory contains higher derivative corrections to classical gravity from stringy$ 1/\lambda $ or quantum effects$ 1/N_c $ corrections. It is natural to conduct computations for finite 't Hooft coupling of gauge theory corresponding to studying the effect of higher derivative corrections on computations in classical Einstein gravity. The leading order correction in$ 1/\lambda $ arises from stringy corrections to the low energy effective action of type-IIB supergravity$ \alpha^{\prime 3}\mathcal{R}^4 $ [19], and such corrections to the ratio of shear viscosity to entropy density of a gauge field,$ \eta/s $ , were calculated in [20, 21]. It was found in [22] that the universal low bound on$ \eta/s $ and causality can be violated given general$ \mathcal{R}^2 $ corrections to the gravitational action in GB gravity [22−28]. For instance, in [25], the authors studied the ratio$ \eta/s $ in a 5-dimensional setting, and in [28], the authors studied this ratio in the solution of RN-AdS black branes, finding that the$ \eta/s $ bound is violated and the Maxwell charge slightly reduces the deviation. Likewise, in [25], the authors studied the ratio$ \eta/s $ in a 5-dimensional setting, and in [28], the authors discovered that the$ \eta/s $ bound is violated and the presence of Maxwell charge slightly reduces the deviation in the RN-AdS black brane solution. Motivated by these vast string landscapes, the effect of high derivative curvature$ \mathcal{R}^4 $ or$ \mathcal{R}^2 $ corrections on different aspects of the properties of QGP was studied in [29−35]. Besides, in [36−39], the authors studied curvature-cubic$ \mathcal{R}^3 $ corrections to$ \mathrm{AdS_5} $ .One of the significant applications of the AdS/CFT correspondence is the investigation of jet quenching phenomena involving high transverse momentum partons produced in HICs. It was found in [40] that introducing
$ \mathcal{R}^2 $ corrections to$ \mathrm{AdS_5} $ yields a substantial increase in the nuclear modification factor$ R_{AA} $ . The initial$ 1/\lambda $ correction to the jet quenching parameter was found in [41], followed by the jet quenching parameter including$ \mathcal{R}^2 $ corrections [42, 43]. The trailing string, which models the drag force on a moving heavy quark, was examined within the framework of higher derivative gravity to investigate the$ \mathcal{R}^2 $ and$ \mathcal{R}^4 $ corrections to drag force in [44, 45]. In this study, we focused on investigating$ \mathcal{R}^2 $ corrections to Langevin diffusion coefficients (LGV-coefficients) that are related to the fluctuations of the trailing string.The organization of this paper is as follows. In Section II, we review the main procedures to deduce LGV-coefficients within the membrane paradigm. Additionally, we discuss numerical results of
$ \mathcal{R}^2 $ corrections to LGV-coefficients. In Section III, we study$ \mathcal{R}^2 $ corrections with GB gravity to LGV-coefficients, as in Section II. Section IV is devoted to discussion and conclusions. -
The curvature squared corrections to the solution of the
$ AdS_5 $ -Schwarzschild black brane can be described by the general action [23, 24]$ \begin{aligned}[b] S = \;&\frac{1}{16\pi G_5}\int {\rm d}^5\times \sqrt{-g}\times \left[\mathcal{R}-\Lambda+L^2\left(c_1 \mathcal{R}^2+c_2\mathcal{R}_{\mu\nu}\mathcal{R}^{\mu\nu}\right.\right.\\ & \left.\left.+c_3 \mathcal{R}_{\mu\nu\rho\sigma}\mathcal{R}^{\mu\nu\rho\sigma}\right)\right], \end{aligned} $

(1) where
$ G_5=\pi L^3/2N_c^2 $ is a 5-dimensional Newton constant,$ \mathcal{R} $ is the Ricci scalar, and$ \mathcal{R}_{\mu\nu} $ and$ \mathcal{R}_{\mu\nu\rho\sigma} $ are the Ricci and Riemann tensors, respectively. The negative cosmological constant$ \Lambda=-\dfrac{12}{L^2} $ creates an$ AdS $ space with radius L. The parameters$ c_i $ are expected to be of$ o(\alpha') $ , which means that$ c_i=0 $ in the limit of large 't Hooft coupling ($ \lambda\rightarrow \infty $ ). The shear viscosity to entropy ratio was found in [22, 46] to be$ \dfrac{\eta}{s}=\dfrac{1}{4\pi}(1-8c_3)+\mathcal{O}(c_i^2) $ , and the viscosity bound is violated when$ c_3 > 0 $ .The black brane solution of the
$ AdS_5 $ space for Eq. (1) is given by [22]$ {\rm d} s^2=-\left(\frac{r^2}{L^2}\right)f(r){\rm d}t^2+ \left(\frac{r^2}{L^2}\right){\rm d}\vec{x}^{\,2}+\frac{L^2}{r^2f(r)}{\rm d}r^2, $

(2) where
$ f(r)=1-\frac{r_0^4}{r^4}+a+b \frac{r_0^8}{r^8}, $

(3) $ a=\frac{2}{3} \left( 10c_1 + 2c_2 +c_3 \right),~~~ b=2c_3. $

(4) The boundary of the asymptotically AdS geometry is located at
$ r\rightarrow \infty $ , where r denotes the 5-dimensional radial coordinate, and$ (t, \vec{x}) $ labels the left 4-dimensional spacetime of the gauge theory on the boundary. One can solve$ f(r_h)=0 $ to find the location of the horizon$ r = r_h $ , where$ r_h $ depends on a, b, and$ r_0 $ . The heat bath temperature is given by$ T_{R^2}=\frac{r_0}{\pi L^2} \left( 1+ \frac{1}{4} a - \frac{5}{4} b\right) , $

(5) where
$ r_0 $ depends on both a and b for a fixed temperature$ T_{R^2} $ .According to [17, 47, 48], we computed the LGV-coefficients of a heavy quark in a squared-curvature correction background. It is more convenient to conduct the calculations in a more general form:
$ {\rm d}s^2=g_{tt}{\rm d}t^2+g_{ii}{\rm d}x_i^2+g_{rr}{\rm d}r^2. $

(6) According to Eq. (2), we have
$ g_{tt}=-\left(\frac{r^2}{L^2}\right)f(r), \quad g_{ii}=\frac{r^2}{L^2}, \quad g_{rr}=\frac{L^2}{r^2f(r)}. $

(7) Holographically, the moving heavy quark of infinite mass on the boundary CFT corresponds to the endpoint of the trailing string. The string dynamics are captured by the Nambu-Goto action:
$ S_{NG}=-\frac{1}{2\pi\alpha'}\int \mathrm{d}\tau \mathrm{d}\sigma \sqrt{-\mathrm{det} \gamma_{\alpha\beta}}, \qquad \gamma_{\alpha\beta}=g_{\mu\nu}\partial_\alpha X^{\mu} \partial_\beta X^{\nu}. $

(8) where
$ \gamma_{\alpha\beta} $ is the induced metric, and$ g_{\mu\nu} $ and$ X^{\mu} $ are the branes metric and target space coordinates.Given a moving heavy quark with a constant velocity v on the boundary along the chosen direction
$ x_{p}(x_p= x,y,z) $ , one can choose to compute in static gauge for the string world-sheet with the usual parametrization,$ t=\tau, \quad r=\sigma,\quad x=vt+\xi(r), $

(9) where ξ is the profile of the string in the bulk. The world-sheet metric is deduced to be
$ \gamma_{\alpha\beta}= \left( \begin{matrix} g_{tt}+v^2g_{pp} & g_{pp}v\xi' \\ g_{pp}v\xi' & g_{rr}+g_{pp}\xi^{'2} \end{matrix} \right), $

(10) and the corresponding action is
$ S_{NG}=-\frac{1}{2\pi\alpha'}\int \mathrm{d}t \mathrm{d}r \sqrt{-(g_{tt}g_{rr}+g_{tt}g_{pp}\xi'^2+g_{pp}g_{rr}v^2)}. $

(11) Note that
$ g_{pp} $ is the corresponding metric component in the$ x_{p} $ direction. It is evident that the radial conjugate momentum$ \pi_{\xi} $ is conserved for the simple motion:$ \pi_{\xi}=\frac{\delta S}{\delta \xi}=-\frac{1}{2\pi\alpha'}\frac{g_{tt}g_{pp} \xi'}{2\sqrt{-(g_{tt}g_{rr}+g_{tt}g_{pp}\xi'^2+g_{pp}g_{rr}v^2)}}. $

(12) It is easy to find
$ \xi' $ from Eq. (12) as$ \xi'=\sqrt{\frac{-g_{tt}g_{rr}-g_{pp}g_{rr}v^2}{g_{tt}g_{xx}(1+\frac{g_{tt}g_{xx}}{C^2})}}, $

(13) where
$ C\equiv 2\pi\alpha'\pi_{\xi} $ . The world-sheet of the string has a horizon that turns out to be the same with critical point$ r_c $ at which both numerator and denominator change their sign. By inserting Eq. (7) into$ \gamma_{\alpha\alpha}(r_c) = 0 $ , one can identify the critical point$ r_c $ as$ r_c=\sqrt[4]{\frac{r_0^4 \sqrt{1-4 b \left(a-v^2+1\right)}+r_0^4}{2 \left(a-v^2+1\right)}}. $

(14) One can also find the effective temperature
$ T_{ws} $ of the world-sheet horizon by diagonalizing the world-sheet metric expressed by Eq. (10). One can change coordinates to diagonalize the induced metric via the following reparametrization:$ {\rm d}\tau\rightarrow {\rm d}\tau-\frac{\gamma_{\alpha\beta}}{\gamma_{\alpha\alpha}}{\rm d}\sigma. $

(15) The diagonal induced world-sheet metric
$ h_{\alpha\beta} $ is given by$ h_{\alpha\beta}= \left( \begin{matrix} g_{tt}+g_{pp}v^2 & \\ & \dfrac{g_{tt}g_{pp}g_{rr}}{g_{tt}g_{pp}+ \left(2\pi\alpha'\pi_{\xi} \right)^2} \end{matrix} \right). $

(16) Following the usual procedure, the effective world sheet temperature reads
$ \begin{split} T^{2}_{ws}\;&=\frac{1}{16\pi^2}\left(h_{\alpha\alpha}'\,\left(h^{\beta\beta}\right)'\right)\bigg|_{r_c}\\ &=\frac{1}{16\pi^2}\left[(g_{tt}+v^2g_{pp})'\left(\frac{g_{tt}g_{pp}+v^2(g_{pp}|_{r_c})^2}{g_{tt}g_{pp}g_{uu}}\right)'\right]^2\bigg|_{r=r_{c}}\\ &=\frac{1}{16\pi^2}\left| {\frac{g^{'2}_{tt}-v^4g^{'2}_{pp}}{g_{tt}g_{pp}}} \right|\bigg|_{r=r_{c}}\\ &=\frac{1}{16\pi^2}\left| {\frac{1}{g_{tt}g_{rr}}(g_{tt}g_{pp})'\left(\frac{g_{tt}}{g_{pp}}\right)'} \right|\bigg|_{r=r_{c}}.\\[-1pt] \end{split} $

(17) By inserting Eq. (14) into Eq. (17), one has the effective world sheet temperature
$ T^{ws}_{R^2} $ :$ \begin{aligned} T^{ws}_{R^2}=\frac{1}{4\pi}\sqrt{\left| {\left(\frac{8 b r_0^8}{r_c^9}-\frac{4 r_0^4}{r_c^5}\right) \left(4 r_c^3 \left(a+\frac{b r_0^8}{r_c^8}-\frac{r_0^4}{r_c^4}+1\right)+r_c^4 \left(\frac{4 r_0^4}{r_c^5}-\frac{8 b r_0^8}{r_c^9}\right)\right)} \right|}. \end{aligned} $

(18) In the conformal limit, where
$ a\rightarrow 0,\,b\rightarrow 0 $ , the background solution reduces to AdS-BH, and the world-sheet temperature expressed by Eq. (18) is simply related to the bulk temperature:$ \lim\limits_{a\rightarrow 0,b\rightarrow 0}T^{ws}_{R2}=\frac{T_{\text{SYM}}}{\sqrt{\gamma_v}}, $

(19) where
$ \gamma_v $ is the Lorentz factor$ \gamma_v=1/\sqrt{1-v^2} $ and$ T_{\text{SYM}} $ is the bulk temperature in the conformal limit.Considering the fluctuation in the classical trailing string, one has
$ t=\tau,\quad r=\sigma,\quad x_{p}=vt+\xi(\sigma)+\delta x_p(\tau,\sigma), $

(20) where the fluctuation takes the form
$ \delta x_p(\tau,\sigma) $ along and transverse to the direction of$ x_p $ . A simple expression for the quadratic action in the world sheet embedding fluctuations that capture fluctuations of heavy quark reads$ \begin{split} \;&S_2= -\frac{1}{2\pi\alpha'}\int {\rm d}\tau {\rm d}\sigma \frac{H^{\alpha\beta}}{2} \Biggr(N[r]\partial_{\alpha}\delta x_p\partial_{\beta}\delta x_p\\ & \qquad +\sum_{i\neq p} g_{ii}\partial_{\alpha}\delta x_i\partial_{\beta}\delta x_i \Biggr),\\ & N(r)\equiv\frac{g_{tt}g_{pp}+C^2}{g_{tt}+g_{pp}v^2},\\ & H^{\alpha\beta}=\sqrt{-{\rm det} (h)}h^{\alpha\beta}, \end{split} $

(21) where
$ h^{\alpha\beta} $ is the inverse of the diagonalized induced world-sheet metric. Please refer to [12, 17, 47, 49] for a detailed proof. For an arbitrary massless fluctuation ϕ with an action, we have$ S_2=-1/2\int {\rm d}x{\rm d}r\sqrt{-g}Q(r)g^{\alpha\beta}\partial_{\alpha}\partial_{\beta}\phi. $

(22) Taking advantage of the membrane paradigm [50], one can directly obtain the transport coefficient associated with the retarded Green’s function form given by Eq. (22) without solving the motion equation as
$ \begin{split} \chi_{R}&=-\lim\limits_{k_{\mu}\rightarrow0}\frac{\Im G_{R}(\omega,\vec{k})}{\omega}=Q(r_h), \end{split} $

(23) where Q is the only effective coupling of the fluctuation and the metric dependence drops out in the 2-dimensional world sheet black hole horizon.
For sufficiently large times, the temporal correlation functions of the random force operator on a Brownian particle are proportional to Dirac delta distributions, with the proportionality factors defining the Langevin diffusion coefficients. The noise term is determined by the symmetrized real-time correlation functions of the random forces over the statistical ensemble. Then, the LGV-coefficient can be defined in terms of the symmetric correlator
$ G_{\rm sym} $ [12] as$ \begin{split} \kappa_{d}&=\lim\limits_{\omega\rightarrow0} G^{d}_{\rm sym}(\omega)\\ &=-\coth{\frac{\omega}{2T_{ws}}}\lim\limits_{\omega\rightarrow0} (\Im G^d_{R}(\omega))\\ &=-2T_{ws}\lim\limits_{\omega\rightarrow0} \frac{\Im G^d_{R}(\omega)}{\omega}\\ &=2T_{ws}\chi_{R}^{d}\\ &=2T_{ws}Q^{d}(r_c). \end{split} $

(24) where
$ d=(\perp,\parallel) $ . The second step requires the$ \omega\rightarrow 0 $ limit of$ G^{d}_{\rm sym}(\omega)=\coth{\dfrac{\omega}{2T}}\Im G^{d}_{R}(\omega) $ [13], and the third step requires Eq. (23). Comparing Eqs. (21) and (22), one obtains$ \begin{aligned}[b] & Q^{\perp}=\frac{1}{2\pi\alpha}g_{kk}\bigg|_{r=r_c},\quad \\ & Q^{\parallel}=\frac{1}{2\pi\alpha}\lim\limits_{r\rightarrow r_c}N(r)=\frac{1}{2\pi\alpha}\frac{(g_{tt}g_{pp})'}{g_{pp}\left(\dfrac{g_{tt}}{g_{pp}}\right)'}\bigg|_{r=r_c}. \end{aligned} $

(25) Given that
$ N(r_c)=\dfrac{0}{0} $ , the L’Hopital’s rule must be applied to calculate the limit. One can also insert Eq. (25) into Eq. (24), which yields$ \kappa_{\perp}= \frac{1}{\pi \alpha'}g_{kk}|_{r=r_c}T_{ws},\qquad \kappa_{\parallel}=\frac{1}{\pi\alpha'} \frac{( g_{tt}g_{pp})'}{g_{pp} \left(\dfrac{g_{tt}}{g_{pp}}\right)'}\bigg|_{r=r_c}T_{ws}. $

(26) In our case, one can also insert the metric given by Eq. (7) into Eq. (26), obtaining
$ \kappa_{\perp}=\frac{\sqrt{\lambda}}{\pi}r_c^2T^{ws}_{R2}, $

(27) and
$ \kappa_{\parallel} =\frac{r_c^2 \sqrt{\lambda} \left(b r_0^8-(a+1) r_c^8\right)}{\pi r_0^4 \left(2 b r_0^4-r_c^4\right)} T^{ws}_{R2}, $

(28) where we used the fact that
$ \alpha' = \dfrac{L^2}{\sqrt{\lambda}} = \dfrac{1}{\sqrt{\lambda}} $ .In the conformal limit, one can obtain well-known results [12, 13] by taking limits of both
$ a \to 0 $ and$ b \to 0 $ ,$ \kappa_{\perp}^{\rm SYM}=\sqrt{\lambda} \pi T_{\text{\rm SYM}}^3 \gamma_v^{{1}/{2}},\qquad \kappa_{\parallel}^{\rm SYM}=\sqrt{\lambda} \pi T_{\text{SYM}}^3 \gamma_v^{{5}/{2}}. $

(29) One can check that Eqs. (27) and (28) reduce to these results by taking the limit for
$ \alpha \rightarrow 0 $ and$ \beta \rightarrow 0 $ .The effects from
$ \mathcal{R}^2 $ corrections to the classical trailing string, which models the drag force on a moving heavy quark in SYM plasma, were studied in [45] for two distinct scenarios:$T_{R^2} < T_{\rm SYM}$ and$T_{R^2} > T_{\rm SYM}$ . Following this convention, we found it convenient to explore the curvature squared corrections on the fluctuations of the trailing string that is related to LGV-coefficients. More precisely, we investigated the$ \mathcal{R}^2 $ corrections to$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ by evaluating Eqs. (27) and (28) using two distinct sets of values for the parameters a and b.Figure 1 demonstrates the impact of
$ \mathcal{R}^2 $ corrections on LGV-coefficients for$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ normalized by the SYM result expressed by Eq. (29), with two scenarios at the same heat bath temperature ($T_{R^2}=T_{\rm SYM}$ ). Plots (a) in Fig. 1 show$ \mathcal{R}^2 $ corrections on LGV-coefficients at fixed small values of$ a=-0.0005 $ and$ b=+0.0006 $ corresponding to$T_{R^2} < T_{\rm SYM}$ . It is clear from these plots that the$ \mathcal{R}^2 $ corrections to both$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ increase monotonically with the moving velocity of the heavy quark, and corrections to the LGV-coefficients are larger than those of the SYM case at all velocities. However, note also that this type of$ \mathcal{R}^2 $ corrections can be smaller than$ \mathcal{N}=4 $ SYM results in plots (b) in Fig. 1 for$ a=-0.0005 $ and$ b=-0.0007 $ corresponding to$T_{R^2} > T_{\rm SYM}$ . In this case, a critical velocity ($ v_c $ ) exists such that the corrections increase both$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ if$ v>v_c $ while corrections decrease both$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ if$ v>v_c $ .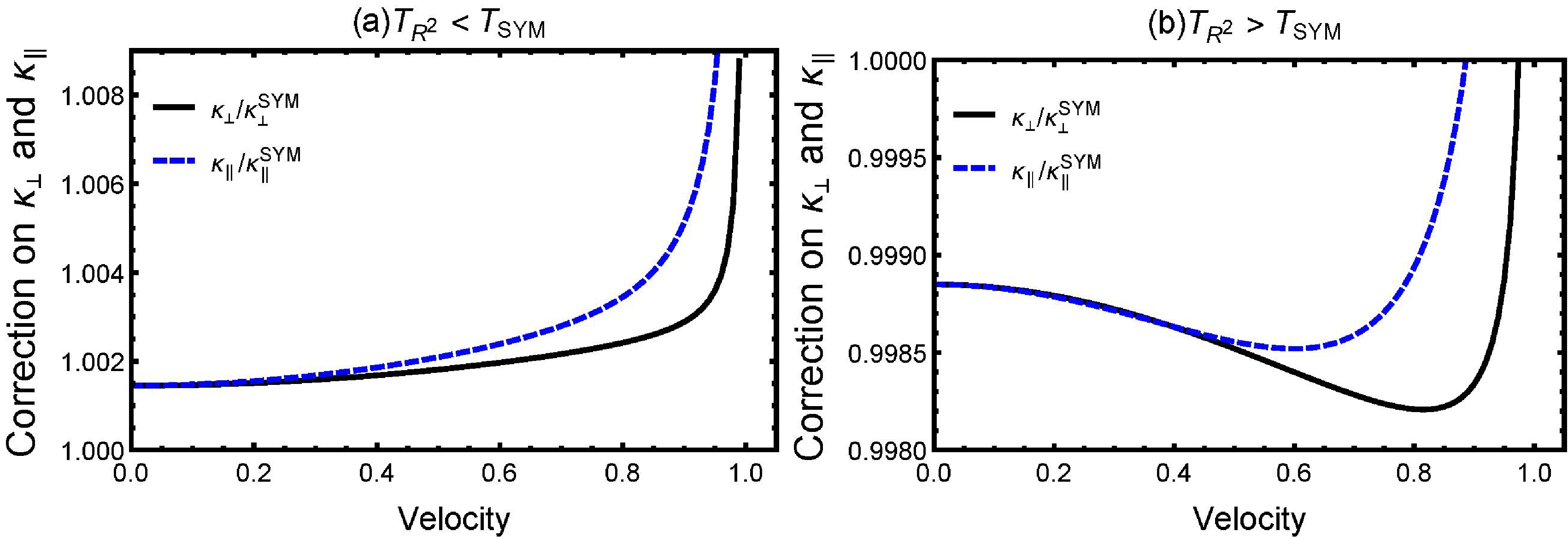
Figure 1. (color online) Corrections to transverse LGV-coefficients
$ \kappa_{\perp} $ and longitudinal LGV-coefficients$ \kappa_{\parallel} $ as a function of the velocity of the heavy quark, normalized by the respective conformal limit.As a result, we conclude that the finite coupling corrections affect both
$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ on a moving quark in the strongly-coupled plasma and depend on the details of curvature squared corrections. The LGV-coefficients can be larger or smaller than those in the infinite-coupling case. However, at a fixed velocity, the corrected$ \kappa_{\parallel} $ is always at least as large as the corrected$ \kappa_{\perp} $ . Moreover, the universal relation$ \kappa_{L}\geq\kappa_{T} $ reported in [17] always holds when$T_{R^2} > T_{\rm SYM}$ and$T_{R^2} < T_{\rm SYM}$ . Our findings are similar to the case of drag force on a moving heavy quark reported in [45]. -
The Gauss-Bonnet (GB) gravity [51] is one of the most interesting theories of gravity with curvature squared correction in five dimensions. The exact solutions and thermodynamic properties of the GB background were discussed in [52−54]. One can also consider the GB gravity as a special case of the general action described by Eq. (1) where
$ c_2=-4c_1 $ and$c_1=c_3=\lambda_{\rm GB}/2$ . This yields an action defined as$ \begin{split} S=\;&\frac{1}{16\pi G_5}\int {\rm d}^5 x\sqrt{-g}\times\Bigg[\mathcal{R}-\Lambda+L^2\frac{\lambda_{\rm GB}}{2}\left(\mathcal{R}^2-4\mathcal{R}_{\mu\nu}\mathcal{R}^{\mu\nu}\right.\\ & \left.+\mathcal{R}_{\mu\nu\rho\sigma}\mathcal{R}^{\mu\nu\rho\sigma}\right)\Bigg]. \\[-1pt] \end{split} $

(30) The dimensionless Gauss-Bonnet coupling constant
$\lambda_{\rm GB}$ can be constrained by causality [23] and the positive boundary energy density on the boundary [55] satisfies$ -\frac{7}{36}<\lambda_{\rm GB}\le \frac{9}{100}. $

(31) A black hole solution in this case is known analytically [52]:
$ {\rm d}s^2=-n\frac{r^2}{L^2}f_{\rm GB}(r){\rm d}t^2+\frac{r^2}{L^2}{\rm d}\vec{x}^2+\frac{L^2}{r^2f_{\rm GB}(r)}{\rm d}r^2 , $

(32) where
$ \begin{split} f_{\rm GB}(r)&=\frac{1}{2\lambda_{\rm GB}}\left(1-\sqrt{1-4\lambda_{\rm GB}(1-r_+^4/r^4)}\right)\,,\\ n&=\frac{1}{2}\left(1+\sqrt{1-4\lambda_{\rm GB}}\right)\,. \end{split} $

(33) The boundary of the metric expressed by Eq. (32) is placed at
$ r\rightarrow \infty $ . We set the positive parameter n such that the speed of light of the boundary gauge theory is unity. As a result, we have$ f_{\rm GB}(r\xrightarrow{}\infty)=\frac{1}{n}. $

(34) The heat bath temperature of the black hole is given by
$ T_{\rm GB}=\frac{\sqrt{n}r_+}{\pi L^2}, $

(35) where
$ r_+ $ depends on$\lambda_{\rm GB}$ for a fixed Hawking temperature.Using a general form of metric, one has
$ \begin{split} g_{tt}(r)=-n\frac{r^2}{L^2}f_{\rm GB}(r),\qquad g_{ii}(r)=\frac{r^2}{L^2}, \qquad g_{rr}(r)=\frac{L^2}{r^2f_{\rm GB}(r)}. \end{split} $

(36) In our analysis, we set the
$ AdS_5 $ -radius to be unity for convenience. Using the same procedures as before, we can easily find the critical value$r^{c}_{\rm GB}$ where the numerator and denominator change sign at the same value,$ r^{c}_{\rm GB}=\frac{\sqrt{n}(r_+)}{\left(n (n-v^2)+\lambda_{\rm GB} v^4\right)^{\frac{1}{4}}}. $

(37) The world-sheet temperature of GB gravity of a quark feel is denoted as
$ T^{ws}_{\rm GB} $ and expressed as$ \begin{split} & T^{ws}_{\rm GB} = \frac{1}{2\pi} \left[\left| \frac{-4n\lambda_{\rm GB}(r_+)^8 +2n(r^c_{\rm GB})^4 r_+^4K}{\lambda_{\rm GB}(r^c_{\rm GB})^2 \left(\left(-1+4\lambda_{\rm GB} \right)(r^c_{\rm GB})^4-4\lambda_{\rm GB} r_+^4\right)} \right|\right]^{{1}/{2}},\\ & K=\left[-1+4\lambda_{\rm GB}+\sqrt{1+\lambda_{\rm GB}\left(-4+\frac{4r_+^4}{(r^c_{\rm GB})^4}\right)} \right]. \end{split} $

(38) Asserting Eq. (36) to Eqs. (17), (28), and (27), we obtain the longitudinal and perpendicular LGV-coefficients as
$ \begin{split} \kappa^{\perp}_{\rm GB} &=\frac{\sqrt{\lambda}}{\pi}(r^c_{\rm GB})^2T^{ws}_{\rm GB} \end{split} $

(39) and
$ \begin{split} \kappa^{\parallel}_{\rm GB} &= -\frac{-2\sqrt{\lambda}\lambda_{\rm GB}(r^c_{\rm GB})^4r_+^4+(r^c_{\rm GB})^8K}{2 \pi \lambda_{\rm GB} (r_{\rm GB}^c)^2r_+^4} T^{ws}_{\rm GB}. \end{split} $

(40) One can check that Eqs. (39) and (40) reduce to the results in the conformal limit given by Eq. (29) by taking the limit of
$\lambda_{\rm GB} \rightarrow 0$ .By employing GB gravity, we now discuss the
$ \mathcal{R}^2 $ corrections on the LGV-coefficients, normalized by the limits given in Eq. (29) for$ \mathcal{N}=4 $ SYM, with two scenarios at the same heat bath temperature ($T_{\rm GB}=T_{\rm SYM}$ ). Plots (a) and (b) in Fig. 2 depict$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ respectively as functions of moving velocity and$\lambda_{\rm GB}$ . It is evident that both$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ are independent of the values of moving velocity and$\lambda_{\rm GB}$ . Note that the correction behaviors to$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ are notably similar, and the universal relation$ \kappa_{\parallel} \geq\kappa_{\perp} $ identified in [17] also holds in the context of GB gravity.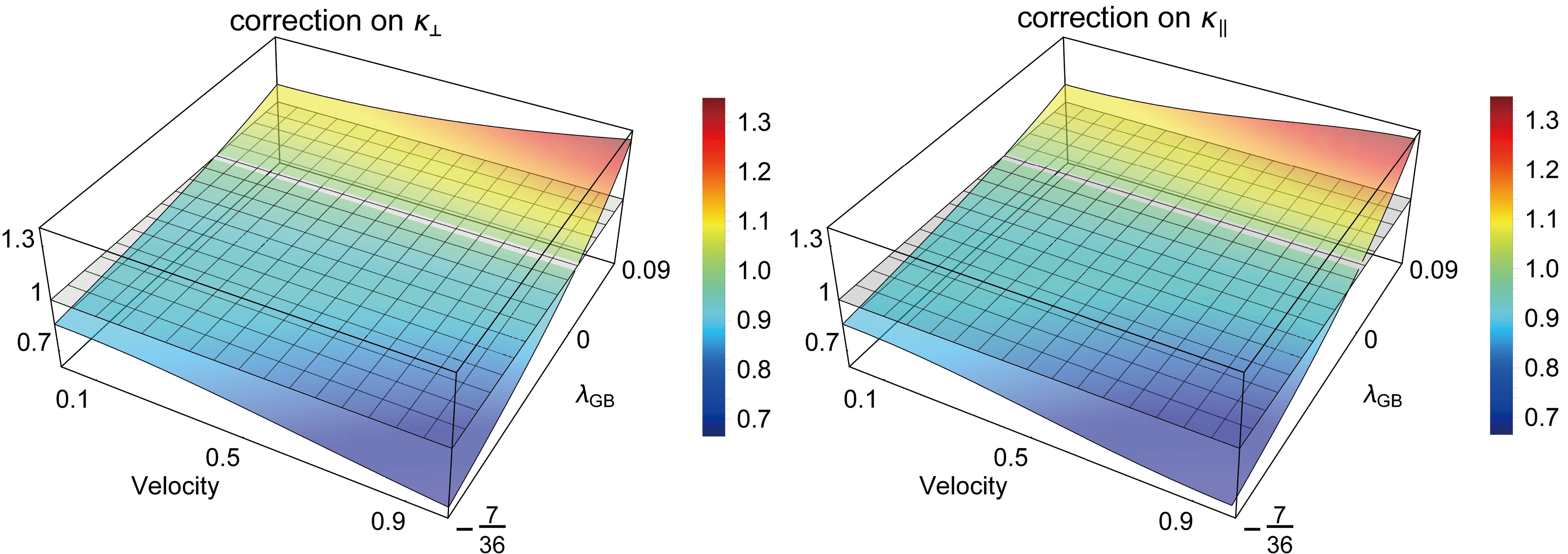
Figure 2. (color online) Corrections to the transverse LGV-coefficients
$ \kappa_{\perp} $ (left panel) and longitudinal LGV-coefficients$ \kappa_{\parallel} $ (right panel) as functions of both velocity and$ \lambda_{\rm GB} $ , normalized by the respective conformal limit.It was found that the results concerning LGV-coefficients in GB gravity when
$\lambda_{\rm GB}=0$ reduce to those of the case corresponding to$ \mathcal{N}=4 $ SYM. For$\lambda_{\rm GB} > 0$ , Fig. 2 demonstrates that the corrections to$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ become monotonically stronger with increasing velocity of the moving heavy quark or increasing$\lambda_{\rm GB}$ . Conversely, for$\lambda_{\rm GB} < 0$ ,$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ for a moving heavy quark under GB gravity become less than those in the$ \mathcal{N}=4 $ SYM case. Furthermore, the corrections to$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ increase monotonically with the absolute value of$ \lambda_{\rm GB} $ and with the growing velocity of the moving heavy quark. We conclude that the finite coupling corrections affect both$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ on a moving quark in the strongly-coupled plasma and depend on the details of curvature squared corrections. The LGV-coefficients can be larger or smaller than those in the infinite-coupling case. -
Using classical gravity to understand a quantum system is one of the most profound discoveries of contemporary theoretical physics. The majority of computations, achieved through classical two-derivative gravity calculations, hold strict validity within the context of a large 't Hooft coupling λ and the limit of color number
$ N_c $ . The modification of quenched jets provides one of the most effective tools for constraining properties of the QGP produced in heavy ion collisions. In this study, we investigated finite coupling corrections to heavy quark diffusion.We examined the influences from curvature-squared
$ \mathcal{R}^2 $ corrections on the AdS black brane metric to LGV-coefficients with both a more general$ \mathcal{R}^2 $ gravity and GB gravity. Our investigation revealed that finite coupling corrections can indeed impact the$ \kappa_{\perp} $ and$ \kappa_{\parallel} $ values of a moving heavy quark. Both$ \kappa_{L} $ and$ \kappa_{T} $ can be larger or smaller than the values in the infinite coupling case, and the specific correction behaviors depend on the details of higher derivative gravity. We confirmed the persistence of the universal relation$ \kappa_{L}\geq\kappa_{T} $ across all cases we examined. Both corrected$ \kappa_{L} $ and$ \kappa_{T} $ values can either increase or decrease in comparison with those in the infinite coupling scenario in GB background. Our findings regarding curvature squared corrections to$ \kappa_{L} $ and$ \kappa_{T} $ closely resemble the features obtained for the drag force on a moving heavy quark discussed in [45]. Finally, we must emphasize that we did not predict the effects of finite 't Hooft correction to$ \mathcal{N} = 4 $ SYM, given that the leading correction in gauge theory emerges at order$ R^4 $ .
${\mathcal{{{R}}}^{\boldsymbol{2}}}$ curvature-squared corrections on Langevin diffusion coefficients
- Received Date: 2024-07-08
- Available Online: 2025-01-15
Abstract: The effect of finite coupling corrections to the Langevin diffusion coefficients on a moving heavy quark in the Super Yang-Mills plasma was investigated. These corrections are related to curvature squared corrections in the corresponding gravity sector. We compared the results of both longitudinal and perpendicular Langevin diffusion coefficients with those for







 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF














 DownLoad:
DownLoad: