Search for invisible decays of the Higgs boson produced at the CEPC

Many pieces of cosmological evidence point towards the existence of dark matter (DM), such as rotation curves in galaxies, the masses of clusters of galaxies, and the gravitational lensing of galaxies [1, 2]. However, there is no candidate for DM in the Stand Model (SM). In collider physics, the Higgs boson might be the portal connecting the new physics, such as DM and the fourth generation neutrino, and the SM [3-5]. In this case, the DM particles, which interact weakly with ordinary matter and are completely invisible to detectors, can be observed indirectly by studying the Higgs decays. In the SM, the Higgs boson can only decay invisibly via $ H\rightarrow ZZ^\ast \rightarrow \nu\overline{\nu}\nu\overline{\nu} $, as shown in Fig. 1, and its branching ratio (BR) is 1.06$ \times10^{-3} $ [6]. Therefore, any evidence of invisible Higgs decays that exceed this BR will indicate a phenomenon that is beyond the SM (BSM).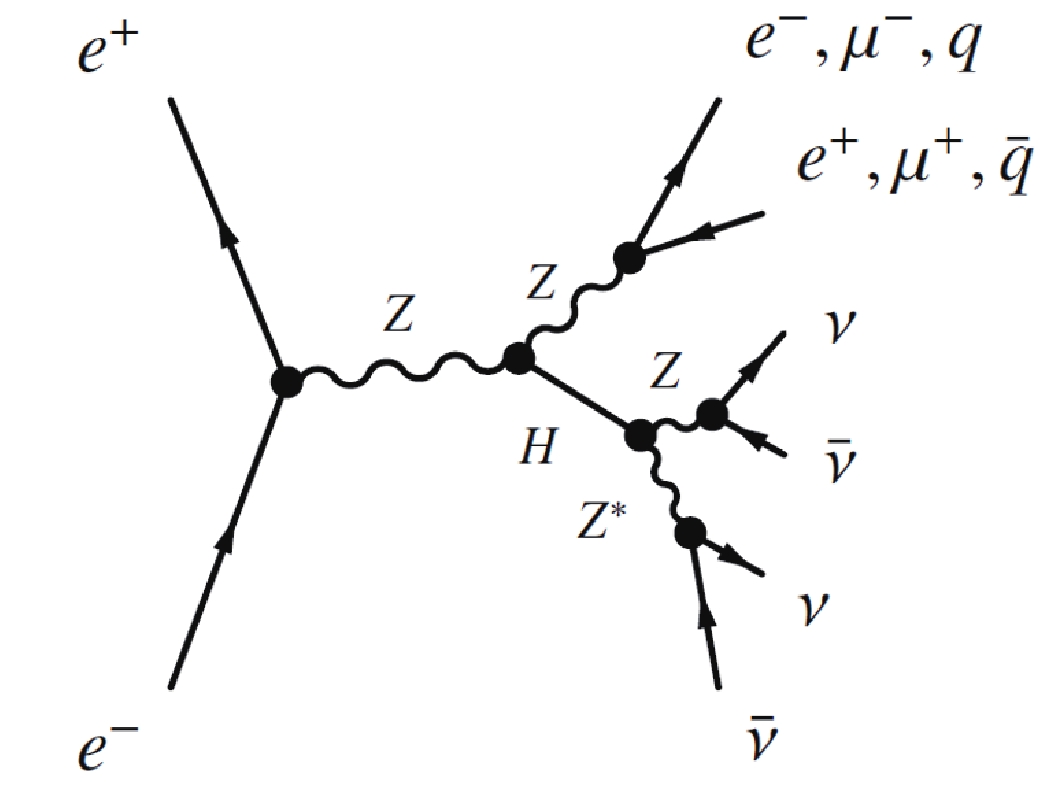

Figure 1.  Feynman diagrams of the Higgs boson's invisible decays at an electron-positron collider such as the CEPC. In the process $e^+e^- \rightarrow ZH$, the invisible decays of the Higgs boson are via $H\rightarrow ZZ^\ast \rightarrow \nu\overline{\nu}\nu\overline{\nu}$.

The search for the invisible decays of the Higgs boson has been performed at the Large Hadron Collider (LHC). The signature for the invisible Higgs decays at the LHC is a large missing transverse momentum recoiling against a visible system. ATLAS and CMS yielded the 95% confidence level (CL) upper limits of 26% [7] and 19% [8], respectively, for the Higgs boson invisible branching ratio (BR($ H \rightarrow $ inv)). These results were obtained by the ATLAS and CMS detectors, respectively, using combined 4.7 fb⁻¹, 20.3 fb⁻¹, 36.1 fb⁻¹ and combined 4.9 fb⁻¹, 19.7 fb⁻¹, 38.2 fb⁻¹ of proton-proton collisions at the center-of-mass energy of 7 TeV, 8 TeV, and 13 TeV at the LHC. Compared with the results reported by the LHC, Higgs boson candidates can be identified using a technique known as the recoil mass method without using its decays at the Circular Electron Positron Collider (CEPC) [6]. An example of the recoil mass method is as follows: in the $ e^{+}e^{-} $$ \rightarrow $ $ ZH(Z \rightarrow f\bar{f} $, $ H \rightarrow $ inv) channel, fermions (f) can be identified, and their momentum can be measured. By selecting the fermion pair from the Z boson decay, the mass of the system recoiling against the fermion pair, commonly known as the recoil mass $ M_{\rm{recoil}} $, can be calculated as

where $ E_{f\bar{f}} $ and $ P_{f\bar{f}} $ are the total energy and the momentum of the two fermions, respectively, and $ \sqrt{s} $ is the center-of-mass energy. The $ M_{\rm{recoil}} $ distribution is expected to exhibit a peak at the Higgs boson mass around 125 GeV for the $ f\bar{f} $$ \rightarrow ZH $ process. In this way, the properties of the Higgs boson can be measured precisely without reconstructing the Higgs boson from its decay products. Therefore, the Higgs boson production can be disentangled from its decay in a model-independent way. Moreover, the $ e^+e^- $ collisions have a much lower hadronic background (Higgs boson channels form the signal) contamination compared with hadron collisions, which allows better exclusive measurements of the Higgs boson decay channels. The electron-positron Higgs factory is an essential machine for understanding the nature of the Higgs boson.

CEPC is a Higgs factory proposed by the Chinese high energy physics community. CEPC is designed for delivering a combined integrated luminosity of 5.6 ab⁻¹ to two detectors in 7 years. CEPC will operate at a center-of-mass energy $ \sqrt{s} $ $ \sim $ 240-250 GeV, and over one million Higgs boson events will be produced during this period. Owing to the large statistics, the good beam energy spread of approximately 0.16% [9], and a novel particle flow algorithm [10], the mass and width of the Higgs boson are expected to be measured with high precision. With the SM $ ZH $ production rate, the upper limit of BR($ H \rightarrow $ inv) could reach 0.26% at the 95% CL, which is an expected improvement of two orders of magnitude over the results of ATLAS and CMS.

In a previous study on CEPC, the upper limit of BR($ H \rightarrow $ inv) was 0.41% [6]. The previous study used the CEPC-v1 detector, whereas the CEPC-v4 detector is used in this study. The main change from CEPC-v1 to CEPC-v4 is the reduction of the solenoidal field intensity from 3.5 Tesla to 3.0 Tesla and changing the$ \sqrt{s} $ of the collider from 250 GeV to 240 GeV. Moreover, the reconstruction algorithm is different in both studies. Therefore, this study does not involve the comparison of the two results.

This study performs three independent analyses corresponding to $ \mu\mu H $, $ eeH $, and $ qqH $ channels, for estimating the upper limit on the BR($ H \rightarrow $ inv) measurement at the CEPC. This paper is organized as follows. Section 2 presents a brief introduction to the CEPC detector and Monte Carlo simulations. Section 3 presents an introduction to the event selection of the three channels. A method for determining the upper limit, along with dependence of the Boson Mass Resolution (BMR), is discussed in Section 4. Section 5 lists our conclusions.

One of the physics programs at the CEPC is precision measurement of the Higgs boson properties. The CEPC detector is expected to reconstruct and identify all key physical objects, including charged leptons, photons, jets, missing energy, and missing momentum.

The CEPC-v4 [6] detector was designed using the International Large Detector (ILD) [11, 12] as a reference. The detector of CEPC-v4 is simulated using MokkaC [13] and Geant4 [14]. It is composed of a tracking system, Time-Projection-Chamber tracker (TPC), high granularity calorimeter system, solenoid that generates a 3 Tesla magnetic field, and muon detector embedded in a magnetic field return yoke. The tracking system consists of silicon vertexing and tracking detectors. The calorimetry system consists of an electromagnetic calorimeter (ECAL) and an iron scintillator for a hadronic calorimeter (HCAL).

The analysis is performed on Monte Carlo (MC) samples simulated at the CEPC-v4 detector. The Higgs boson signal and SM backgrounds at the center-of-mass energy of 240 GeV, corresponding to the overall luminosity of 5.6 ab⁻¹, are generated with WHIZARD1.95 [15]. The generated events are then processed with MokkaC, and an attempt is made to reconstruct every visible particle with ARBOR [10]. The cross sections of the major SM processes of the $ e^{+}e^{-} $ collisions as functions of the center-of-mass energy $ \sqrt{s} $ are used in the simulations, including the Higgs boson production as well as the major backgrounds, where the initial-state radiation (ISR) effect has been taken into account. The Higgs boson signal and backgrounds are processed using Geant4 based full detector simulations and reconstruction. Limited by finite computational resources, approximately 20% of the two fermion backgrounds are used in full simulations.

All samples are grouped into signal and backgrounds, and the backgrounds are classified according to their final states. For the signal, this paper mainly focuses on the process $ e^{+}e^{-}\rightarrow ZH $, which is called the “$ ZH $” process. Then, Z bosons decay into leptons or hadrons, and the Higgs particle decays into two Z bosons, which eventually decay into four neutrinos. For the backgrounds, the major SM backgrounds are divided into 2-fermion processes and 4-fermion processes, according to the final states. The 2-fermion backgrounds are $ e^{+}e^{-} $$ \rightarrow $ $ f\bar{f} $, where f refers to all lepton and quark pairs, except $ t\overline{t} $. The 4-fermion backgrounds are divided into 6 types: “single_z,” “single_w,” “szorsw,” “zz,” “ww,” and “zzorww,” which are shown in Table 1. The processes whose four final states are a pair of electrons and two other fermions, or a pair of electron neutrinos and two other fermions, are named “single_z”. The “single_w” processes include one electron, one electron neutrino, and two other fermions. If a final state includes a pair of electrons and a pair of electron neutrinos simultaneously, the corresponding processes are named “szorsw”. In addition to the above-mentioned backgrounds, the same four fermions in the final state can be combined into different two intermediate bosons. If the two intermediate bosons can be two Z bosons, the processes are named “zz”. The “ww” process is the one in which two intermediate bosons can become two W bosons. If two intermediate bosons can become two Z bosons or two W bosons, the corresponding process is “zzorww”.

The signal in this analysis consists of three different channels, namely $ ZH(Z \rightarrow qq, H \rightarrow $ inv), $ ZH(Z \rightarrow \mu\mu, H \rightarrow $ inv), and $ ZH(Z \rightarrow ee, H \rightarrow $ inv). Table 2 lists detailed information about the Higgs boson decay channels. The observed upper limit on BR($ H \rightarrow $ inv) at 95% CL at the CMS is 19%, and the CEPC is expected to yield more accurate results. In the event selection part, this analysis uses BR($ H \rightarrow $ inv) = 10%, and the event selection is based on the distribution of the signal and backgrounds. Event selection for each channel is detailed below.

Process	Cross sections /fb	Expected
$ffH$	203.66	1140496
$e^{+}e^{-} H$	7.04	39424
${\mu^+}{\mu^-} H$	6.77	37912
$q\bar{q} H$	136.81	766136

Table 2.  Cross sections of the Higgs boson production at $\sqrt{s}$ = 240 GeV and number of events expected in 5.6 ab⁻¹.

In the $ ZH(Z \rightarrow qq, H \rightarrow $ inv) process, owing to the presence of quarks, many final states are expected. The event selection uses the information about all visible particles, and the distributions of the signal and backgrounds are shown in Fig. 2. The comprehensive event selections are as follows. In the $ e^+e^- \rightarrow ZH(Z \rightarrow qq, H \rightarrow $ inv) process, the mass of the system recoiling against all visible particles from the Z boson is $ M_{\rm{recoil}}^{\rm{visible}} $, which can be calculated using Eq. (1) by replacing $ E_{f\bar{f}} $, $ P_{f\bar{f}} $ with $ E_{\rm{visible}} $, $ P_{\rm{visible}} $. $ E_{\rm{visible}} $ and $ P_{\rm{visible}} $ is the total energy and momentum of all visible particles. The peak of the $ M_{\rm{recoil}}^{\rm{visible}} $ distribution is close to the Higgs boson mass. Considering the resolution of the detector, $ M_{\rm{recoil}}^{\rm{visible}} $ is limited to (100,150) GeV, as shown in Fig. 2(a). To suppress 2-fermion backgrounds, the transverse momentum of all visible particles is required to satisfy $ P_{\rm{T}}^{\rm{visible}}> $ 18 GeV, as shown in Fig. 2(b), and the difference between the azimuthal angles of the two jets should be below 175°. Two jets are reconstructed from Z boson decay particles. $ E_{\rm{visible}} $ is the energy of all visible particles, which can be described as

Figure 2.  (color online) The distributions of $M_{\rm{recoil}}^{\rm{visible}}$, $P_{\rm{T}}^{\rm{visible}}$, $E_{\rm{visible}}$, and $M_{\rm{visible}}$ for signal and backgrounds in the $ZH(Z \rightarrow qq, H \rightarrow$ inv) process with BR($H \rightarrow$ inv) = 10% are plotted with all cuts except the ones shown already applied in the figure and the subsequent cuts (based on Table 3). The blue arrows mark the cut ranges.

where $ M_{\rm{recoil}}^{\rm{visible}} $ is approximately 125 GeV, $ \sqrt{s} = 240 $ GeV, and the invariant mass of the visible system ($ M_{\rm{visible}} $) is equal to the Z boson mass, which is 91.2 GeV. $ M_{\rm{visible}} $ should be limited to (85,102) GeV, as shown in Fig. 2(c). Using the values of the parameters in Eq. (2), $ E_{\rm{visible}} $ should be near 105 GeV, as shown in Fig. 2(d). According to the equation $ P_{\rm{visible}}^2 = E_{\rm{visible}}^2 - M_{\rm{visible}}^2 $, $ P_{\rm{visible}} $ should be near 52 GeV. Owing to the presence of quarks, the final states may include many charged particles. It is necessary to limit the number of charged particles ($ N_{\rm{charged}} $) with energy greater than 1 GeV to be larger than 5. To suppress the backgrounds from tau particles, a dedicated tau-finding algorithm TAURUS has been developed [16]. Since the $ ZH(Z \rightarrow qq, H\rightarrow $ inv) process may include tau particles, the mass of all tau particles ($ M_{\tau} $) should be less than 95 GeV, for suppressing the backgrounds containing tau and quarks.

Table 3 lists the yields of signal ($ qqH $_inv) and its backgrounds of the cut chain. The value of the significance [17] is used to judge the effect of the cuts. After the event selection, the signal selection efficiency is 60.81%, and the total background rejection efficiency is 99.97%. The backgrounds, which contain neutrinos and two quarks, account for 95% of the total remaining backgrounds. The compositions of these backgrounds are similar to the signal channel and are difficult to suppress further.

Process	$qqH$_inv	2f	single_w	single_z	szorsw	zz	ww	zzorww	ZH_visible	total_bkg	Significance
Total generated	76614	801152072	19517400	9072952	1397088	6389432	50826216	20440840	1140496	909936496	2.54
100 GeV$<M_{\rm{recoil}}^{\rm{visible}}<$150 GeV	73800	47294924	1388875	822729	229217	507567	1752827	658204	97387	52751730	10.16
18 GeV$<P_{\rm{T}}^{\rm{visible}}<$60 GeV	67115	9165311	1000762	269328	152273	282630	1294265	462029	79965	12706563	18.81
90 GeV$<E_{\rm{visible}}<$117 GeV	63912	5748712	595697	223049	92958	231058	785392	272518	33705	7983089	22.59
85 GeV$<M_{\rm{visible}}<$102 GeV	53786	605791	238191	148850	39280	135641	392277	113043	18284	1691357	41.14
$\Delta\phi_{\rm{dijet}}<175^ \circ $	51911	390077	230273	141494	38359	129135	379931	109735	17395	1436399	43.06
30 GeV$<P_{\rm{visible}}<$58 GeV	48572	241510	148607	69457	24393	46807	226883	74781	13466	845904	52.32
$N_{\rm{charged}}>5,E_{\rm{charged}}>1$GeV	47772	7986	18399	62990	6	43728	121365	4110	11699	270283	89.36
$M_{\tau}<$95 GeV	46589	7111	11044	59815	1	41180	104784	3126	11111	238172	92.58
Efficiency	60.81%	0.00%	0.06%	0.66%	0.00%	0.64%	0.21%	0.02%	0.97%	0.03%

Table 3.  Yields for backgrounds and $ ZH(Z \rightarrow qq, H \rightarrow $ inv) signal at the CEPC, with $ \sqrt{s} $ = 240 GeV, BR($ H \rightarrow $ inv) = 10%, and integrated luminosity of 5.6 ab⁻¹.

The $ ZH(Z \rightarrow \mu^+\mu^-, H \rightarrow $ inv) process and the $ ZH(Z \rightarrow e^+e^-, H \rightarrow $ inv) process are similar, and the two processes will be introduced together. Firstly, it is natural that only a pair of oppositely charged muons or electrons is required in the visible final states. By selecting two muons or two electrons, many related parameters can be used for background suppression. The event selections are as follows. The recoil masses of two muons ($ M_{\rm{recoil}}^{\mu^{+}\mu^{-}} $) and two electrons ($ M_{\rm{recoil}}^{e^{+}e^{-}} $) can be calculated using Eq. (1). The peak of $ M_{\rm{recoil}}^{\mu^{+}\mu^{-}} $ and $ M_{\rm{recoil}}^{e^{+}e^{-}} $ distribution should be around the Higgs boson mass 125 GeV. Considering the resolutions of muons and electrons and the distributions of signal and backgrounds as shown in Fig. 3 and Fig. 4, the recoil mass should satisfy 120 GeV$ <M_{\rm{recoil}}^{\mu^{+}\mu^{-}}< $150 GeV or 120 GeV$ <M_{\rm{recoil}}^{e^{+}e^{-}}< $170 GeV, and the invariant mass of two muons ($ M_{\mu^{+}\mu^{-}} $) or two electrons ($ M_{e^{+}e^{-}} $) is closer to the Z boson mass. To suppress the 2-fermion backgrounds, the transverse momenta of the muon pair ($ P_{\rm{T}}^{\mu^+\mu^-} $) and the electron pair ($ P_{\rm{T}}^{e^{+}e^{-}} $) are required to be more than 12 GeV, as shown in Fig. 3(c) and Fig. 4(c). Moreover, the angle between the two muons ($ \Delta\phi_{\mu^+\mu^-} $) should be under 175° or that between the two electrons ($ \Delta\phi_{e^+e^-} $) should be under 176° to suppress the 2-fermion backgrounds. The visible energy ($ E_{\rm{visible}} $), which is described in Eq. (2), is mainly the energy of two muons ($ E_{\mu^{+}\mu^{-}} $) or two electrons ($ E_{e^{+}e^{-}} $) from the Z boson decays; the value of $ E_{\rm{visible}} $ is approximately 105 GeV, as shown in Fig. 3(d) and Fig. 4(d). Using the approximate values of $ M_{\mu^{+}\mu^{-}} $ and $ E_{\mu^{+}\mu^{-}} $ in the relativistic energy-momentum relation $ M_{\mu^{+}\mu^{-}}^2 = E_{\mu^{+}\mu^{-}}^2- P_{\mu^{+}\mu^{-}}^2 $, the value of $ {E_{\mu^{+}\mu^{-}}}\big/{P_{\mu^{+}\mu^{-}}} $ is close to 2, similar to that of $ {E_{e^+e^-}}\big/{P_{e^+e^-}} $.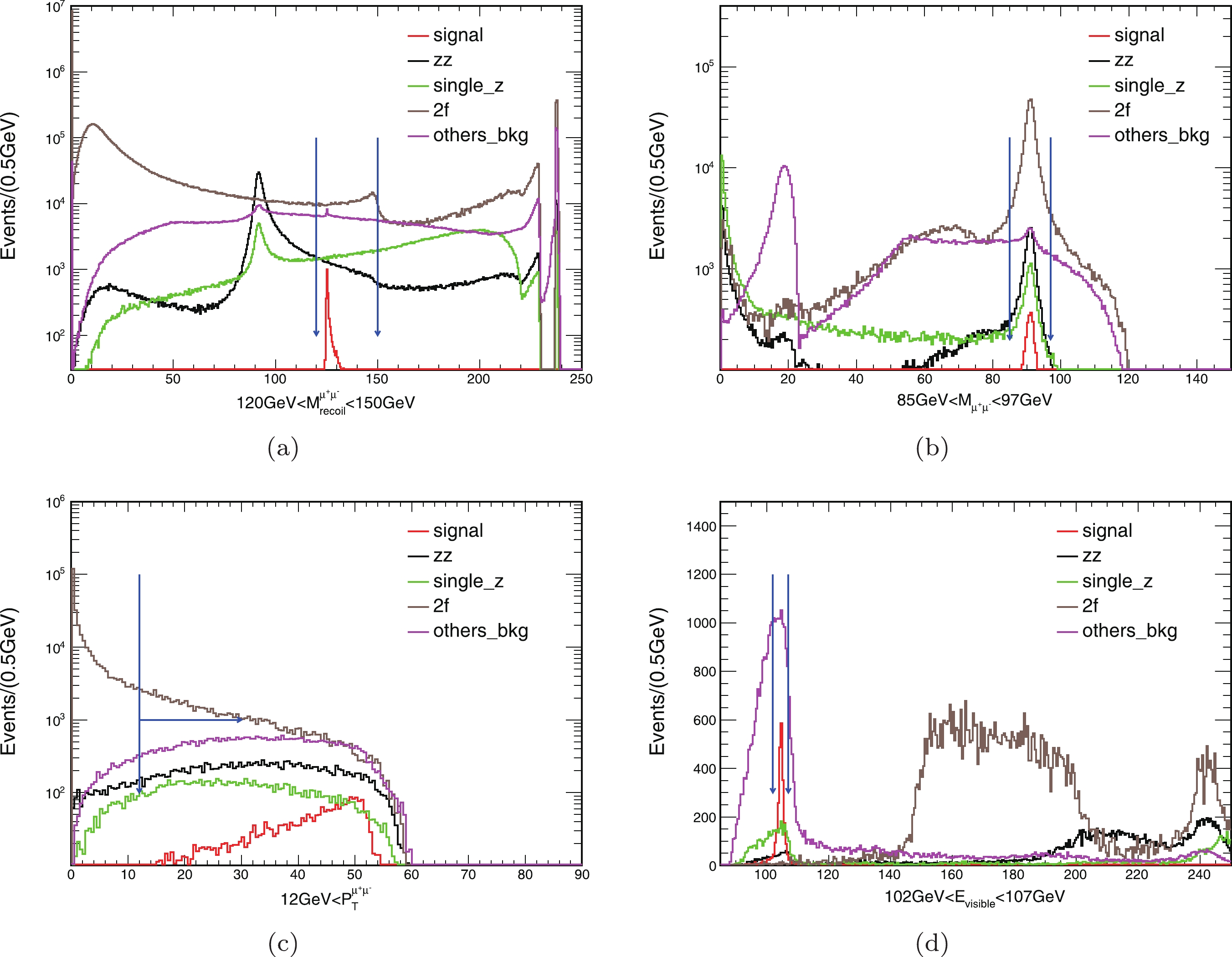

Figure 3.  (color online) The distributions of $M_{\rm{recoil}}^{\mu^{+}\mu^{-}}$, $P_{\rm{T}}^{\mu^+\mu^-}$, $E_{\rm{visible}}$, and $M_{\mu^+\mu^-}$ for signal and backgrounds in the $ZH(Z \rightarrow \mu^+\mu^-, H \rightarrow$ inv) process with BR($H \rightarrow$ inv) = 10% are plotted with all cuts except the ones shown already applied in the figure and the subsequent cuts (based on Table 4). The blue arrows mark the cut ranges.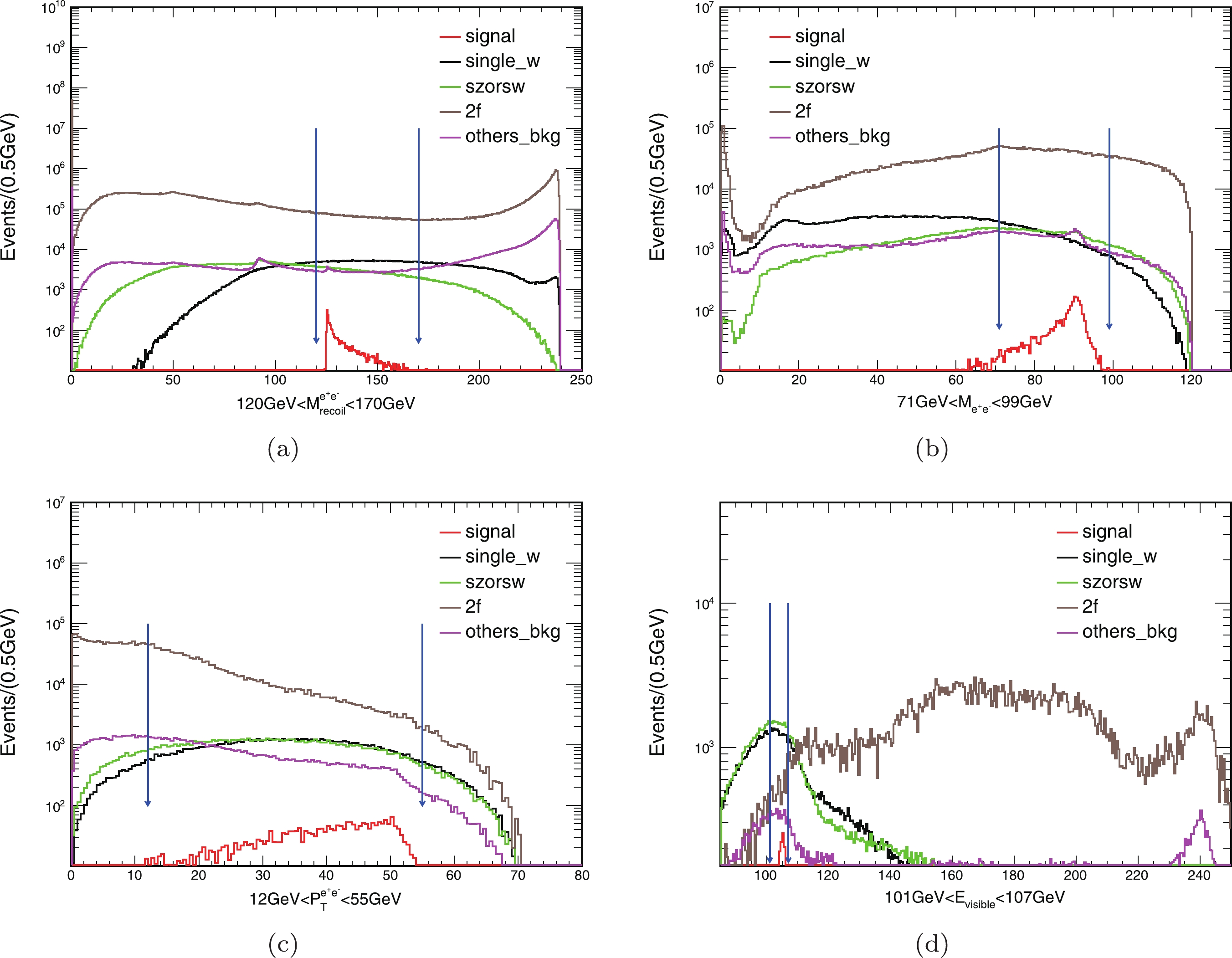

Figure 4.  (color online) The distributions of $M_{\rm{recoil}}^{e^+e^-}$, $P_{\rm{T}}^{e^+e^-}$, $E_{\rm{visible}}$, and $M_{e^+e^-}$ for signal and backgrounds in the $ZH(Z \rightarrow e^+e^-, H \rightarrow$ inv) process with BR($H \rightarrow$ inv) = 10% are plotted with all cuts except the ones shown already applied in the figure and the subsequent cuts (based on Table 5). The blue arrows mark the cut ranges.

Table 4 lists the yields for the $ \mu\mu H $_inv signal and its backgrounds. The remaining backgrounds containing two muons and two neutrinos account for 61% of the total backgrounds. These backgrounds have similar topology as the signal which is difficult to suppress further. The remaining backgrounds containing the muon, tau, and two neutrinos account for 38% of the total backgrounds. The algorithm TAURUS does not increase the significance of the $ \mu\mu H $_inv signal.

Process	$\mu\mu H$_inv	2f	single_w	single_z	szorsw	zz	ww	zzorww	ZH	total_bkg	Significance
Total generated	3791	801152072	19517400	9072952	1397088	6389432	50826216	20440840	1140496	909936496	0.13
$N_{\mu^{+}}=1,N_{\mu^{-}}=1$	3370	22737312	36123	723402	0	702045	1255617	1223596	59978	26738073	0.65
120 GeV$<M_{\rm{recoil}}^{\mu^{+}\mu^{-}}<$150 GeV	3286	652655	24	100435	0	62463	250819	112143	5708	1184247	3.02
85 GeV$<M_{\mu^{+}\mu^{-}}<$97 GeV	2791	381056	0	10739	0	20857	16720	24419	4493	458284	4.12
12 GeV$<P_{\rm{T}}^{\mu^{+}\mu^{-}}$	2705	92200	0	9483	0	18257	15906	21063	4331	161240	6.72
$\Delta\phi_{\mu^{+}\mu^{-}}<175^ \circ $	2598	72197	0	8894	0	17029	14769	20231	4142	137262	6.99
102 GeV$<E_{\rm{visible}}<$107 GeV	2273	62	0	1456	0	484	4379	5435	10	11826	20.28
$\frac{E_{\mu^{+}\mu^{-}}}{P_{\mu^{+}\mu^{-}}}<2.4$	2243	27	0	1344	0	440	3502	4090	6	9409	22.29
Efficiency	59.17%	0.00%	0.00%	0.01%	0.00%	0.01%	0.01%	0.02%	0.00%	0.00%

Table 4.  Yields for backgrounds and $ ZH(Z \rightarrow \mu^+\mu^-, H \rightarrow $ inv) signal at the CEPC, with $ \sqrt{s} $ = 240 GeV, BR($ H \rightarrow $ inv) = 10% and integrated luminosity of 5.6 ab⁻¹.

Table 5 lists the yields for the $ eeH $_inv signal and its backgrounds at the CEPC. The cut Vertex$ _{\tau}< $0.0011, which is the position of the decay vertex, changes the value of significance from 10.91 to 13.79. Since the signal channel does not contain tau, the Vertex$ _{\tau} $ of the signal channel is much smaller than the backgrounds from tau. The final states of the remaining backgrounds, which are composed of two electrons and two neutrinos, account for 70% of the total background. These backgrounds are the same as the final particles of the signal channel. The final particles of the remaining backgrounds containing tau, electron, and two neutrinos account for 23% of the total background, and the information about tau particles cannot further suppress these backgrounds. In conclusion, the tau-finding algorithm TAURUS can improve the significance of the Higgs invisible decays to a certain extent, but it cannot completely suppress the backgrounds containing tau.

Process	$eeH$_inv	2f	single_w	single_z	szorsw	zz	ww	zzorww	ZH	total_bkg	Significance
Total generated	3942	801152072	19517400	9072952	1397088	6389432	50826216	20440840	1140496	909936496	0.13
$N_{e^{+}}=1,N_{e^{-}}=1$	3472	120476492	1286971	1945217	1161098	134637	796860	292694	117024	126210993	0.31
120 GeV$<M_{\rm{recoil}}^{e^{+}e^{-}}<$170 GeV	3179	6469896	515411	226357	288321	4901	12836	34015	12443	7564180	1.16
71 GeV$<M_{e^{+}e^{-}}<99$GeV	2617	2415241	98434	69803	105255	453	926	10853	9153	2710118	1.59
12 GeV$<P_{\rm{T}}^{e^{+}e^{-}}<$55 GeV	2511	1351168	87124	45901	91180	352	788	9396	8774	1594683	1.99
$\Delta\phi_{e^{+}e^{-}}<176^ \circ $	2397	462573	81317	37220	87409	208	712	8613	8456	686508	2.89
101 GeV$<E_{\rm{visible}}<$107 GeV	1614	6555	15198	2820	17583	12	54	1175	47	43444	7.70
$1.8<\frac{E_{e^+e^-}}{P_{e^+e^-}}<2.4$	1455	1423	6634	1127	7685	4	17	393	23	17306	10.91
${\rm{Vertex}}_{\tau}$$<$0.0011	1393	323	2436	926	5967	1	7	86	9	9755	13.79
Efficiency	35.34%	0.00%	0.01%	0.01%	0.43%	0.00%	0.00%	0.00%	0.00%	0.00%

Table 5.  Yields for backgrounds and $ ZH(Z \rightarrow e^+e^-, H \rightarrow $ inv) signal at the CEPC, with $ \sqrt{s} $ = 240 GeV, BR($ H \rightarrow $ inv) = 10%, and integrated luminosity of 5.6 ab^-1.

After the event selections, the 95% CL upper limit of BR($ H \rightarrow $ inv) is computed within the CL$ _{s} $ formalism, using the profile likelihood ratio as a test statistic [18], in which systematic uncertainties are ignored. The likelihood ratio method uses $ \mu $S+B, where $ \mu $ is the signal strength, S is the signal, and B is the background. First, the signal and background samples are fitted to obtain their distribution functions, which are used for generating Asimov data. The Asimov data provide a simple method for obtaining the median experiment sensitivity of the measurement as well as fluctuations around this expectation. Then, the test statistic distribution generated for signal+background and background-only hypotheses is constructed assuming the signal strength $ \mu $, and each $ \mu $ corresponds to the CL$ _{s} $ value calculated by the ratio of the two hypothesis probabilities [19]. When the CL$ _{s} $ value is 0.05, the $ \mu $ value is its 95% CL upper limit. The corresponding negative logarithmic profile likelihood ratio $-\Delta$log(L) as a function of $ \mu $ is shown in Fig. 5. The horizontal axis corresponding to $-\Delta$log(L) = 2 on the y-axis is approximately 95% CL interval of $ \mu $.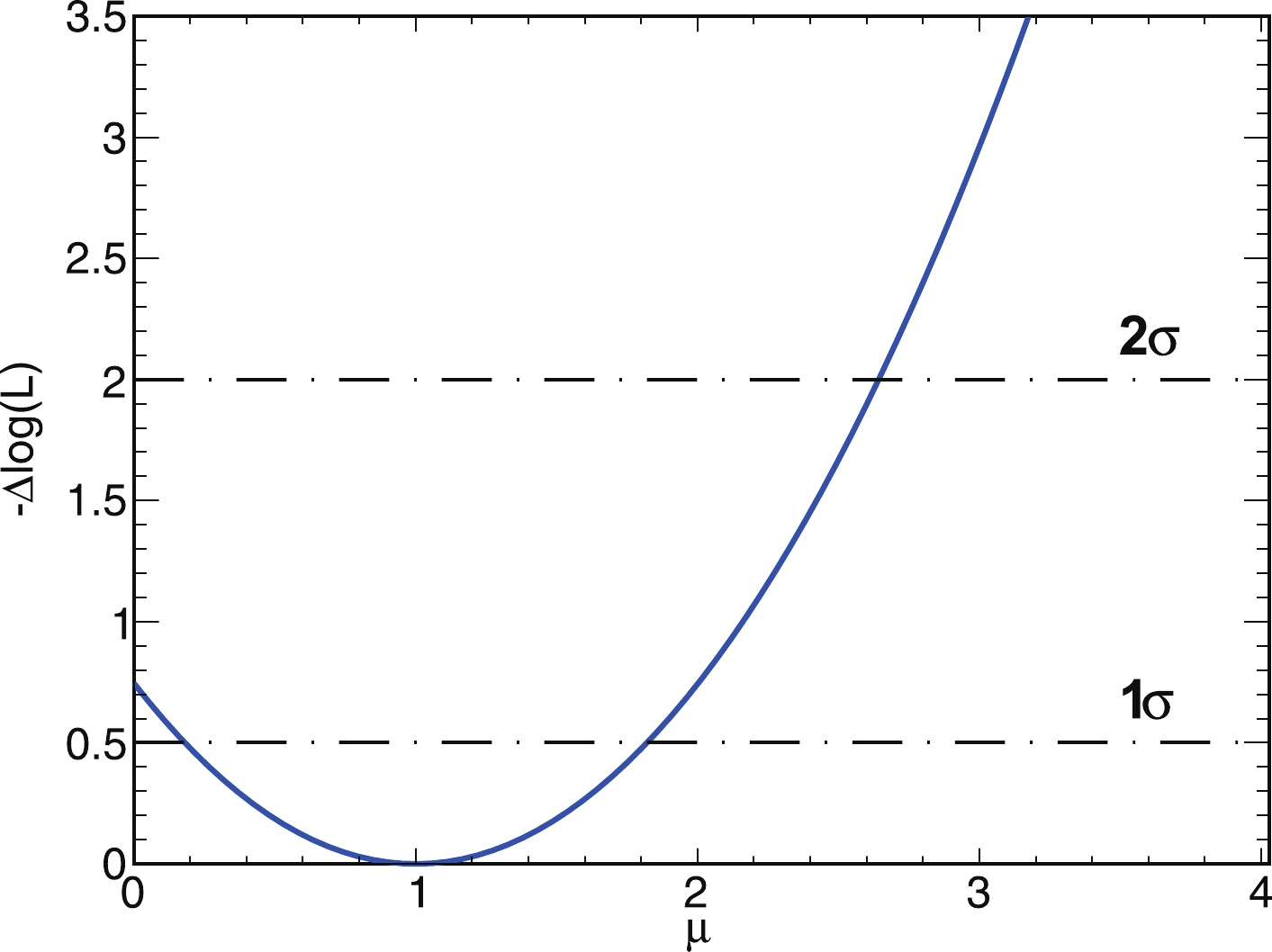

Figure 5.  (color online) The $\mu$ distribution from the likelihood profile, where the horizontal dash-dotted lines indicate the location of the approximately 68%, 95% CL interval, which corresponds to $-\Delta$log(L) = 0.5, 2 on the y-axis.

Table 6 summarizes the expected precision of the measurement of BR($ H \rightarrow $ inv) and the 95% CL upper limit on BR($ H \rightarrow $ inv), for the dataset of 5.6 ab^-1. The estimated combined 95% CL upper limit of three channels is 0.26%. Any evidence of invisible Higgs decays that exceeds this value will indicate the BSM phenomenon.

$ZH$ final states	Precision of BR($H \rightarrow$ inv)$\times$100 (%)	Upper limit on BR($H \rightarrow$ inv) (%)
$Z \rightarrow e^+e^-$, $H \rightarrow$ inv	45.37	1.08
$Z \rightarrow \mu^+\mu^-$, $H \rightarrow$ inv	23.57	0.55
$Z \rightarrow q\overline{q}$, $H \rightarrow$ inv	9.54	0.27
Combination	8.68	0.26

Table 6.  Expected precision of the measurement of BR($H \rightarrow$ inv) and the 95% CL upper limit on BR($H \rightarrow$ inv) for the dataset 5.6 ab⁻¹.

The precision of the upper limit is affected by various systematic uncertainties [20], such as the luminosity, beam energy, efficiency of the object reconstruction, and acceptance of the detector. The precision of luminosity can be 0.1%, and the beam energy is expected to be better than 1 MeV, which can be ignored in experimental recoil mass measurements. For tracks within the detector acceptance and transverse momenta larger than 1 GeV, the track finding efficiency is better than 99%. These systematic uncertainties are expected to be small and will be ignored in this study.

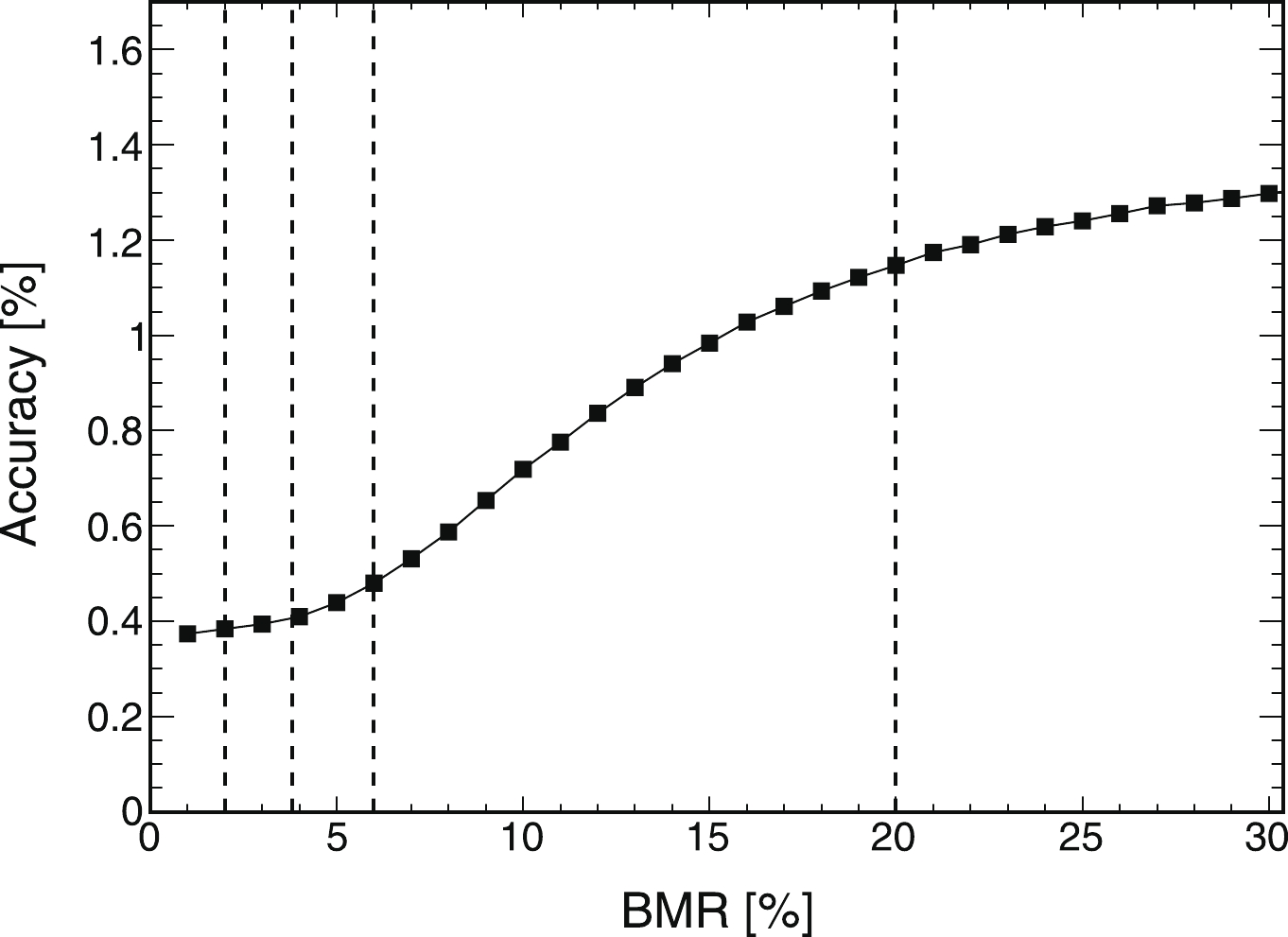
The precision of the upper limit of BR($ H \rightarrow $ inv) in $ ZH(Z \rightarrow qq, H \rightarrow $ inv) channel strongly relies on the invariant mass reconstruction of the Z boson. The boson mass resolution (BMR) is defined as the visible invariant mass resolution of the $ ZH(Z \rightarrow qq, H \rightarrow $ inv) event for quantifying the invariant mass reconstruction of the Z boson.

The BMR of the CEPC detector can reach 3.8% using the ARBOR reconstruction algorithm [10, 21]. A fast simulation was performed for quantifying this dependence. The fast simulation took into account the signal of $ ZH(Z \rightarrow qq, $$H \rightarrow $ inv) and the main background of $ ZZ (Z \rightarrow qq, Z \rightarrow $ inv) after the event selection. Fig. 6 shows the accuracy [16] of $ ZH(Z \rightarrow qq, H \rightarrow $ inv) for different BMR values. For BMR values between 4% and 20%, the accuracy degrades rapidly with increasing BMR, while for BMR values below 4%, the change in the accuracy is below 0.06%. Based on the fast simulation, it can be concluded that BMR is vital and will affect the measurement precision of the $ ZH(Z \rightarrow qq, H \rightarrow $ inv) channel. Therefore, the BMR value of 4% can be used as an essential reference upper bound for detector design and optimization.

Figure 6.  Accuracy of the qqH (H$\rightarrow$inv) channel vs. BMR, for the background of $ZZ(Z \rightarrow qq, Z \rightarrow$ inv). The dashed vertical lines show the accuracy at BMR is 2%, 3.8%, 6%, and 20%, assuming BR($H \rightarrow$ inv) = 10%.

This paper studied the measurement of the Higgs invisible decays at the CEPC. The upper limit on the Higgs invisible decays was measured using three independent channels $ ZH(Z \rightarrow qq, H \rightarrow $ inv), $ ZH(Z \rightarrow \mu^+\mu^-, H \rightarrow $ inv), and $ ZH(Z \rightarrow e^+e^-, H \rightarrow $ inv). The combined result for the 95% CL upper limit of BR($ H \rightarrow $ inv) was 0.26% for the three channels. Compared with the LHC results (26% for ATLAS and 19% for CMS), the result obtained at the CEPC is better by two orders of magnitude. Compared with the High-Luminosity LHC (HL-LHC) result for 14 TeV, which is expected to be 2.5% [22], the result obtained at the CEPC is better by one order of magnitude. The accuracy of the upper limit for the CEPC is significantly better than those for hadron colliders, because the reconstructed Higgs recoil mass spectrum at the electron-positron Higgs factories gives a very clear and distinct signature of the Higgs boson, as well as the high productivity of the Higgs bosons at the CEPC.

The CEPC result is consistent with the results for other electron-positron colliders, such as the International Linear Collider (ILC) and the Future Circular Collider (FCC-ee), for which the 95% CL upper limits on BR($ H \rightarrow $ inv) were 0.26% for ILC [23] and 0.22% for FCC-ee (5 ab^-1 at 240 GeV and 0.19% by combining 365 GeV) [24]. Among these three signal channels, the $ qqH $ channel yielded the best result owing to its largest number of events. The precision of the upper limit on the $ qqH $ channel strongly relies on the invariant mass of the visible hadronic decay system, and BMR better than 4% provides a clear separation between the Higgs signal and the $ ZZ $ background, which shall be pursued as one of the key physics requirements for designing future CEPC detectors.

The authors would like to thank Chengdong FU and Xianghu ZHAO for providing the simulation tools and samples. We also thank Patrick Janot for a helpful discussion about FCC results.