-
Quantum chromodynamics (QCD) is the theory of the strong interaction between quarks mediated by gluons, which are color-charged [1]. In principle, QCD allows complex quark and gluon compositions of hadrons, such as multiquark hadrons, hadronic molecules, hybrid hadrons, and glueballs, which are nonstandard hadronic particles. Most of these nonstandard hadrons have unusual masses, decay widths, etc., which cannot be given a satisfactory explanation by the traditional quark model. To date, more than thirty non-
$ q\bar{q} $ state candidates in light and heavy sectors have been reported experimentally [2]. These resonances are crucial for the deep understanding of hadron spectroscopy and the nonperturbative nature and spontaneous chiral symmetry breaking of QCD.In the low-lying scalar meson sector,
$ f_0(980) $ [3],$ a_0(980) $ [4], and$ D^\ast_{s0}(2317) $ [5] have nonexotic$ J^P (=0^+) $ quantum numbers. However, their masses are much lower than the quark model expectation for the corresponding P-wave$ q\bar{q} $ states [6, 7]. Their natures are still under debate in spite of the efforts during the past several decades. Since their masses are near the threshold of the constituent particles and have spin-parity quantum numbers corresponding to the S-wave combinations of the constituent particles, one would naturally identify them as hadronic molecules, which are analogs of nuclei.$ f_0(980) $ and/or$ a_0(980) $ could be$ K\bar{K} $ molecules [8–15] and$ D^\ast_{s0}(2317) $ could be a$ DK $ molecule [16–26]. Such a picture leads to results consistent with the experiments. In addition to these particles, the possible S-wave bound states of the$ \bar{B}{K} $ ,$ D\bar{D} $ ,$ B\bar{B} $ ,$ BD $ ,$ \bar{B}\bar{K} $ ,$ DD $ ,$ \bar{B}\bar{B} $ , and$ \bar{B}D $ systems have not been observed experimentally.On the theoretical aspect, the authors in Ref. [26] systematically studied the possible S-wave bound state of two pseudoscalar mesons by the nonrelativistic Schr
$\mathrm{\ddot{o}}$ dinger (NRS) equation. Reference [18] predicted the existence of a$ B\bar{K} $ bound state$ B_{s0}^\ast $ with a mass of 5.725$ \pm $ 0.039 GeV based on the heavy chiral unitary approach. Subsequently, Refs. [27] and [28] confirmed the existence of$ B_{s0}^\ast $ in the$ B\bar{K} $ bound state scenario and further studied the decay widths of its possible decay channels. Recently, Kong et al. [24] systematically investigated$ DK $ /$ \bar{B}K $ and$ \bar{D}K $ /$ BK $ systems in a quasipotential Bethe-Salpter equation (qBSE) approach by considering the light meson exchange potential and found that only the isoscalar systems can exist as molecular states. However, the mass of$ X(5568) $ reported by the D0 collaboration [29] is considerably below the$ BK $ threshold, and hence, it cannot be a$ BK $ molecule [30–35]. In Ref. [36], the authors reported their findings on the S-wave$ D\bar{D} $ ,$ BD $ , and$ B\bar{B} $ systems in the chiral SU(3) quark model (QM); their calculations favor the existence of the isoscalar$ B\bar{B} $ molecule, but the existence of isovector$ D\bar{D} $ and$ BD $ molecules is disfavored. The authors of Ref. [37] studied$ D^{(\ast)}D^{(\ast)} $ and$ B^{(\ast)}B^{(\ast)} $ molecular states by solving the coupled channel Schr$ \mathrm{\ddot{o}} $ dinger (CCS) equations; only$ I(J^P)=1(0^+) BB $ can be a bound state in the$ PP $ ($ P=D, B $ ) system because the kinetic term is suppressed in the bottom sector, and the effect of channel couplings becomes more important. With the qBSE approach [38], the existence of$ D\bar{D} $ and$ B\bar{B} $ molecular states with$ I(J^P)=0(0^+) $ were predicted, yet no bound state was produced from the$ DD $ and$ \bar{B}\bar{B} $ interaction. In Ref. [39], a new hidden charm resonance with a mass of 3.7 GeV was predicted within the coupled channel unitary approach. Later, the$ D\bar{D} $ bound state was searched in several processes, such as$ B \rightarrow D \bar{D} K $ [40],$ \psi (3770) \rightarrow \gamma D^0 {\bar{D}}^0 $ [41], and$ \gamma\gamma \rightarrow D\bar D $ [42, 43]. There are some differences in the results of different methods. Therefore, more efforts are needed to investigate the possible S-wave bound state composed of two pseudoscalar mesons.In the present study, we systematically investigate whether the S-wave bound states of two-pseudoscalar meson systems exist in the Bethe-Salpeter (BS) approach (in the ladder approximation and the instantaneous approximation for the kernel). For doubly heavy pseudoscalar meson systems, we consider not only the interaction through exchanged light mesons (ρ, ω and σ) but also the contribution of heavy vector mesons (
$ J/\psi $ or$\Upsilon $ ). As reported in Refs. [44, 45], in spite of the large mass of$ J/\psi $ , which suppresses the propagator of the exchanged$ J/\psi $ , it was found that the interaction could bind the$ D^\ast\bar{D}^\ast $ and$ D\bar{D}^\ast $ systems. Similarly, Refs. [38, 46] also reported that the contribution from a heavy meson exchange ($ J/\psi $ or$\Upsilon $ ) is very important to form a molecular state, especially in systems in which the contributions from ρ and ω cancel each other out.The remainder of this paper is organized as follows. In Sec. II, we discuss and establish the BS equation for two-pseudoscalar meson systems. This equation is solved numerically, and the numerical results of the two-pseudoscalar meson systems are presented in Sec. III. In the last section, we summarize the study.
-
The BS wave function for the bound state
$ |P\rangle $ composed of two pseudoscalar mesons have the following form:$ \begin{equation} \chi\left(x_1,x_2,P\right) = \langle0|T{\cal{P}}_1(x_1){\cal{P}}_2(x_2)|P\rangle, \end{equation} $

(1) where
$ {\cal{P}}_1(x_1) $ and$ {\cal{P}}_2(x_2) $ are the field operators of the two constituent particles at space coordinates$ x_1 $ and$ x_2 $ , respectively. The BS wave function in momentum space is defined as$ \begin{equation} \chi_P(x_1,x_2,P) = {\rm e}^{-{\rm i}PX}\int\frac{{\rm d}^4p}{(2\pi)^4}{\rm e}^{-{\rm i}px}\chi_P(p), \end{equation} $

(2) where p represents the relative momentum of the two constituent particles, and
$ p= \lambda_2 p_1-\lambda_1 p_2 $ ($ p_1=\lambda_1P+p $ ,$ p_2=\lambda_2P-p $ ) with$ \lambda_1 = m_1/(m_1 + m_2) $ and$\lambda_2 = m_2/(m_1 + m_2)$ .$ p_{1(2)} $ and$ m_{1(2)} $ represent the momentum and mass of the constituent particle, respectively.The BS wave function
$ \chi_P(p) $ satisfies the following BS equation:$ \begin{equation} \chi_{P}(p)=S_{{\cal{P}}_1}(p_1)\int\frac{{\rm d}^4q}{(2\pi)^4}K(P,p,q)\chi_{P}(q)S_{{\cal{P}}_2}(p_2), \end{equation} $

(3) where
$ S_{{\cal{P}}_1}(p_1) $ and$ S_{{\cal{P}}_2}(p_2) $ are the propagators of constituent particles, and$ K(P,p,q) $ is the kernel, which is defined as the sum of all two-particle irreducible diagrams.In the following, we use the variables
$ p_l (=p\cdot v) $ and$ p_t(=p- p_lv) $ as the longitudinal and transverse projections of the relative momentum (p) along the bound state velocity (v), respectively. Then, the propagators of the constituent mesons can be expressed as$ \begin{equation} S_{{\cal{P}}_1}(\lambda_1P+p)=\frac{\rm i}{\left(\lambda_1M+p_l\right)^2-\omega_1^2+{\rm i}\epsilon}, \end{equation} $

(4) and
$ \begin{equation} S_{\bar{{\cal{P}}}_2}(\lambda_2P-p)=\frac{\rm i}{\left(\lambda_2M-p_l\right)^2-\omega_2^2+{\rm i}\epsilon}, \end{equation} $

(5) where
$ \omega_{1(2)} = \sqrt{m_{1(2)}^2+p_t^2} $ (we have defined$ p_t^2=-p_t\cdot p_t $ ).To obtain the interaction kernel of the two-pseudoscalar meson system through exchanging light and heavy vector mesons and the light scalar meson, the following effective Lagrangians, as in Refs. [38, 47, 48], are needed:
$ \begin{aligned}[b] {\cal{L}}_{KK\mathbb{V}}=& {\rm i}g_{KK\rho}\vec{\rho}^\mu\cdot\left(K^\dagger\vec{\tau}\partial_\mu K-\partial_\mu K^\dagger\vec{\tau} K\right)\\&+{\rm i}\left(g_{KK\omega}\omega^\mu+g_{KK\omega}\phi^\mu\right)(K^\dagger\partial_\mu K-\partial_\mu K^\dagger K),\\ {\cal{L}}_{DD\mathbb{V}}=& {\rm i}g_{{DD}\mathbb{V}}(D_b \partial_\alpha D_a^\dagger-D_a^\dagger\partial_\alpha D_b)\mathbb{V}^\alpha_{ba}\\&+{\rm i}g_{DDJ/\psi}\left(D \partial_\alpha D^\dagger-D^\dagger\partial_\alpha D\right)J/\psi^\alpha,\\ {\cal{L}}_{BB\mathbb{V}}=& {\rm i}g_{{BB}\mathbb{V}}(B_b \partial_\alpha B_a^\dagger-B_a^\dagger\partial_\alpha B_b)\mathbb{V}^\alpha_{ba}\\&+{\rm i}g_{BB\Upsilon}\left(B \partial_\alpha B^\dagger-B^\dagger\partial_\alpha B\right)\Upsilon^\alpha,\\ {\cal{L}}_{DD\sigma}=& g_{DD\sigma}D_aD^\dagger_a\sigma, \,\,\,\,\,\,{\cal{L}}_{BB\sigma}= g_{BB\sigma}B_aB^\dagger_a\sigma, \end{aligned} $

(6) where
$ J/\psi^\alpha $ ,$ \Upsilon^\alpha $ , and σ represent the$ J/\psi $ ,$\Upsilon $ , and σ field operators, respectively, and the nonet vector meson matrix reads as$ \begin{eqnarray} \mathbb{V}&=&\left(\begin{array}{ccc} \dfrac{\rho^{0}}{\sqrt{2}}+\dfrac{\omega}{\sqrt{2}}&\rho^+&K^{*+}\\ \rho^-&-\dfrac{\rho^{0}}{\sqrt{2}}+\dfrac{\omega}{\sqrt{2}}& K^{*0}\\ K^{*-} &\bar{K}^{*0}&\phi \end{array}\right). \end{eqnarray} $

(7) The coupling constants involved in Eq. (6) are taken as
$ g_{KK\rho}=g_{KK\omega}=g_{KK\phi}=3 $ ,$g_{DD\mathbb{V}}=g_{BB\mathbb{V}}= {\beta g_v}/{\sqrt{2}}$ with$ g_v = 5.8 $ ,$ \beta = 0.9 $ ,$ g_{DDJ/\phi}=m_{J/\psi}/f_{J/\psi} $ with$ f_{J/\psi}=405 $ MeV, and$ g_{BB\Upsilon}=m_{\Upsilon}/f_{\Upsilon} $ with$ f_{\Upsilon}=715.2 $ MeV.In the so-called ladder approximation, the interaction kernel
$ K(P,p,q) $ can be derived in the lowest-order form as follows:$ \begin{aligned}[b]\\[-8pt] K(p_1,p_2;q_1,q_1,m_V)=&-(2\pi)^2\delta^4(q_1+q_2-p_1-p_2) C_Ig_{PPV}g_{P'P'V}(p_1+q_1)_\mu(p_2+q_2)_\nu\Delta^{\mu\nu}(k,m_V),\\ K(p_1,p_2;q_1,q_1,m_\sigma)=&-(2\pi)^2\delta^4(q_1+q_2-p_1-p_2) C_Ig_{PP\sigma}^2\Delta_\sigma(k,m_\sigma),\\ \end{aligned} $ 
(8) where
$ m_V $ represent the masses of the exchanged light and heavy vector mesons (ρ, ω, ψ,$ J/\psi $ , and$\Upsilon $ ).$ \Delta^{\mu\nu}(k,m_V) $ and$ \Delta_\sigma(k,m_\sigma) $ represent the propagators for the vector and scalar mesons, respectively, and have the following forms:$ \begin{equation} \begin{split} \Delta^{\mu\nu}(k,m_V)=\frac{-\rm i}{k^2-m_V^2}\left(g^{\mu\nu}-\frac{k^\mu k^\nu}{m_V^2}\right),\quad\quad \Delta_\sigma(k,m_\sigma)=\frac{\rm i}{k^2-m_\sigma^2}. \end{split} \end{equation} $

(9) $ C_I $ in Eq. (8) is the isospin coefficient for$ I=0 $ and$ I=1 $ . For the$ K\bar{K} $ ,$ DK $ ,$ \bar{B}{K} $ ,$ D\bar{D} $ ,$ B\bar{B} $ , and$ BD $ systems,$ \begin{equation} C_0=\left\{\begin{array}{rl}3/2&\; \; {\rm for}\; \rho\\1/2&\; \; {\rm for}\; \omega\\1&\; \; {\rm for}\; \phi\\1&\; \; {\rm for}\; J/\psi\\1&\; \; {\rm for}\; \Upsilon\\1&\; \; {\rm for}\; \sigma\end{array}\right.,\; \; \; \; \; \; \; \; \; \; \; \; \; \; C_1=\left\{\begin{array}{rl}-1/2&\; \; {\rm for}\; \rho\\1/2&\; \; {\rm for}\; \omega\\1&\; \; {\rm for}\; \phi\\1&\; \; {\rm for}\; J/\psi\\1&\; \; {\rm for}\; \Upsilon\\1&\; \; {\rm for}\; \sigma\end{array}\right. . \end{equation} $

(10) For the
$ \bar{K}\bar{K} $ ,$ D\bar{K} $ ,$ \bar{B}\bar{K} $ ,$ DD $ ,$ \bar{B}\bar{B} $ , and$ \bar{B}D $ systems,$ \begin{equation} C_0=\left\{\begin{array}{rl}3/2&\; \; {\rm for}\; \rho\\-1/2&\; \; {\rm for}\; \omega\\-1&\; \; {\rm for}\; \phi\\-1&\; \; {\rm for}\; J/\psi\\-1&\; \; {\rm for}\; \Upsilon\\1&\; \; {\rm for}\; \sigma\end{array}\right.,\; \; \; \; \; \; \; \; \; \; \; \; \; \; C_1=\left\{\begin{array}{rl}-1/2&\; \; {\rm for}\; \rho\\-1/2&\; \; {\rm for}\; \omega\\-1&\; \; {\rm for}\; \phi\\-1&\; \; {\rm for}\; J/\psi\\-1&\; \; {\rm for}\; \Upsilon\\1&\; \; {\rm for}\; \sigma\end{array}\right.. \end{equation} $

(11) In Eqs. (10) and (11), the exchanged mesons of ϕ,
$ J/\psi $ , and Υ only appear for the$ K\bar{K} $ /$ \bar{K}\bar{K} $ ,$ D\bar{D} $ /$ DD $ , and$ B\bar{B} $ /$ \bar{B}\bar{B} $ systems, and σ is only considered in the doubly heavy pseudoscalar meson systems.In order to manipulate the off shell effect of the exchanged mesons and finite size effect of the interacting hadrons, we introduce a form factor
$ {\cal{F}}(k^2) $ at each vertex. Generally, the form factor has the following form:$ \begin{equation} {\cal{F}}_M(k^2)=\frac{\Lambda^2-m^2}{\Lambda^2-k^2}, \end{equation} $

(12) where Λ, m, and k represent the cutoff parameter, mass, and momentum of the exchanged meson, respectively. This form factor is normalized at the on shell momentum of
$ k^2=m^2 $ . In contrast, if$ k^2 $ is taken to be infinitely large ($ -\infty $ ), the form factor, which can be expressed as the overlap integral of the wave functions of the hadrons at the vertex, would approach zero. Considering the difference in the wave functions and masses of the light and heavy mesons, and ensuring a positive form factor, different magnitudes of cutoff Λ will be chosen for the heavy and light mesons.The propagators (4) and (5), interaction kernel (8), and form factor (12) are substituted into the BS equation, i.e., Eq. (3), and the instantaneous approximation (
$ p_l=q_l $ , where the energy exchanged between the constituent particles of the binding system is neglected) in the kernel is considered. Consequently, when a vector meson and a scalar meson in the center-of-mass frame of the bound state ($ \vec{P} = 0 $ ) are exchanged, Eq. (3) becomes$ \begin{aligned}[b]\\[-7pt] \chi_P(p_l,\vec{p}_t)=\frac{\rm i}{\left[\left(\lambda_1M+p_l\right)^2-\omega_1^2+{\rm i}\epsilon\right]\left[\left(\lambda_2M-p_l\right)^2-\omega_2^2+{\rm i}\epsilon\right]}\int\frac{{\rm d} q_l}{2\pi}\frac{{\rm d}^3\vec{q}_t}{(2\pi)^3} \end{aligned} $ 
$ \begin{aligned} \quad\quad &\times \Bigg\{C_Ig_{PPV}g_{P'P'V}\frac{4\left(\lambda_1M+p_l\right)\left(\lambda_2-p_l\right)+\left(\vec{p}_t+\vec{q}_t\right)^2+\left(\vec{p}_t^2-\vec{q}_t^2\right)/m_V^2}{-\left(\vec{p}_t-\vec{q}_t\right)-m_V^2} \frac{\left(\Lambda^2-m_V^2\right)^2}{\left[\Lambda^2+\left(\vec{p}_t+\vec{q}_t\right)^2\right]^2}\\ &+C_Ig_{PP\sigma}g_{P'P'\sigma}\frac{1}{-\left(\vec{p}_t-\vec{q}_t\right)-m_\sigma^2}\frac{\left(\Lambda^2-m_\sigma^2\right)^2}{\left[\Lambda^2+\left(\vec{p}_t+\vec{q}_t\right)^2\right]^2}\Bigg\}\chi_P(q_l,\vec{q}_t). \end{aligned}$

(13) In the above equation, there are poles in the
$ p_l $ plane at$-\lambda_1M-\omega_1+{\rm i}\epsilon$ ,$-\lambda_1M+\omega_1-{\rm i}\epsilon$ ,$\lambda_2M+\omega_2-{\rm i}\epsilon$ , and$\lambda_2M-\omega_2+{\rm i}\epsilon$ . After integrating$ p_l $ on both sides of Eq. (13) by selecting the proper contour, we can obtain the three-dimensional integral equation for$ \tilde{\chi}_P(\vec{p}_t) $ ($\tilde{\chi}_P(\vec{p}_t)= \int {\rm d} p_l\chi_P(p_l,\vec{p}_t)$ ), which only depends on the the three momentum,$ \vec{p}_t $ . By completing the azimuthal integration, the three-dimensional BS equation becomes a one-dimensional integral equation as$ \begin{equation} \tilde{\chi}_P(|\vec{p}_t|)=\int {\rm d} |\vec{p}_t|A\left(|\vec{p}_t|,|\vec{q}_t|\right)\tilde{\chi}_P(|\vec{q}_t|), \end{equation} $

(14) where the propagators and kernels after one-dimensional simplification are included in
$ A\left(|\vec{p}_t|,|\vec{q}_t|\right) $ . The numerical solutions for$ \tilde{\chi}_P(|\vec{p}_t|) $ can be obtained by discretizing the integration region into n pieces (with n sufficiently large). In this way, the integral equation becomes a matrix equation, and the BS scalar function$ \tilde{\chi}_P(|\vec{p}_t|) $ becomes an n dimensional vector. -
In this section, we will solve the BS equation numerically and study whether the S-wave bound states composed of two pseudoscalar mesons exist. In our model, there is only one parameter, the cutoff Λ, which comes from the form factor. The binding energy
$ E_b $ is defined as$ E_b=M-m_1-m_2 $ in the rest frame of the bound state. We take the averaged masses of the pseudoscalar mesons and the exchanged light and heavy mesons from PDG [2],$ m_K $ = 494.988 MeV,$ m_D $ = 1868.04 MeV,$ m_{B} $ = 5279.44 MeV,$ m_{\rho} $ = 775.26 MeV,$ m_{\omega} $ = 782.65 MeV,$ m_{\phi} $ = 1019.461 MeV,$ m_{J/\psi} $ = 3096.9 MeV, and$ m_\Upsilon $ = 9460.3 MeV.In Fig. 1, we present some possible bound states composed of two pseudoscalar mesons when only the light meson (ρ, ω, ϕ, and σ) exchange contributions are considered. Here, we vary the binding energy from 0 to –50 MeV and the cutoff in a wide range of 0.8–5 GeV. We find that only the
$ K\bar{K} $ ,$ DK $ ,$ \bar{B}{K} $ ,$ D\bar{D} $ ,$ B\bar{B} $ ,$ BD $ ,$ D\bar{K} $ ,$ \bar{B}\bar{K} $ , and$ \bar{B}D $ systems with$ I=0 $ can exist as bound states. The$ \bar{K}\bar{K} $ ,$ DD $ , and$ \bar{B}\bar{B} $ systems with$ I=0 $ are forbidden because of Bose symmetry, and the interactions in$ I=1 $ systems are repulsive; hence, no bound states exist in the$ \bar{K}\bar{K} $ ,$ DD $ , and$ \bar{B}\bar{B} $ systems. Furthermore, we cannot predict with certainty the masses of bound states that will be measured experimentally because our results are dependent on the cutoff Λ. The contribution of the σ exchange is included in our work, despite the large uncertainties in its mass and structure. In our previous works [49, 50] and Ref. [51], it was found that the contribution of the σ exchange is too small to form bound states, and the same result is found in our current work.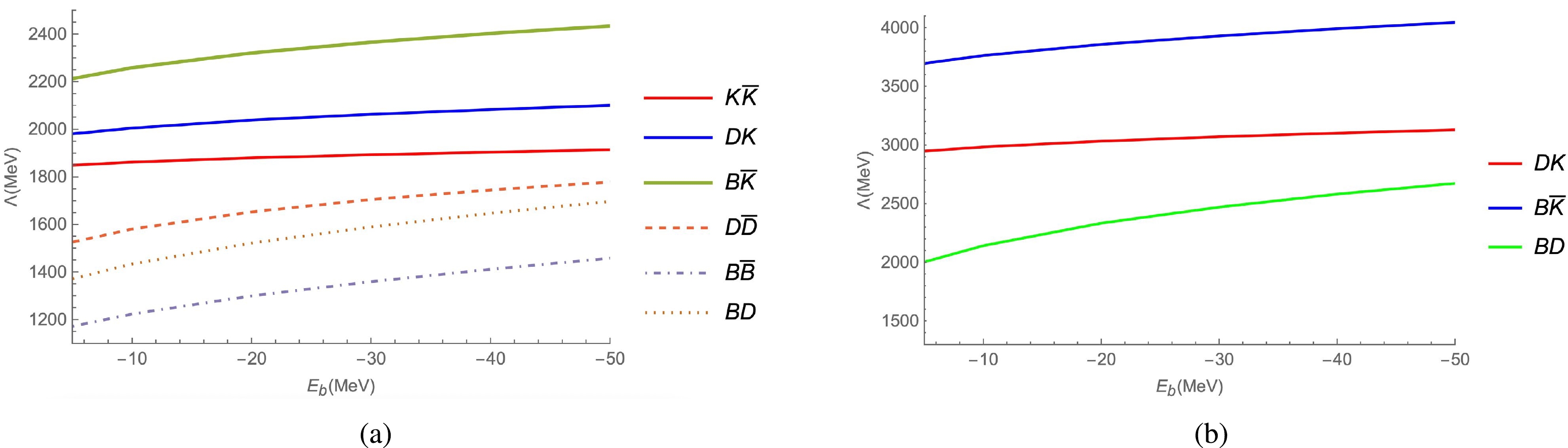
Figure 1. (color online) The numerical results for
$ K\bar{K} $ ,$ DK $ ,$ \bar{B}{K} $ ,$ D\bar{D} $ ,$ B\bar{B} $ , and$ BD $ systems with$ I=0 $ (a) and$ D\bar{K} $ ,$ \bar{B}\bar{K} $ , and$ \bar{B}D $ systems with$ I=0 $ (b).The systems that may exist as bound states are presented in Fig. 1. It is noted that, in the hidden bottom system, the cutoff is the smallest in Fig. 1(a). This is because the B meson has the largest mass, which requires a smaller cutoff value as compared with those in the other systems, and the cutoff is determined by the overlap integrals of the wave functions and hadrons at the vertices. The size of the B meson is the smallest among the constituent particles. There is no bound state for the system with
$ I=1 $ . This is because the isospin coefficients of ρ and ω are –1/2 and 1/2, respectively, as shown in Eq. (10), and the masses of ρ and ω are almost equal. Therefore, contributions from the ρ and ω exchanges almost cancel each other out. Among these possible bound states, the$ K\bar{K} $ and$ DK $ bound states can be related to the experimentally observed$ f_0(980) $ and$ D_{s0}^\ast(2317) $ , respectively [52]. Based on the heavy chiral unitary approach [18] and linear chiral symmetry [53], the authors predicted the existence of a b-partner state$ B_{s0}^\ast $ of$ D_{s0}^\ast(2317) $ as the$ B\bar{K} $ bound state, which can also be confirmed in our model with the cutoff Λ = 2436 MeV. The experimentally observed$ X(5568) $ cannot be a$ B\bar{K} $ bound state in our model [35]. In Ref. [39], a new hidden charm resonance with a mass of 3.7 GeV (named as$ X(3700) $ ) was predicted corresponding mostly to a$ D\bar{D} $ state. Later, it has been searched in$ B\rightarrow D\bar{D}K $ [40],$ e^+e^-\rightarrow J/\psi D\bar{D} $ [54],$ \psi(3770)\rightarrow X(3700)\gamma $ [55],$ \gamma\gamma\rightarrow D\bar{D} $ [42, 43],$ \Lambda_b\rightarrow \Lambda D\bar{D} $ [56], etc. Recently, lattice QCD also found a$ D\bar{D} $ bound state just below the threshold with the binding energy$ E_b=-4.0^{+3.7}_{-5.0} $ MeV [57]. The existence of the$ B\bar{B} $ bound state was also confirmed by the effective potential model [58], the heavy quark effective theory [59], the chiral SU(3) QM [36], and the qBSE [38]. The$ BD $ system, analogous to the$ DK $ system, can also be a bound state in the local hidden gauge symmetry (HGS) approach [60].Recently, the
$ T_{cc} $ with the quantum number$ I(J^P)=0(1^+) $ and quark content$ cc\bar{u}\bar{d} $ was reported by the LHCb Collaboration [61], which is the first experimentally discovered open charmed tetraquark state. The$ T_{cc} $ has a mass just below the threshold of$ DD^\ast $ and can be an ideal candidate for the$ DD^\ast $ bound state. In fact, this inspired us to investigate the possibility of two-pseudoscalar meson systems as bound states with open flavor. In Ref. [62], the authors systematically investigated possible deuteron-like molecular states with two heavy quarks using the one-boson-exchange (OBE) model. According to their results, the$ I=1 $ $DD $ system might not be a molecule; the$ I=1 $ $\bar{B}\bar{B} $ ,$ I=0 $ , and$ I=1 $ $D\bar{B} $ systems might be molecule candidates, but the results are sensitive to the cutoff. Based on the Heavy-Meson Effective Theory, the systems of$ DD $ with$ I=1 $ ,$ \bar{B}\bar{B} $ with$ I=1 $ , and$ D\bar{B} $ with$ I=0 $ and$ I=1 $ can exist as shallow bound states [63].According to the results of the two-pseudoscalar meson systems, which are presented in Fig. 1(b), only the isoscalar system can exist as bound states. This is because, for the isovector systems, the isospin coefficients corresponding to the ρ and ω exchanges are –1/2, as shown in Eq. (11); hence, the total interaction is repulsive in the isovector systems. Comparing the results in Fig. 1 (a) and Fig. 1(b), we can see that the cutoff Λ is larger in Fig. 1(b), which is caused by the difference in the isospin coefficients, i.e., 1/2 and –1/2 in the systems with
$ I=0 $ due to the ω exchange in Eq. (10) and Eq. (11), respectively. From Fig. 1(a) and Fig. 1(b), we can also find that, for the constituent particles with same masses, the larger the mass of the constituent particle, the smaller the cutoff Λ; moreover, the larger the difference in masses of the constituent particles, the larger the cutoff Λ. To easily compare the results of different theoretical models, we list the results of some models and our results in Table 1.$ K\bar{K} $ 

$ DK $ 

$ \bar{B}K $ 

$ D\bar{D} $ 

$ B\bar{B} $ 

$ BD $ 

$ \bar{K}\bar{K} $ 

$ D\bar{K} $ 

$ \bar{B}\bar{K} $ 

$ DD $ 

$ \bar{B}\bar{B} $ 

$ \bar{B}D $ 

I 0 1 0 1 0 1 0 1 0 1 0 1 0 1 0 1 0 1 0 1 0 1 0 1 qBSE [24, 38] $ - $ 

$ - $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ - $ 

$ - $ 

HGS [60, 64] $ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ \surd $ 

$ \times $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ \times $ 

$ \times $ 

$ \surd $ 

$ \times $ 

OBE [58, 62] $ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \surd $ 

$ \times $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \surd $ 

$ \surd $ 

$ \surd $ 

CCS [37, 65] $ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ \times $ 

$ \surd $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \surd $ 

$ - $ 

$ - $ 

QM [36] $ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

$ - $ 

NRS [26] $ \times $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \surd $ 

$ \times $ 

Our results $ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \surd $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \times $ 

$ \surd $ 

$ \times $ 

Table 1. The results for different theoretical models.
$ \surd $ and$ \times $ indicate whether the corresponding system is a bound state or not, respectively.$ - $ indicates the corresponding system without any study.In Ref. [45], the authors systematically studied the interaction of
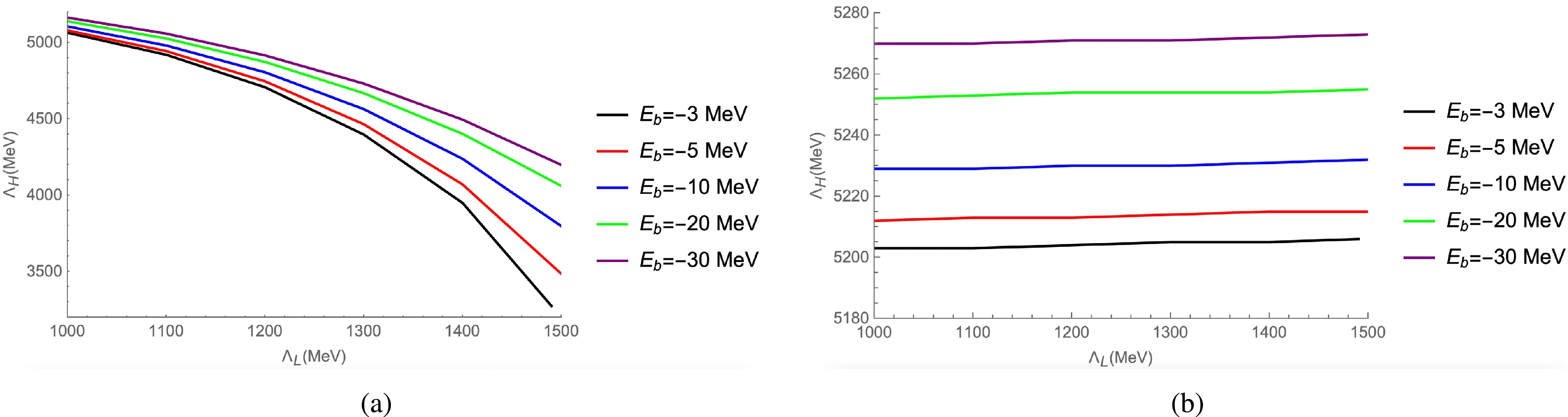
$ D\bar{D}^\ast $ in the isospin$ I=0 $ channel. In their work, it is shown that the exchange of a light$ q\bar{q} $ is OZI forbidden in the$ I=1 $ channel. As a consequence, only the$ J/\psi $ exchange is allowed in the case of$ I=1 $ , and the simultaneous two pion exchange, which was evaluated in Ref. [45,44], was found to be weaker than the exchange of the vector meson. In spite of the large mass of the$ J/\psi $ , which suppresses the propagator of the exchanged$ J/\psi $ , it was found in Refs. [45,44] that the interaction could bind the$ D\bar{D}^\ast $ and$ D^\ast\bar{D}^\ast $ systems with$ I=1 $ weakly. Subsequently, in Ref. [66], it was also found that the bound state$ D\bar{D}^\ast $ with$ I^G(J^P)=1^+(1^+) $ would disappear if the$ J/\psi $ exchange was removed, which means that the$ J/\psi $ exchange is important to provide an attractive interaction and produce the pole in the isovector system. In the present work, we also consider the effects of exchanged heavy mesons. Considering the differences in the wave functions and masses of the light and heavy mesons, and ensuring a positive from factor, we choose different magnitudes of cutoffs$ \Lambda_L $ and$ \Lambda_H $ for the exchanged light and heavy mesons, respectively.We vary the cutoff
$ \Lambda_L $ of the exchanged light mesons in the range of 800–1500 MeV to find the cutoff$ \Lambda_H $ of the exchanged heavy mesons that can form bound states. The results for some possible bound states of$ D\bar{D} $ and$ B\bar{B} $ are presented in Figs. 2 and 3. From these results in Figs. 2 and 3, we can see that the effect of the exchange of a heavy meson cannot be ignored. As the contributions of the exchanged ρ and ω almost cancel each other out in the$ I=1 $ $D\bar{D} $ and$ B\bar{B} $ systems, the main contribution comes from the heavy meson exchange. It can be seen that the results for$ \Lambda_H $ are almost twice the mass of the exchanged heavy meson in Figs. 2(b) and 3(b). This is similar to the case of considering only the light meson exchange, in which the value of the cutoff Λ is also approximately twice the mass of the exchange meson, as in Fig. 1(a). The possibility of bound states being formed when only considering the contribution of the heavy meson exchange, along with the existence of$ \bar{D}D $ and$ B\bar{B} $ bound states with$ I=1 $ , is yet to be confirmed experimentally. -
In this study, we derived the BS equation for the S-wave
$ K\bar{K} $ ,$ DK $ ,$ {B}\bar{K} $ ,$ D\bar{D} $ ,$ B\bar{B} $ ,$ BD $ ,$ KK $ ,$ D\bar{K} $ ,$ \bar{B}\bar{K} $ ,$ DD $ ,$ \bar{B}\bar{B} $ , and$ \bar{B}D $ systems and systematically investigated the possible bound states of these systems with the ladder approximation and instantaneous approximation for the kernel. In our model, the kernel containing one-particle-exchange diagrams was induced by the light meson (ρ, ω, ϕ, and σ) and heavy meson ($ J/\psi $ and$\Upsilon $ ) exchanges. To investigate the bound states, we have numerically solved the BS equations for S-wave systems composed of two pseudoscalar mesons. The possible S-wave bound states investigated in our study are helpful in explaining the structures of experimentally discovered exotic states and predicting unobserved exotic states.We found that the
$ K\bar{K} $ ,$ DK $ ,$ \bar{B}{K} $ ,$ D\bar{D} $ ,$ B\bar{B} $ ,$ BD $ ,$ D\bar{K} $ ,$ \bar{B}\bar{K} $ , and$ \bar{B}D $ systems with$ I=0 $ can exist as bound states. The$ \bar{K}\bar{K} $ ,$ DD $ , and$ \bar{B}\bar{B} $ systems with$ I=0 $ are forbidden because of Bose symmetry, and the interactions in the$ I=1 $ systems are repulsive; hence, no bound states exist in the$ \bar{K}\bar{K} $ ,$ DD $ , and$ \bar{B}\bar{B} $ systems. We also found that, for the constituent particles with the same mass, the larger the mass of the constituent particle, the smaller the cutoff Λ; moreover, the larger the difference in the masses of the constituent particles, the larger the cutoff Λ. The contribution of the σ exchange is too small to form bound states.In the calculation, we considered the heavy meson exchanges in the kernel. We found that the effect of the heavy meson exchange cannot be neglected for the
$ D\bar{D} $ and$ B\bar{B} $ systems. Since the contributions from the ρ and ω exchanges almost cancel each other out in the$ I=1 $ $D\bar{D} $ and$ B\bar{B} $ systems, the main contribution comes from the heavy meson exchanges, and the$ I=1 $ $D\bar{D} $ and$ B\bar{B} $ systems can exist as bound states. However, since the cutoff$ \Lambda_H $ for the heavy meson exchanges is very large, the possibility of bound states being formed when only considering the contribution of the heavy meson exchange, along with the existence of$ \bar{D}D $ and$ B\bar{B} $ bound states with$ I=1 $ , is yet to be confirmed experimentally.With the restarted LHC and other experiments, more experimental studies of exotic hadrons will be performed in the near future. Recently, the LHCb collaboration observed three never-before-seen particles: a new kind of pentaquark and the first-ever pair of tetraquarks, which includes a new type of tetraquark [67]. These will help physicists better understand how quarks bind together into exotic particles. The theoretical explanation of the structures of experimentally observed exotic hadrons and the existence of possible molecular states predicted theoretically remain controversial. Therefore, more precise experimental studies of the exotic states will be needed to test the results of theoretical studies and improve theoretical models.
-
One of the authors (Z.-Y. Wang) thanks Professor Jia-Jun Wu and Dr. Rui-Cheng Li for helpful discussions and useful suggestions.
Investigating S-wave bound states composed of two pseudoscalar mesons
- Received Date: 2022-08-23
- Available Online: 2023-01-15
Abstract: In this study, we systematically investigated two-pseudoscalar meson systems with the Bethe-Salpeter equation in the ladder and instantaneous approximations. By solving the Bethe-Salpeter equation numerically with the kernel containing the one-particle exchange diagrams, we found that the






 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF



























 DownLoad:
DownLoad: