-
The creation of a state of matter known as quark-gluon plasma (QGP), which is a mixture of deconfined quarks and gluons, is believed to occur in heavy-ion collisions at ultra-relativistic energies at extremely high temperatures and/or densities. Understanding the quantum chromodynamics (QCD) phase diagram of strongly interacting matter is crucial in the field of nuclear physics. The QCD phase diagram can be represented in a two-dimensional graph of temperature (T) versus baryon chemical potential (
$ \mu_{B} $ ). According to lattice QCD calculations, the transition from the hadronic phase to QGP occurs as a smooth crossover at low values of$ \mu_{B} $ [1, 2]. However, at finite values of$ \mu_{B} $ , it is predicted to be a first-order phase transition based on QCD model calculations [3−6]. If these predictions hold true, there must be a QCD critical point, marking the endpoint of the first-order phase boundary. Despite ongoing theoretical discussions on the location and even the existence of the QCD critical point, relativistic heavy-ion collisions provide a controlled means to explore the QCD phase structure and search for the critical point [7−17].As the system approaches the QCD critical point, the correlation length and density fluctuations increase. The fluctuations of conserved quantities, such as net-baryon, net-charge, and net-strangeness, are acutely influenced by the correlation length. The STAR experiment conducted measurements of the high-order cumulants and second-order off-diagonal cumulants of net-proton, net-charge, and net-kaon multiplicity distributions [12, 14, 15, 18] in Au+Au collisions across a broad range of energies, from as low as
$ \sqrt{s_{NN}} = $ 7.7 GeV up to 200 GeV. In the most central collisions, a non-monotonic behavior of the fourth-order net-proton cumulant ratio was observed, with a minimum around 19.6 GeV. The non-monotonic behavior observed in the fourth-order net-proton cumulant ratio in the STAR experiment cannot be explained by existing model calculations unless the physics of the QCD critical point is considered.Moreover, as the correlation length increases and instability spinodal domains form, critical fluctuations and first-order phase transitions can give rise to significant baryon density fluctuations. It is predicted that the production of light nuclei is sensitive to baryon density fluctuations and can thus be used to probe the QCD phase transition in heavy-ion collisions [19−24]. For instance, the light nuclei yield ratio
$ N_pN_t/N_d^2 $ of a produced proton (p), deuteron (d), and triton (t) can be described by the relative neutron density fluctuation$ \langle (\delta n)^2\rangle /\langle n\rangle^2 $ (also denoted as$ \Delta n $ in Ref. [19]). Interestingly, the yield ratio and extracted neutron density fluctuation in central Au+Au collisions measured by the STAR experiment exhibited clear non-monotonic energy dependence, with a peak at$\sqrt{s_{NN}}$ =$20-30$ GeV [25, 26].In this paper, we study the light nuclei yield ratio in Au+Au collisions at
$\sqrt{s_{NN}}$ = 7.7, 11.5, 14.5, 19.6, 27, 39, 62.4, and 200 GeV using the framework of the PYTHIA8 Angantyr model. Angantyr offers a framework wherein AA-collisions can be constructed as a superposition of binary nucleon-nucleon collisions, as implemented in the PYTHIA8 event generator. Our paper is organized as follows. In Sec. II.A, we give a brief introduction to the PYTHIA8 Angantyr model. In Sec. II.B, the relationship between the relative neutron density fluctuation and the light nuclei yield ratio in heavy ion collisions is given. In Sec. III, we present the behavior of neutron density fluctuation and neutron-proton correlation (α). Finally, a summary is given in Sec. IV. -
PYTHIA [27] is an event generator that is extensively and successfully used for the study of proton-proton and proton-lepton collisions. In pp collisions, a multi-parton interaction (MPI) is generated under the assumption that every partonic interaction is almost independent. PYTHIA8 does not natively support heavy-ion systems. Recently, the PYTHIA8 Angantyr model [28] extrapolated pp dynamics into heavy-ion collisions using the PYTHIA8 event generator, enabling the study of heavy nuclei collisions, namely, proton-nuclei (pA) and nuclei-nuclei (AA) collisions. The Angantyr model combines several nucleon-nucleon collisions into one heavy-ion collision. It is a combination of many-body physics (theoretical) models suitable for producing hard and soft interactions, initial and final-state parton showers, particle fragmentations, multi-partonic interactions, color reconnection (CR) mechanisms, and decay topologies. However, it does not include any mechanism of the QGP medium believed to be produced in AA collisions.
In the current version of the PYTHIA8 Angantyr model [29], in a heavy-ion collision, each projectile nucleon can interact with several target nucleons, and the number of participant nucleons is determined by the Glauber model. This model incorporates several algorithms to distinguish different types of nucleon-nucleon interactions, such as elastic, diffractive, and absorptive interactions, and its purpose is to effectively describe final-state properties, such as multiplicity and transverse momentum distributions in AA collisions [30, 31].
We use the 8.308 version of PYTHIA8 in this study. A simulation is considered with different PYTHIA tunes using MPI and CR configurations. Approximately one million events are generated for each collision energy in the Au + Au collisions. In the PYTHIA8 Angantyr model, both the density and density fluctuation are dependent on system volume. To remove the system volume effect, we use two dimensionless statistical quantities,
$ \langle (\delta p)\rangle /\langle p\rangle $ and$ \langle (\delta n)\rangle /\langle n\rangle $ (Sec. II.B). The nucleons are extracted for different rapidity ranges and centralities. We define the centrality intervals based on the summed transverse energy ($ \sum E_T $ ) in the pseudorapidity interval [–0.5, 0.5]. -
Based on Ref. [24, 32, 33], it has been proposed that the creation of light nuclei occurs through the process of nucleon coalescence. Via this mechanism, protons and neutrons come together to form deuterons and tritons. If we neglect the binding energy, the number of these particles can be expressed as
$ \begin{equation} N_d \approx \frac{3}{2^{1/2}}\left(\frac{2\pi}{mT}\right)^{3/2}N_p\langle n \rangle(1+\alpha), \end{equation} $

(1) $ \begin{equation} N_t \approx \frac{3^{3/2}}{4}\left(\frac{2\pi}{mT}\right)^{3}N_p\langle n \rangle^2(1+\Delta n+2\alpha), \end{equation} $

(2) and then, to eliminate the energy dependence on the local effective temperature (T) at coalescence, we have the light nuclei ratio
$ \begin{equation} \frac{N_tN_p}{N_d^2}=g\frac{1+\Delta n+2\alpha}{(1+\alpha)^2}, \end{equation} $

(3) where
$ N_p $ ,$ N_d $ , and$ N_t $ represent the number of protons, neutrons, and tritons, respectively, m denotes the mass of a nucleon, and$ \Delta n = \langle (\delta n)^2\rangle /\langle n\rangle^2 $ is the dimensionless relative neutron density fluctuation. The standard deviation and mean value of neutron production are represented by$ \langle (\delta n) \rangle $ and$ \langle n\rangle $ , respectively, in our paper.$ \alpha= \langle\delta n \delta p\rangle/ (\langle n\rangle\langle p\rangle) $ is the neutron and proton number density correlation coefficient, and g =$\dfrac{3^{3/2}}{4} \Big/\left(\dfrac{3}{2^{1/2}}\right)^{2}$ =$ \dfrac{1}{2\sqrt{3}} $ .If we assume that the correlation coefficient between the neutron and proton density is small, α can be approximated as zero (
$ \alpha\approx0 $ ), and Eq. (3) can be rewritten as$ \begin{equation} \frac{N_tN_p}{N_d^2}=\frac{1+\Delta n}{2\sqrt{3}}. \end{equation} $

(4) -
Except for the physical parameters used in the model calculations mentioned in last paragraph of Sec. II.A, we have the option to incorporate the MPI based CR mechanism by switching on/off the ColourReconnection: reconnect and PartonLevel: MPI.
The results in Figs. 1, 2, 3, 4, and 6 are analyzed using the PYTHIA8 Angantyr model with the MPI based CR mechanism.
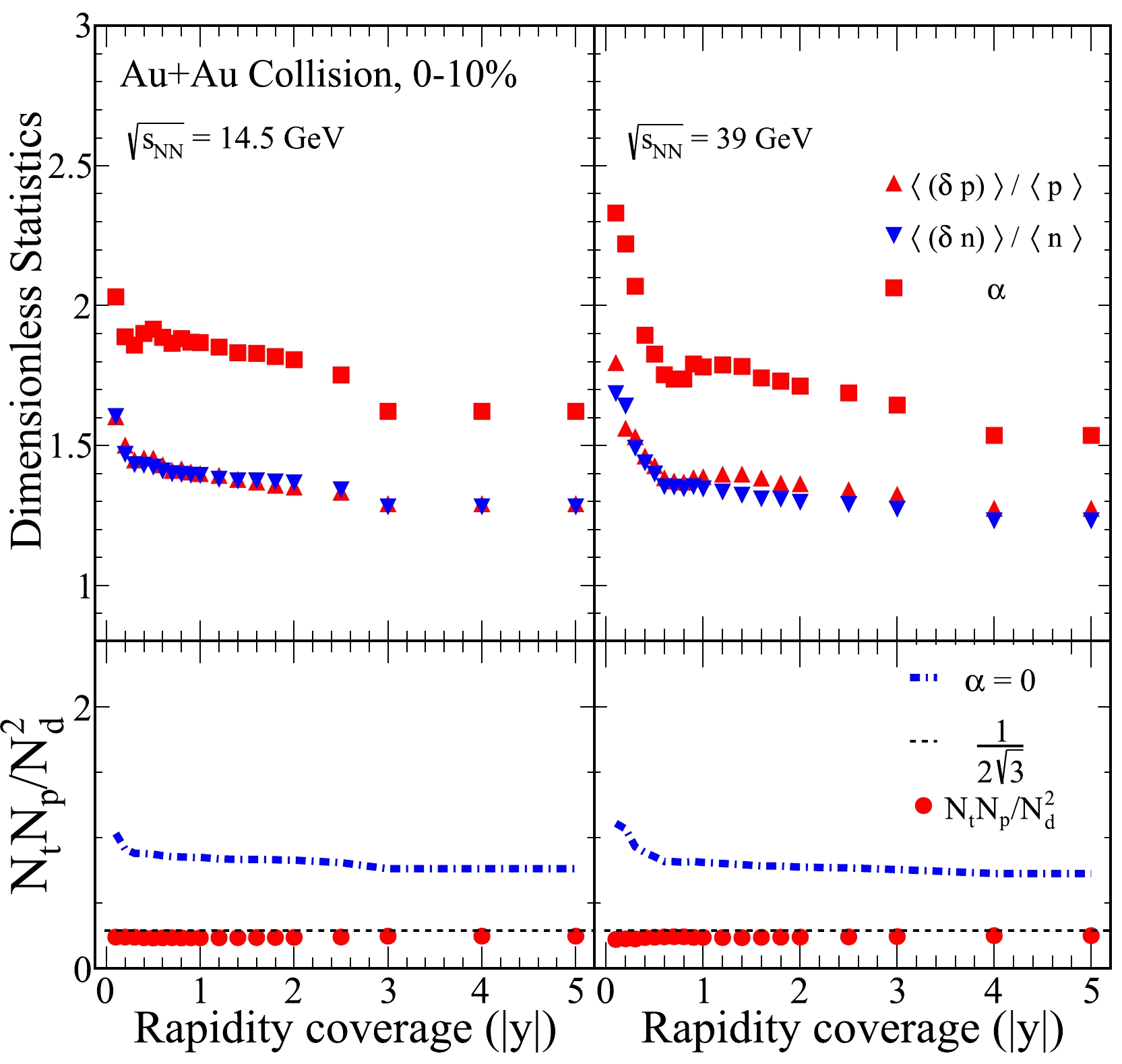
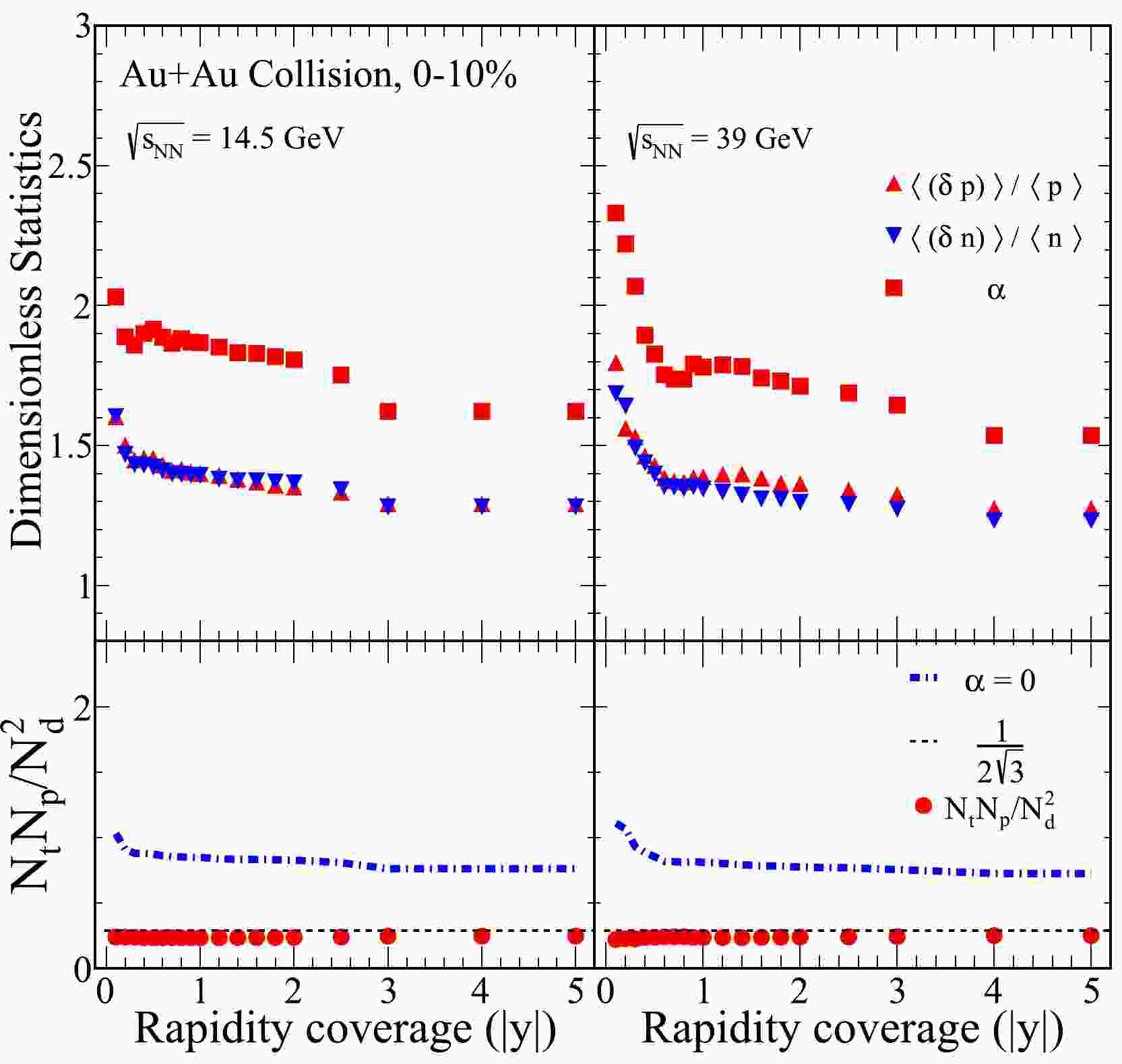
Figure 1. (color online) Top panels show various dimensionless statistics, including
$ \langle (\delta p) \rangle /\langle p \rangle $ ,$ \langle (\delta n) \rangle /\langle n \rangle $ , and α, which are associated with 0-10% Au+Au collisions at center-of-mass energies of$\sqrt{s_{NN}}=$ 14.5 and 39 GeV. Meanwhile, the bottom panels depict the ratio of yields for light nuclei,$ N_tN_p/N_d^2 $ , which is calculated using data from the top panels, represented as solid circles in accordance with Eq. (3) and as a dash-dot line in accordance with Eq. (4).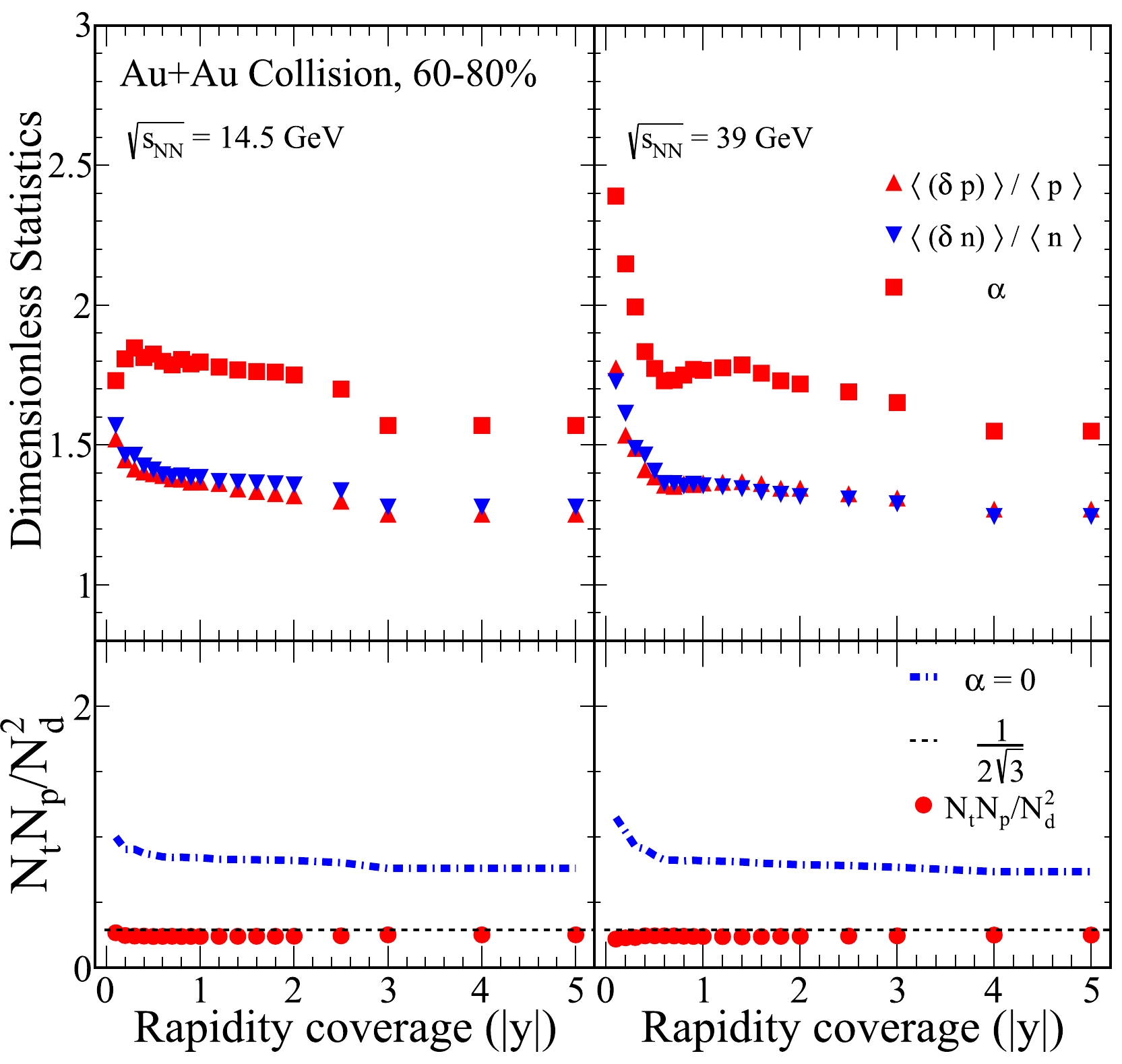
Figure 2. (color online) Top panels show various dimensionless statistics, including
$ \langle (\delta p)\rangle /\langle p\rangle $ ,$ \langle (\delta n)\rangle /\langle n\rangle $ , and α, which are associated with 60%−80% Au+Au collisions at center-of-mass energies of$\sqrt{s_{NN}}=$ 14.5 and 39 GeV. Meanwhile, the bottom panels depict the ratio of yields for light nuclei,$ N_tN_p/N_d^2 $ , which is calculated using data from the top panels, represented as solid circles in accordance with Eq. (3) and as a dash-dot line in accordance with Eq. (4).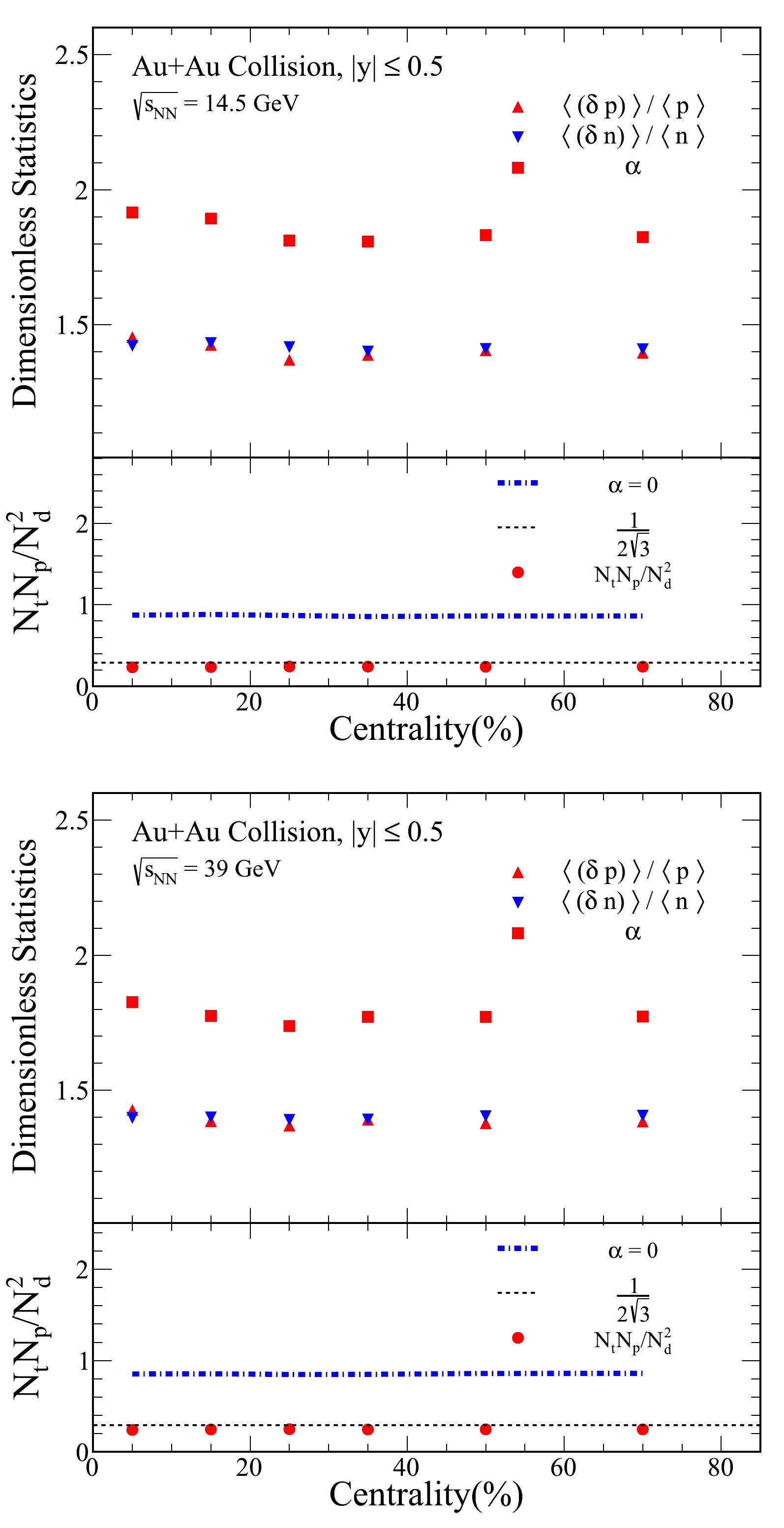
Figure 3. (color online) Dimensionless statistics
$ \langle (\delta p)\rangle /\langle p\rangle $ ,$ \langle (\delta n)\rangle /\langle n\rangle $ , and α for Au+Au collisions at center-of-mass energies of$\sqrt{s_{NN}}=$ 14.5 and 39 GeV, with rapidities confined to the range$ |y|\leq 0.5 $ . In addition, the ratio of yields for light nuclei,$ N_tN_p/N_d^2 $ , is shown, represented as solid circles in accordance with Eq. (3) and as a dash-dot line in accordance with Eq. (4).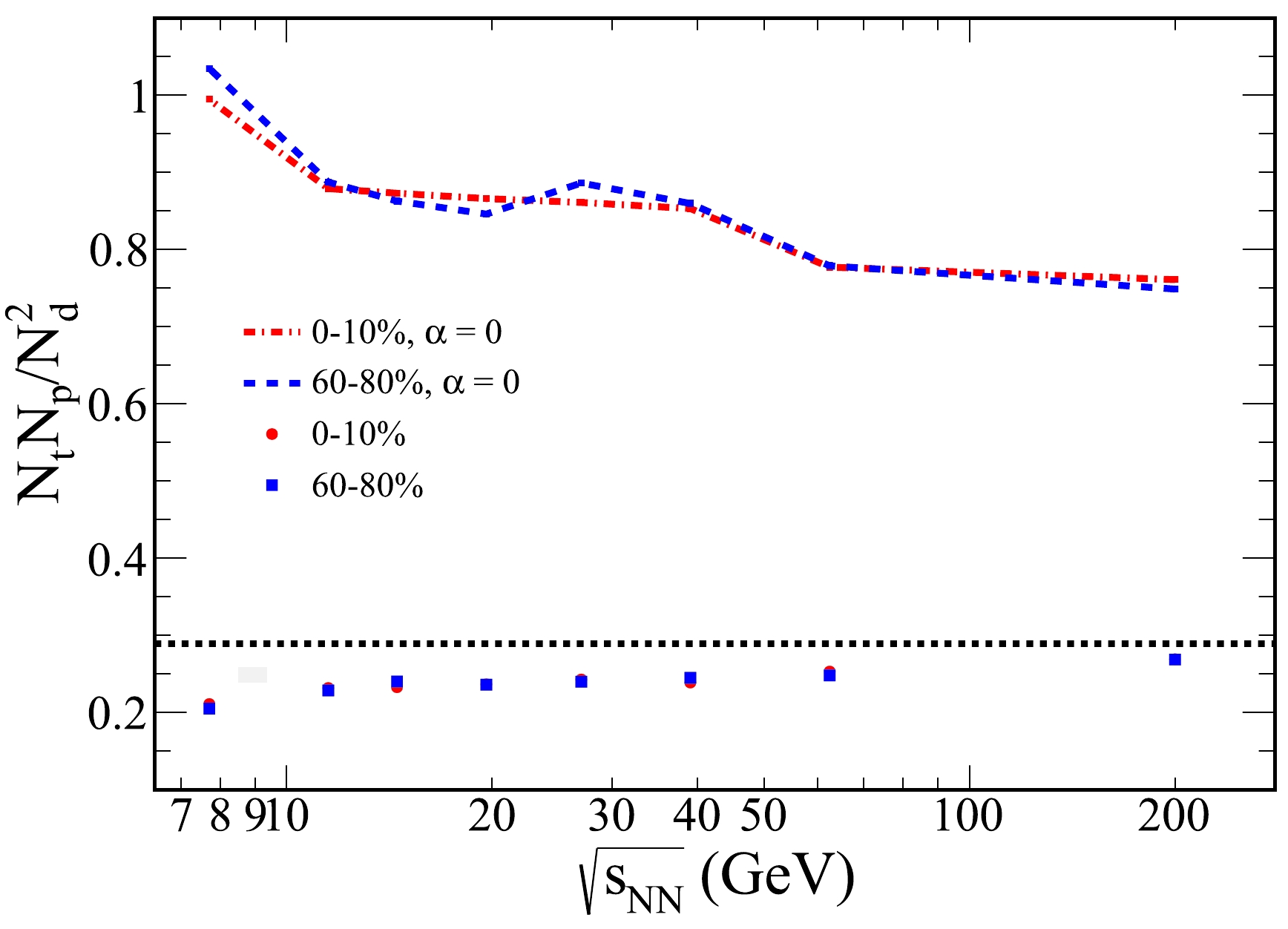
Figure 4. (color online) PYTHIA8 Angantyr model used to examine the collision energy dependence of the light nuclei yield ratio
$ N_tN_p/N_d^2 $ in Au+Au collisions with$ |y|\leq 0.5 $ . The results reveal solid circles and squares for 0−10% central and 60−80% peripheral collisions, respectively. Additionally, the dash-dot lines represent the corresponding results with vanished α.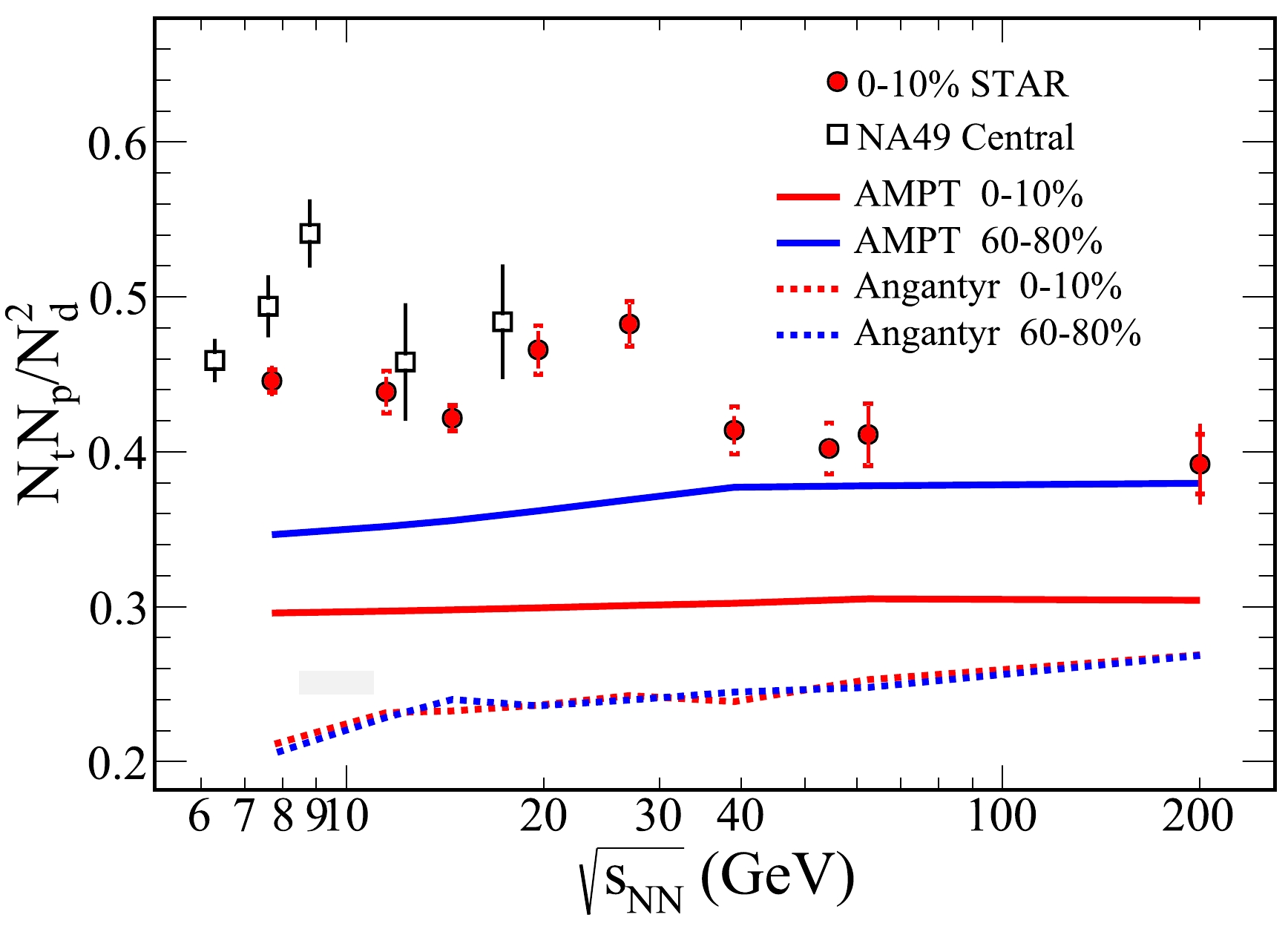
Figure 6. (color online) Yield ratio of
$ N_tN_p/N_d^2 $ from the PYTHIA8 Angantyr model, which considers the collision energy and centrality, is analyzed in the context of$ |y|\leq 0.5 $ , represented as dash lines from the PYTHIA8 Angantyr model and as solid lines from the AMPT model [33]. The PYTHIA8 Angantyr model is under the MPI and RC mode. The solid circles are the results from STAR detector at 0−10% central Au+Au collisions [26, 34]. Additionally, the open squares represent the findings from NA49 in central Pb+Pb collisions [24, 35].The top panels of Fig. 1 illustrate the rapidity dependence of the dimensionless statistics
$ \langle (\delta p)\rangle /\langle p\rangle $ and$ \langle (\delta n)\rangle /\langle n\rangle $ for 0−10$ \% $ Au+Au collisions at$\sqrt{s_{NN}}=$ 14.5 and 39 GeV. As the rapidity coverage is increased, the relative fluctuations in nucleon density ($ \langle (\delta p)\rangle /\langle p\rangle $ and$ \langle (\delta n)\rangle /\langle n\rangle $ ) displayed in the top panels of Fig. 1 appear to decrease. As the rapidity coverage is expanded, the nucleon density fluctuations appear to converge toward a constant value.$ \langle (\delta p)\rangle /\langle p\rangle $ and$ \langle (\delta n)\rangle /\langle n\rangle $ are consistent in Fig. 1. The correlation α has a similar trend to relative fluctuations in nucleon density with rapidity coverage for 0−10$ \% $ Au+Au collisions at both$\sqrt{s_{NN}}=$ 14.5 and 39 GeV. We plot the line of$ 1/2\sqrt{3} $ , which indicates that both density fluctuation and correlation disappear. From Eq. (3), the light nuclei ratio$ N_tN_p/N_d^2 $ can be calculated. The rapidity dependence of this ratio, represented by solid circles, is shown in the bottom panel of Fig. 1. The ratio for 0−10$ \% $ Au+Au collisions at both$\sqrt{s_{NN}}=$ 14.5 and 39 GeV is lower than the line of$ 1/2\sqrt{3} $ with rapidity distribution. However, the light nuclei ratio with the dash-dot lines ($ \alpha\approx0 $ ) is higher than the line of$ 1/2\sqrt{3} $ . Therefore, α cannot be neglected in the central collision to calculate the relative neutron density fluctuation from the light nuclei yield ratio using Eq. (4). Results from other collision energies at central collision are similar.The rapidity dependence of
$ \langle (\delta p)\rangle /\langle p\rangle $ and$ \langle (\delta n)\rangle /\langle n\rangle $ for 60%−80$ \% $ Au+Au collisions at$\sqrt{s_{NN}}=$ 14.5 and 39 GeV and the related light nuclei ratio are shown in the top and bottom panels of Fig. 2, respectively. Similar to the results of the central collisions,$ \langle (\delta p)\rangle /\langle p\rangle $ and$ \langle (\delta n)\rangle /\langle n\rangle $ decrease with increasing rapidity coverage. The correlation α is dependent on rapidity coverage and has a similar trend to relative fluctuations in nucleon density. We present the light nuclei yield ratios, calculated using Eqs. (3) and (4), in the bottom sections of Fig. 2. Our results reveal that the exclusion of the α parameter leads to a significant increase in the light nuclei yield ratio. In other words, the omission of α results in a smaller relative neutron density fluctuation with the light nuclei yield ratio.The top panels of Fig. 3 show the centrality dependent
$ \langle (\delta p)\rangle /\langle p\rangle $ and$ \langle (\delta n)\rangle /\langle n\rangle $ for Au+Au collisions at$\sqrt{s_{NN}}=$ 14.5 and 39 GeV with rapidity coverage$ |y|\leq $ 0.5. Both quantities are flat from central to peripheral collisions. At the bottom of Fig. 3, we find that the two ratios given by Eqs. (3) and (4) are flat at central, mid-central, and peripheral collisions. Note that the relative nucleon density fluctuation cannot be extracted directly from the light nuclei ratio, and the effects of the neutron-proton correlation α must be considered in different collision centralities.The collision energy dependence of
$ N_tN_p/N_d^2 $ from 0−10% central to 60%−80% peripheral Au+Au collisions with$ |y|\leq 0.5 $ is shown in Fig. 4. It is clear from Fig. 4 that the light nuclei yield ratio increases slightly with increasing collision energy from the PYTHIA8 Angantyr model. The light nuclei yield ratio of peripheral collisions is similar to that of central collisions, and both results are lower than$ 1/2\sqrt{3} $ . The results of vanished α decrease with increasing collision energy, which are shown as dash-dot lines. In Au+Au collisions, both central (0−10%) and peripheral (60%−80%) collisions, the yield ratios of light nuclei are not highly consistent with each other after the disappearance of α.To observe the effect of different PYTHIA tunes, we consider the following configurations: MPI with CR, No CR, No MPI, and both MPI and CR off. Figure 5 shows the collision energy dependence of the light nuclei yield ratio
$ N_tN_p/N_d^2 $ from 0−10% central and 60%−80% peripheral Au+Au collisions with$ |y|\leq 0.5 $ in different PYTHIA8 Angantyr model tunes. It is also clear from this figure that the light nuclei yield ratio increases slightly with increasing collision energy from the PYTHIA8 Angantyr model. However, the results of vanished α decreases with increasing collision energy, which are shown as dash-dot lines. For Au+Au collisions, in different PYTHIA8 Angantyr model tunes, there is no effect of CR if the MPI is off.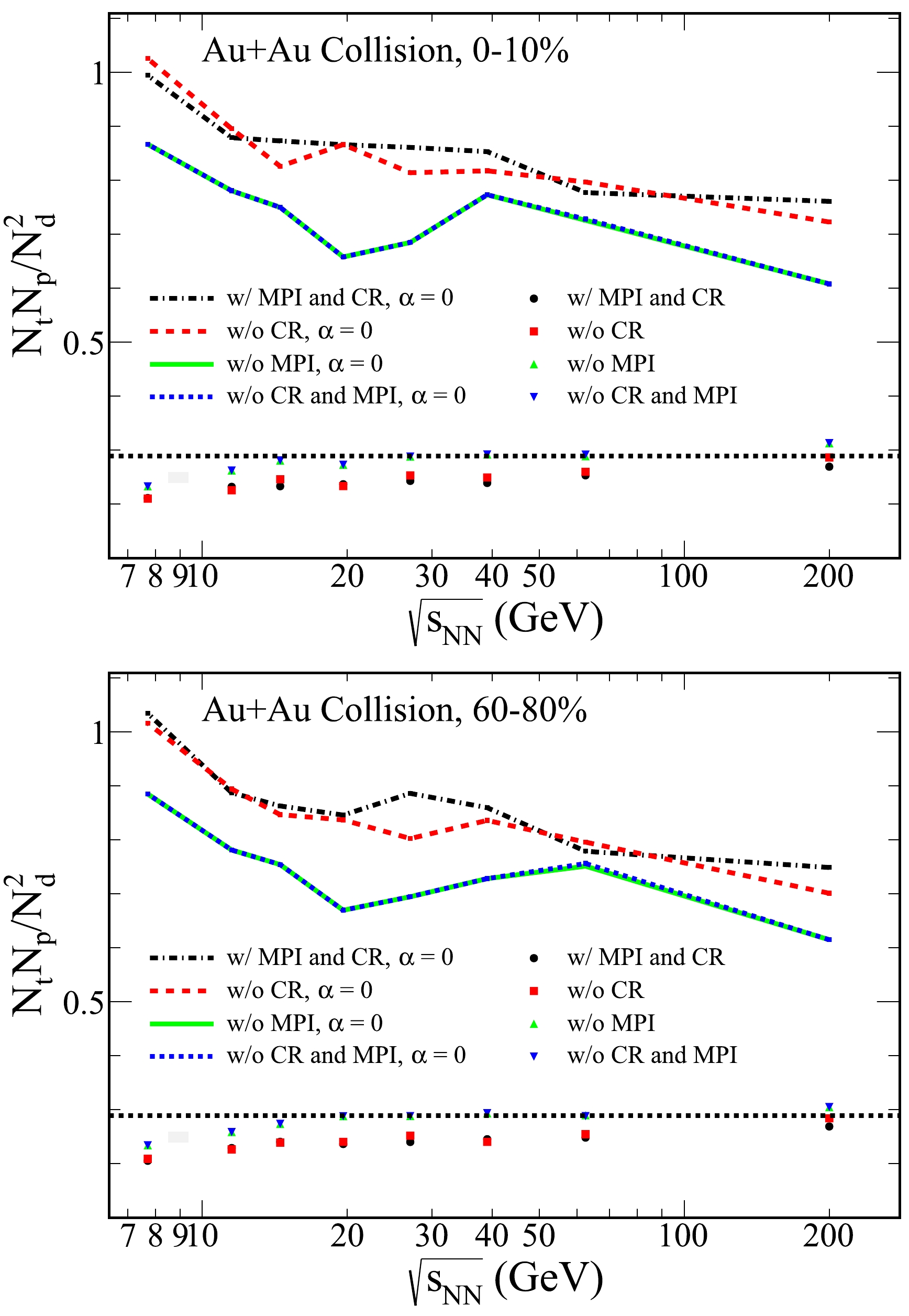
Figure 5. (color online) PYTHIA8 Angantyr model used to examine the collision energy dependence of the light nuclei yield ratio
$ N_tN_p/N_d^2 $ in Au+Au collisions with$ |y|\leq 0.5 $ in different PYTHIA8 Angantyr model tunes. The results shown in the upper and lower panels are related to 0−10% central and 60%−80% peripheral collisions, respectively.Figure 6 presents the experimental results of the STAR detector at 0−10% central Au+Au collisions [26, 34] and NA49 at central Pb+Pb collisions [24, 35], which are then compared with results from the AMPT and PYTHIA8 Angantyr models. From the experimental results, it is evident that there is a non-monotonic energy dependence. The yield ratio of light nuclei peaks at
$\sqrt{s_{ NN}}= 20 - 30$ GeV, which implies the most significant relative neutron density fluctuations in this energy range. Both the AMPT and PYTHIA8 Angantyr model results are lower than the experimental results. Moreover, the AMPT model results, which include QGP medium mechanisms and do not consider critical physics, are higher than the PYTHIA8 Angantyr model results. Owing to the absence of critical physics and QGP medium mechanisms, the PYTHIA8 Angantyr model is unable to describe this non-monotonic energy dependence. -
We employed the PYTHIA8 Angantyr model to explore the dependence of the relative neutron density fluctuation, neutron-proton correlation α, and corresponding light nuclei yield ratio
$ N_{t}N_{p}/N_{d}^2 $ on rapidity, centrality, and collision energy. Using the PYTHIA8 Angantyr model, the relative nucleon density fluctuation cannot be extracted directly from the light nuclei ratio, and the effects of the neutron-proton correlation α must be considered in different collision centralities.$ N_tN_p/N_d^2 $ does not change with increasing rapidity coverage and collision centrality; however, it increases slightly with increasing collision energy from the PYTHIA8 Angantyr model. We present the interplay between the MPI and CR on the light nuclei yield ratio and find that there is no effect of CR if the MPI is off. The experimental results reveal that the light nuclei yield ratio exhibits a peak at$\sqrt{s_{NN}}=20 - 30$ GeV, indicating a significant fluctuation in relative neutron density. The non-monotonic energy dependence measurements are underestimated by the PYTHIA8 Angantyr model owing to the lack of critical physics and QGP medium mechanisms. Nevertheless, it can be utilized as a baseline in scenarios where critical physics and QGP medium mechanisms are absent.
Study of neutron density fluctuation and neutron-proton correlation in Au+Au collisions using PYTHIA8/Angantyr
- Received Date: 2023-05-04
- Available Online: 2023-11-15
Abstract: Utilizing the PYTHIA8 Angantyr model, which incorporates the multiple-parton interaction (MPI) based color reconnection (CR) mechanism, we study the relative neutron density fluctuation and neutron-proton correlation in Au+Au collisions at





 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF
















 DownLoad:
DownLoad: