-
Since the prediction of the superheavy island in 1960s based on an independent particle model [1, 2], the synthesis and properties of superheavy nuclei (SHN) have drawn significant interest theoretically and experimentally in modern nuclear physics. To date, SHN with atomic number
$Z = 104-118$ have been successfully synthesized in laboratories with cold-fusion reactions involving lead and bismuth targets irradiated by medium-mass projectiles as well as with hot-fusion reactions involving actinide targets irradiated by$ ^{48} $ Ca projectile [3–8]. All observed SHN are inherently unstable, with α-decay being the most important decay mode. In experiments, α-decay is essential for identifying new elements or new nucleus by observing α-decay chain from an unknown parent nucleus to a known nuclide. On the theoretical side, α-decay is understood as the tunneling of an α-particle through a potential barrier between an α particle and a daughter nucleus [9, 10], and a full understanding of α-decay mechanism involves how α-particle forms in the parent nucleus [11, 12]. Consequently, considerable attention has been devoted to theoretical calculations of α-decay of SHN using various models which serve experimental design and identification.The two most important α-decay properties of SHN that can be measured experimentally are decay energy
$ Q_{\alpha} $ and half-life. Furthermore,$ Q_{\alpha} $ value is particularly essential for estimating α-decay half-life. The half-life is highly sensitive to$ Q_{\alpha} $ value such that an uncertainty of 1 MeV in$ Q_{\alpha} $ corresponds to an uncertainty of α-decay half-life ranging from$ 10^3 $ to$ 10^5 $ times in heavy nuclei region [13]. The most remarkable structural feature of superheavy nuclei (SHN) is the location of shell closures, or magic numbers. Given the scarcity of observed physical data on SHN, uncovering their underlying structural properties—particularly shell stabilization—requires leveraging the valuable information provided by measured α-decay energies and half-lives. Although shell effects are inherently embedded in α-decay energies, disentangling them remains a challenging task.The interplay between the Coulomb interaction among protons, which tends to deform the nucleus and surface energy, which favors a spherical shape, results in the emergence of a potential barrier that resists nuclear fission when the proton number Z is below 103 according to the liquid drop model. For nuclei with
$ Z>103 $ , the fission barrier predicted by the liquid drop model almost vanishes, rendering the existence of SHN untenable due to prompt fission within this framework. However, calculations based on independent-particle shell models have indicated that the shell effects arising from the quantum motion of nucleons inside the nucleus strongly enhance nuclear binding on SHN [1, 14, 15]. Early theoretical calculations predicted SHN with proton number$ Z=114 $ and neutron number$ N=184 $ as the center of the 'island of stability' of SHN [15]. This implies that the doubly-magic spherical nucleus beyond$ ^{208} $ Pb is predicted as$ ^{298} $ Fl, and the SHN located at or around this center is expected to be long-lived with lifetimes ranging from minutes to millions of years.Subsequently, many theoretical approaches have been employed to predict the spherical magic nuclei in superheavy mass region. However, the results are generally model-dependent due to insufficient knowledge of the effective nuclear force and difficulty of nuclear many-body techniques. For instance, the macroscopic-microscopic models with various parameterizations predict the spherical shell closures at
$ Z=114 $ and$ N=184 $ [16, 17]. The non-relativistic energy density functional with Skyrme effective nucleon-nucleon interactions favor$ Z=124 $ , 126 and$ N=184 $ [18, 19]. As two types of relativistic energy density functional, the relativistic mean-field model usually favors$ Z=120 $ ,$ N=172 $ [19–21] while the relativistic-Hartree-Fock leads to$ Z=120 $ and$ N=184 $ [22]. Hence, the precise location of the spherical shells in superheavy mass region remains an open question. There is still a long way to go to reach these predicted doubly-magic SHN in experiment.Nevertheless, some deformed shells have received significant interest and achieved important progress experimentally and theoretically. The existence of a "shallow" of SHN has been suggested both experimentally and theoretically, which is expected to include the deformed SHN and is expected to be centered on
$ Z=108 $ and$ N=162 $ [23–28]. Lazarev et al. discussed the enhanced nuclear stability near$ Z=108 $ and$ N=162 $ by assigning α-decay to even-even daughter nucleus$ ^{266} $ Sg [29]. Later, experimental measurements clearly showed the doubly-magic nature of$ ^{270} $ Hs [30], which is the only deformed doubly-magic SHN produced to date [28, 31–33]. Additionally, by extending the systematics of the one-quasiparticle energies in$ N =151 $ nuclei into those in$ ^{245} $ Pu, the shell gap of$ N=152 $ is reduced in energy with decreasing proton number [34]. The study of$ ^{250} $ Fm high-spin state along with the comparison with the known two-quasiparticle structure of$ ^{254,252} $ No, supports the existence of deformed shell gaps at$ N=152 $ [35]. The direct measurement of nuclear binding energies for nobelium and lawrencium isotopes pin down the deformed$ N=152 $ shell gap in$ Z=102,103 $ isotopes, and these results are claimed to be highly relevant for improving predictions of 'island of stability' [36].On the theoretical side, the deformed doubly-magic nature of
$ ^{270} $ Hs ($ Z=108 $ ,$ N=162 $ ) have been predicted by macroscopic-microscopic models and energy density functional approaches [26–28, 31, 33]. Its basic properties, such as binding energy and moments of inertia, are obviously affected by higher-order deformations [37–40]. Recently, the multidimensionally-constrained relativistic mean-field model with PC-PK1 effective interaction [41] was used to study$ ^{270} $ Hs, and large shell gaps were found to exhibit at$ Z=108 $ and$ N=162 $ in single-particle levels. Interestingly, it is concluded that higher-order deformations, such as$ \beta_6 $ , have significant influence on the binding energy and shell gaps of$ ^{270} $ Hs [38]. The macroscopic-microscopic model has predicted$ N = 152 $ as a neutron shell [42]. By examining the behavior of neutron number variation of α-decay half-life, the neutron magic numbers$ N=152 $ was suggested [43]. However, current modern self-consistent theories cannot effectively reproduce the locations of this deformed neutron shell.The testing of the robustness of the shell closures in heavy nuclei region is of significant interest [44, 45], and it is even more intriguing in superheavy nuclei region. For instance,
$ N=152 $ neutron shell has been confirmed experimentally in$ ^{254} $ No and$ ^{255} $ Lr [36]. However, it remains unclear whether$ N = 152 $ is still a magic number for other isotones. Hence, we focus on$ N=152 $ neutron shell and$ Z=108 $ proton shell in the current study, aiming to explore their robustness in heavy or superheavy nuclei region via analysis of α-decay energy. Given that a parent nucleus and its daughter are of the same odevity of proton and neutron numbers, some structural effects, such as pairing correlation, can be canceled to a large extent if the shell is not crossed during α-decay. Therefore, α-decay energy can serve as an excellent physical quantity to probe the shell closures of SHN. Given the absence of sufficient data on α-decay energies for$ N=162 $ isotones (to date, there is only one experimental data), we do not discuss$ N=162 $ shell.Firstly, we examine the systematic behavior of the experimental α-decay energies
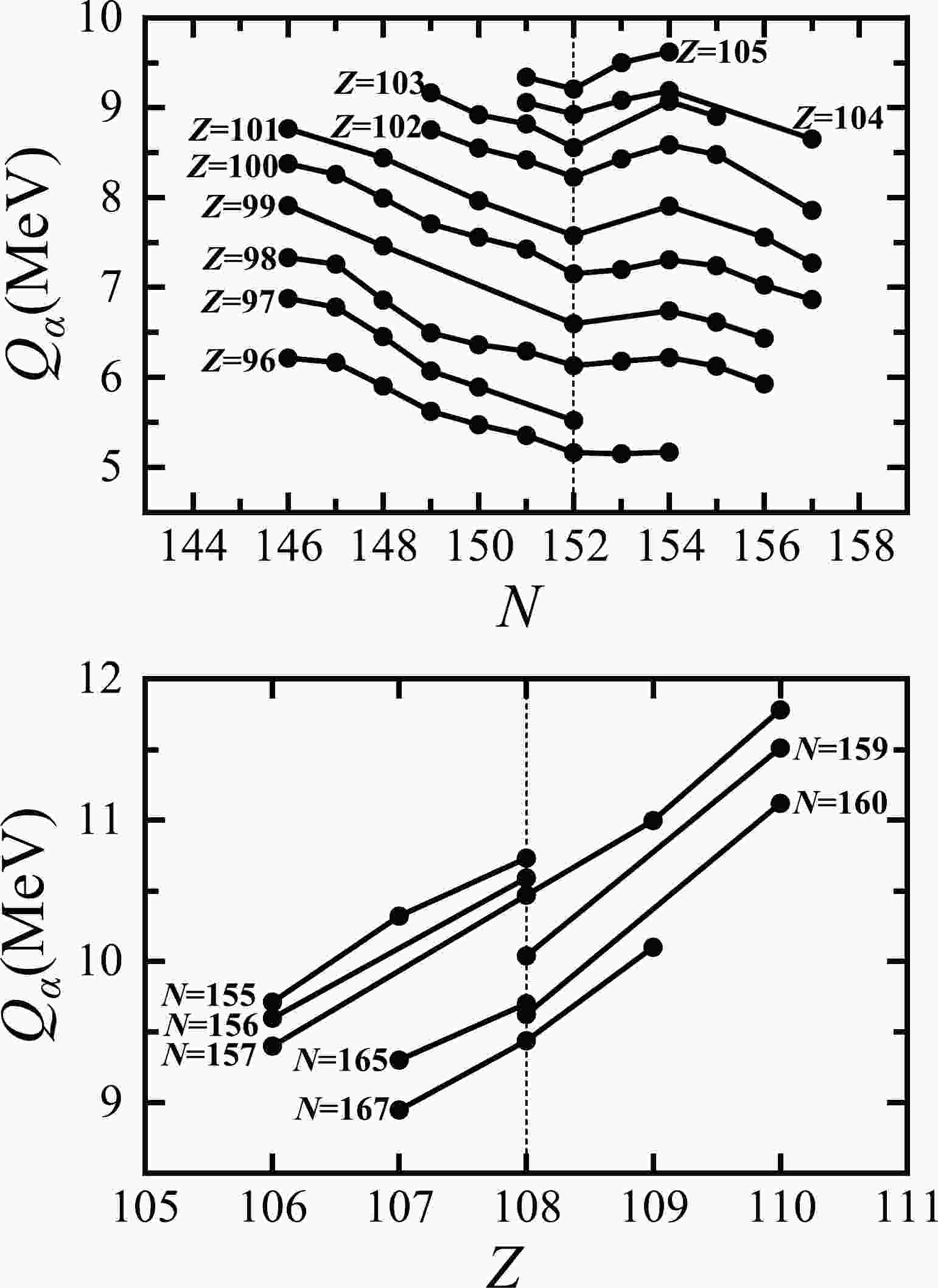
$ Q_{\alpha} $ . The experimental$ Q_{\alpha} $ values of SHN belonging to$ Z=96-105 $ isotopic chains versus neutron number N are displayed in Fig. 1(a), while the experimental$ Q_{\alpha} $ values of SHN belonging to$ N=155-167 $ isotonic chains versus proton number Z are displayed in Fig. 1(b), with all experimental data from Ref. [46]. For a given isotopic chain, the$ Q_{\alpha} $ value gradually decreases on the whole as the neutron number increases, mainly due to the influence of symmetry energy [47] that contributes negatively to$ Q_{\alpha} $ value [48]. For$ Z=102 $ and 103 isotopes, the experimental data of high-precision mass measurements have pinned the shell gap at$ N=152 $ with the aid of two neutron separation energy [36]. The local minimum of$ Q_{\alpha} $ values in$ Z=98-105 $ isotope chains is located at$ N=152 $ , suggesting the increased stability of isotopes at$ N=152 $ . An irregular behavior around$ N=152 $ for the α-decay energy$ Q_{\alpha} $ of$ Z=102 $ and 103 isotopes is clearly shown in Fig. 1(a), which can also serve as an indication for the presence of$ N=152 $ neutron shell. Intriguingly, such an irregular behavior at$ N=152 $ also visibly appears in$ Z=101,104,105 $ isotopes. For other isotopic chains, either the irregular behavior is not as distinct as for$ Z=102,103 $ or no experimental data on$ Q_{\alpha} $ exhibits shell stabilization. Unfortunately, for a given isotonic chain, as exhibited in Fig. 1(b), the overall trend shows a persistent increase in$ Q_{\alpha} $ values with increasing neutron number, thereby concealing the irregular behavior around a proton shell. Therefore, it is not straightforward to identify proton shell effect with the$ Q_{\alpha}-Z $ relationship as shown in Fig. 1(b).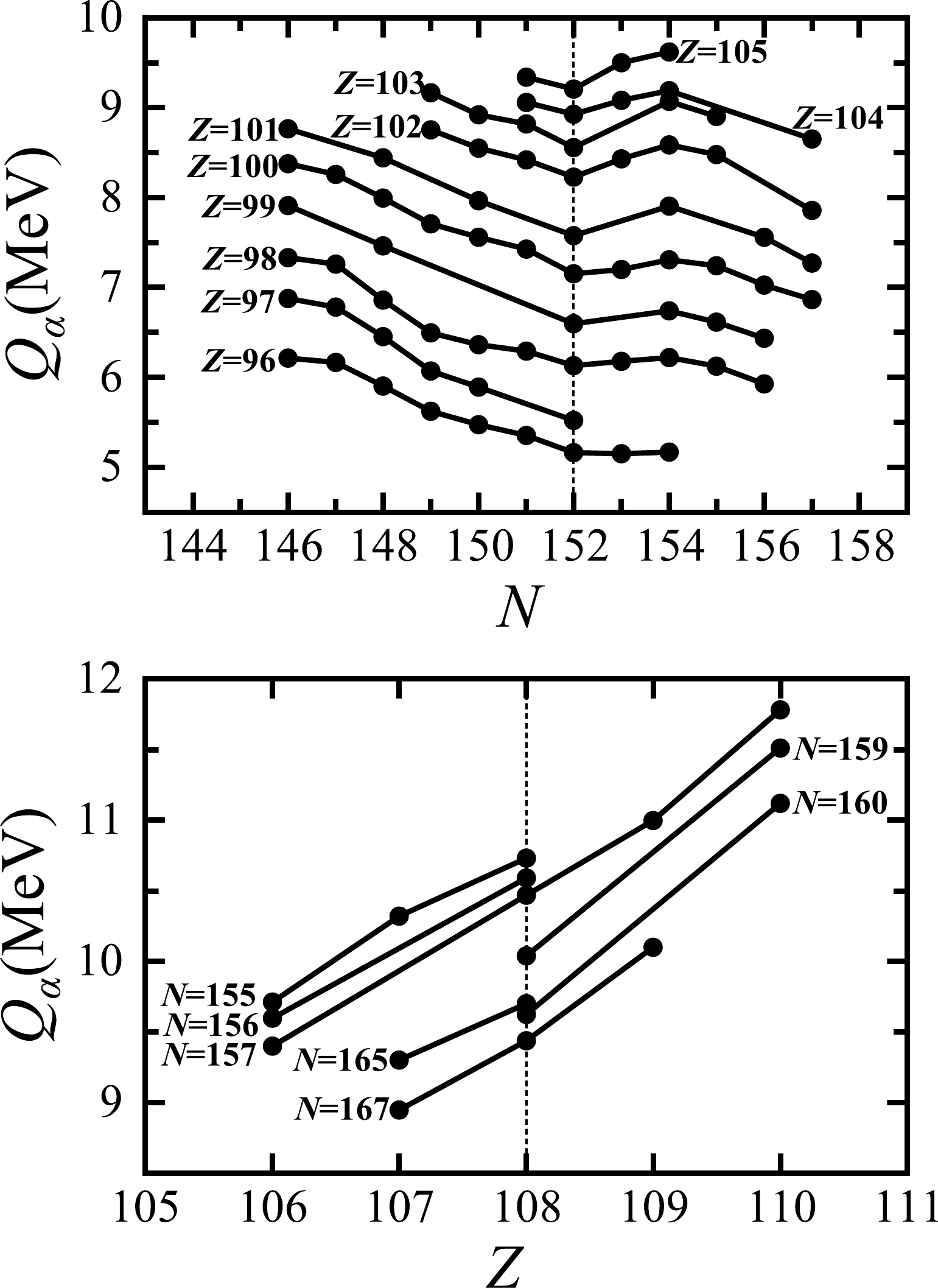
Figure 1. Experimental α-decay energy
$ Q_\alpha $ are provided for (a)$Z=96-105$ isotopic chains and (b) some$N=155-167$ isotonic chains. The experimental data are obtained from the atomic mass table of Wang et al [46].Given the drawback of
$ Q_{\alpha}-Z $ relationship shown in Fig. 1(b) for identifying the proton shell, we adopt an alternative strategy to investigate the proton shell at$ Z=108 $ . We test the stability of neutron shell with this strategy to gain a further insight into$ N=152 $ shell evolution. Dong et al. proposed a simple formula for calculating$ Q_{\alpha} $ of SHN [48]. Based on this formula, a novel method to calculate$ Q_{\alpha} $ is presented, that is, to estimate$ Q_{\alpha} $ of a SHN with the aid of its neighbors [47]. Here, we provide a concise overview without delving into the specifics of this approach. If we choose the proton number Z and isospin asymmetry$ \beta=(N-Z)/A $ as variables, then the relationship between$ Q_{\alpha} $ values of nuclei belonging to an isotopic chain with a proton number Z is provided by:$ \begin{split} Q_{\alpha 2}=&\;Q_{\alpha 1}-(\beta _{2}-\beta _{1})\Bigg[ \dfrac{2^{5/3}}{9} a_{c}Z^{2/3}(1-\beta )^{-2/3}(1+2\beta )\\&+8a_{\text{sym}}\beta \Bigg] , \end{split} $

(1) where
$ Q_{\alpha 2} $ and$ Q_{\alpha 1} $ denote α-decay energies of target nucleus and reference nucleus, respectively. The α-decay energy of the reference nucleus is obtained from the measured data in Ref. [46]. Furthermore,$ \beta_2 $ ($ \beta_1 $ ) is the isospin asymmetry of target (reference) nucleus, with$ \beta=(\beta_1+\beta_2)/2 $ . The first and second terms in the square bracket correspond to the contributions from Coulomb energy and symmetry energy, respectively. The mass$ A=N+Z $ dependence of the symmetry energy coefficient of nuclei is provided by$ a_{\text{sym}}=c_{\text{sym}}(1+\kappa A^{-1/3})^{-1} $ , where$ c_{\text{sym}} $ denotes the volume symmetry energy coefficient of the nuclei and κ denotes the ratio of the surface symmetry coefficient to the volume symmetry coefficient. Considering the presence of small uncertainties in these parameters, it is necessary to assess how these uncertainties affect the final calculated results. The values of$ a_c $ reported by different authors are consistently close to one another, ranging from 0.71 to 0.72 MeV. We choose value of$ c_{\text{sym}}= $ 31.1$ \pm $ 1.7 MeV and$ \kappa= $ 2.31$ \pm $ 0.38 from Ref. [49] to test the impact of these uncertainties, and we determined that these uncertainties result in an uncertainty of$ a_{\text{sym}} $ by approximately 2 MeV. Therefore, the uncertainty of$ Q_\alpha $ value is slightly less than 1% (0.1 MeV). Given that the error is small, we conclude that the uncertainties of these parameters have slight effect on the final results.When considering the neutron number N and isospin asymmetry β as variables, the relationship between
$ Q_{\alpha} $ of nuclei belonging to an isotonic chain with a neutron number N follows a similar expression.$ \begin{aligned}[b] Q_{\alpha 2}=&\;Q_{\alpha 1}-(\beta _{2}-\beta _{1})\Bigg[ \dfrac{2^{5/3}}{9} a_{c}N^{2/3}(1+\beta )^{-5/3}(11\\&+5\beta +2\beta ^{2})+8a_{\text{sym}}\beta \Bigg] . \end{aligned} $

(2) Equations (1), (2) tend to realize high accuracy when a shell is not crossed during α-decay [47]. However, if a shell is crossed, the deviations are likely to be substantial, as these equations do not take shell effects into account. This is positive news as it enables the investigation of shell closures by comparing calculated results with experimental data. A significant deviation between the calculated and experimental results could indicate the presence of a shell closure.
The calculated α-decay energies
$ Q_{\alpha} $ for nuclei with$Z=96-105, N=152$ subtracted by the corresponding experimental values, i.e.,$ \Delta Q=Q_{\text{Eq}.(1)}-Q_{\text{exp}} $ , are plotted in Fig. 2. In this analysis, the target nucleus has the same proton number as the reference nuclei, with the$ Q_{\alpha} $ value of the reference nucleus obtained from experimental data. A significant non-zero value of$ \Delta Q $ suggests the presence of a shell effect. As depicted in Fig. 2, when the neutron number of the reference nucleus is close to but below$ N=152 $ ,$ \Delta Q $ values are relatively small. However, they become substantial when the reference nuclei with$ N=154 $ are used. Specifically,$ \Delta Q $ values for$Z= 102, N=152$ and$ Z=103, N=152 $ in Fig. 2 are 0.6 MeV and 0.8 MeV, respectively. If the existence of$ N=152 $ shell in$ Z=102 $ and no isotope is confirmed, as claimed in Ref. [36], then this neutron shell can also be pinned in$ Z=101,104,105 $ isotopes. This is due to the fact that$ \Delta Q $ values are approximately 0.6−0.8 MeV for these three nuclei, which are as large as that in$ Z=102 $ case. For other nuclei ($Z=96-100$ ),$ \Delta Q $ values are just 0.4 MeV, indicating a relatively weak shell stabilization at$ N=152 $ in these nuclei. For nuclei with$ Z>105 $ , drawing definitive conclusions is hindered by the lack of experimental data. Hence,$ N=152 $ is a shell gap in$ Z=101-105 $ isotopes ($ ^{253}\text{Md} $ ,$ ^{254}\text{No} $ ,$ ^{255}\text{Lr} $ ,$ ^{256}\text{Rf} $ ,$ ^{257}\text{Db} $ ), reinforcing the findings from Fig. 1(a), and$ N=152 $ shell gradually weakens for lighter nuclei. Therefore, with the aid of mere knowledge about the measured α-decay properties combined with our methodology outlined in Ref. [47], some valuable structural information about SHN is uncovered. The experimental$ Q_{\alpha} $ values of$ N=152 $ isotones are accurately reproduced by applying Eq. (1) with$ N<152 $ reference nuclei, indicating not only the consistency of the experimental measurements but also the reliability of Eq. (1) to predict$ Q_{\alpha} $ values of unobserved SHN if shell closure is not crossed for α-decay.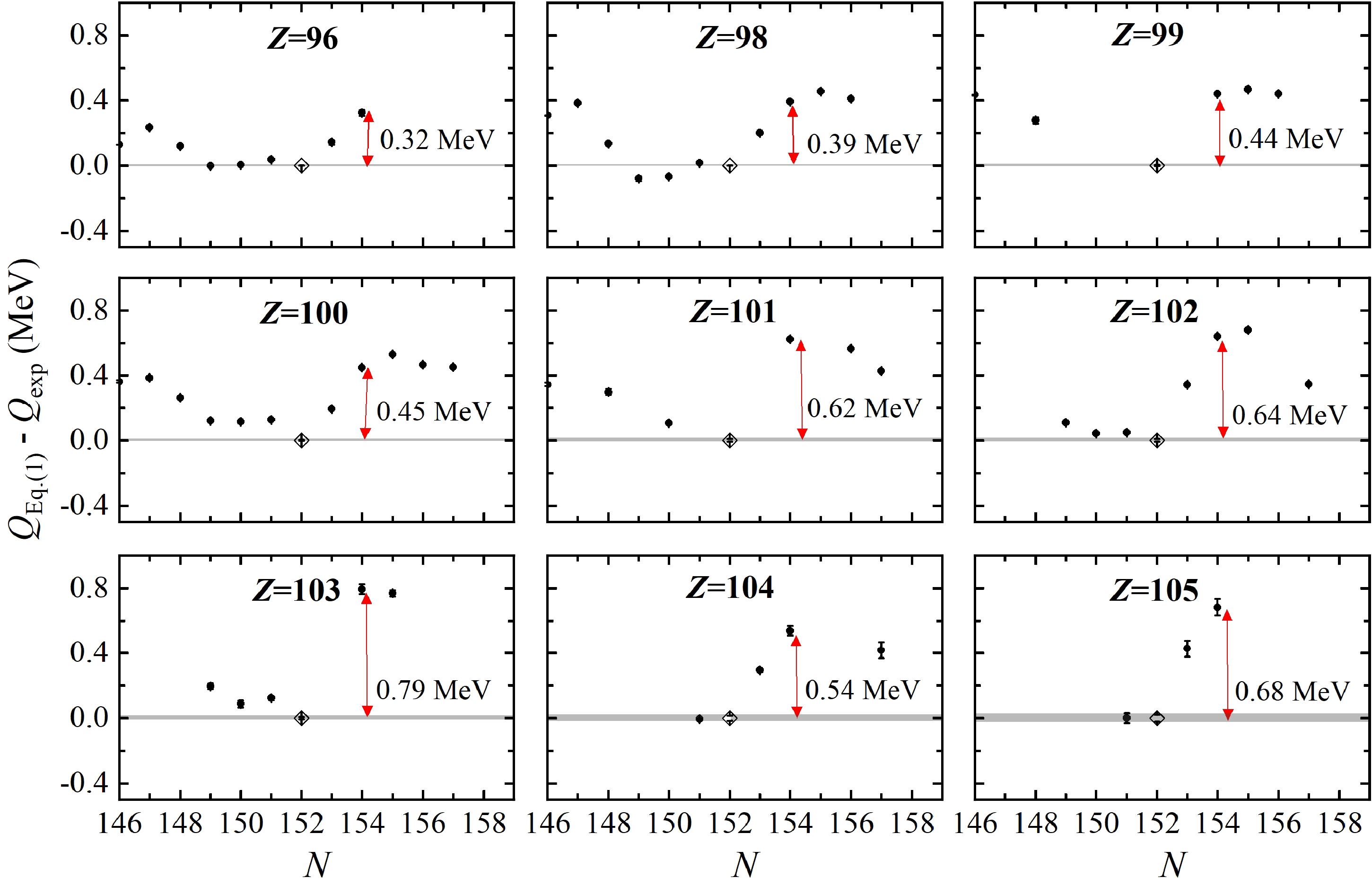
Figure 2. (color online) Calculated α-decay energies
$ Q_{\alpha} $ for nuclei with$ Z=96-105 $ and$ N=152 $ (circular symbols) are obtained by applying Eq. (1), subtracted by the corresponding experimental data [46] (diamond symbols). The reference nucleus and target nucleus share the same proton number but differ in neutron number. The error bars in the calculated$ Q_\alpha $ originate from error bars in the experimental$ Q_\alpha $ of reference nuclei. The horizontal axis denotes the neutron number of reference nuclei.Similarly, Fig. 3 exhibits Eq. (2)-calculated
$ Q_{\alpha} $ values of SHN with$ Z=108 $ and$ N=157-160 $ subtracted by the corresponding experimental values, i.e.,$\Delta Q= Q_{\text{Eq}.(2)}-Q_{\text{exp}}$ , aiming to reveal whether$ Z=108 $ proton shell exists or not for other$ Z=108 $ isotopes in addition to the well-known doubly-magic nucleus$ ^{270} $ Hs. In contrast to Fig. 1(b), Fig. 3 provides a clear and intuitive representation. When the proton number of the reference nucleus is close to but below$ Z=108 $ ,$ \Delta Q $ values are relatively small. However, if the nuclei with$ Z=110 $ are selected as reference nuclei, then$ \Delta Q $ is as large as 0.6 MeV for$ Z=108 $ isotopes with$ N=159,160 $ , comparable in magnitude to the aforementioned$ \Delta Q $ for examining$ N=152 $ shell in$ ^{255} $ Lr. This indicates a shell closure at$ Z=108 $ for these two nuclei. Due to a lack of experimental data, definitive conclusions about the shell structure for other nuclei cannot be drawn at present. Therefore, more experimental data on the nuclear mass or decay energy are required. Nevertheless, the identified robust proton shell$ Z=108 $ in Hs isotopes with$ N= $ 159, 160 along with robust neutron shell$ N=152 $ in$Z=101-105$ isotopes provide crucial benchmarks for current nuclear energy-density functionals.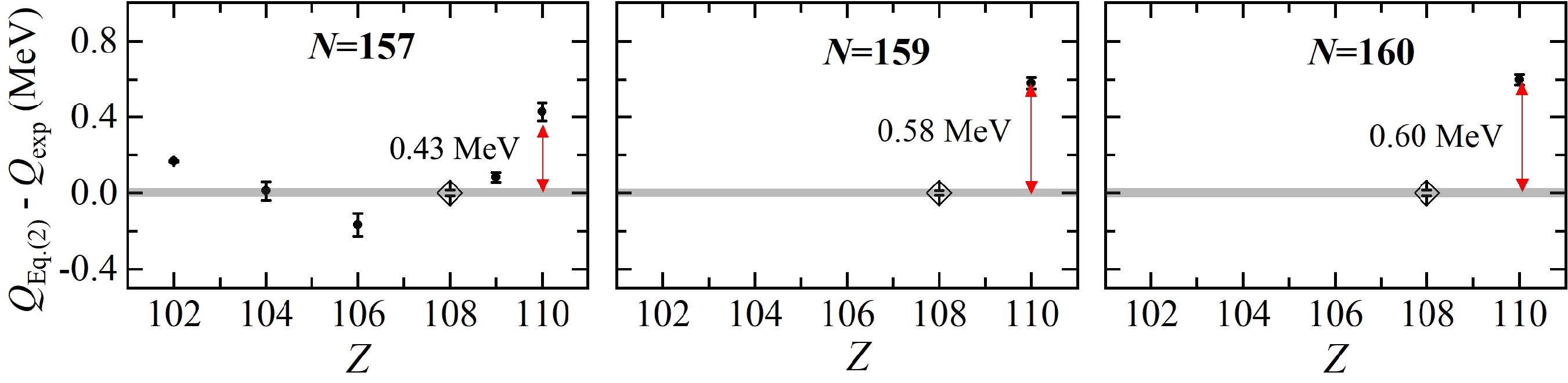
Figure 3. (color online) Calculated α-decay energy
$ Q_{\alpha} $ of Hs isotopes ($ Z=108 $ ) with$N=155-167$ (circular symbols) are obtained by applying Eq. (2), subtracted by the corresponding experimental values [46] (diamond symbols). The reference nucleus and target nucleus share the same neutron number but differ in proton number. The horizontal axis denotes the proton number of the reference nuclei.We calculate
$ Q_{\alpha} $ values of SHN with$Z=96-105$ and$N=136-157$ in the framework of a widely-used energy density functional approach, i.e., axially deformed relativistic mean field theory combined with the Bardeen-Cooper- Schrieffer approximation (RMF+BCS), and the results are displayed in Fig. 4. It can be observed that some irregular behaviors are displayed at$ N=152 $ , just with$Z=104-105$ , when FSUGarnet interaction is employed. However, for other interactions, these types of irregular behaviors cannot be reproduced. This implies that RMF+BCS approach cannot generally provide the location of deformed$ N=152 $ shell. Therefore, the neutron shell$ N=152 $ in$Z=101-105$ isotones is highly beneficial for future theoretical improvements to better adapt to the superheavy mass region.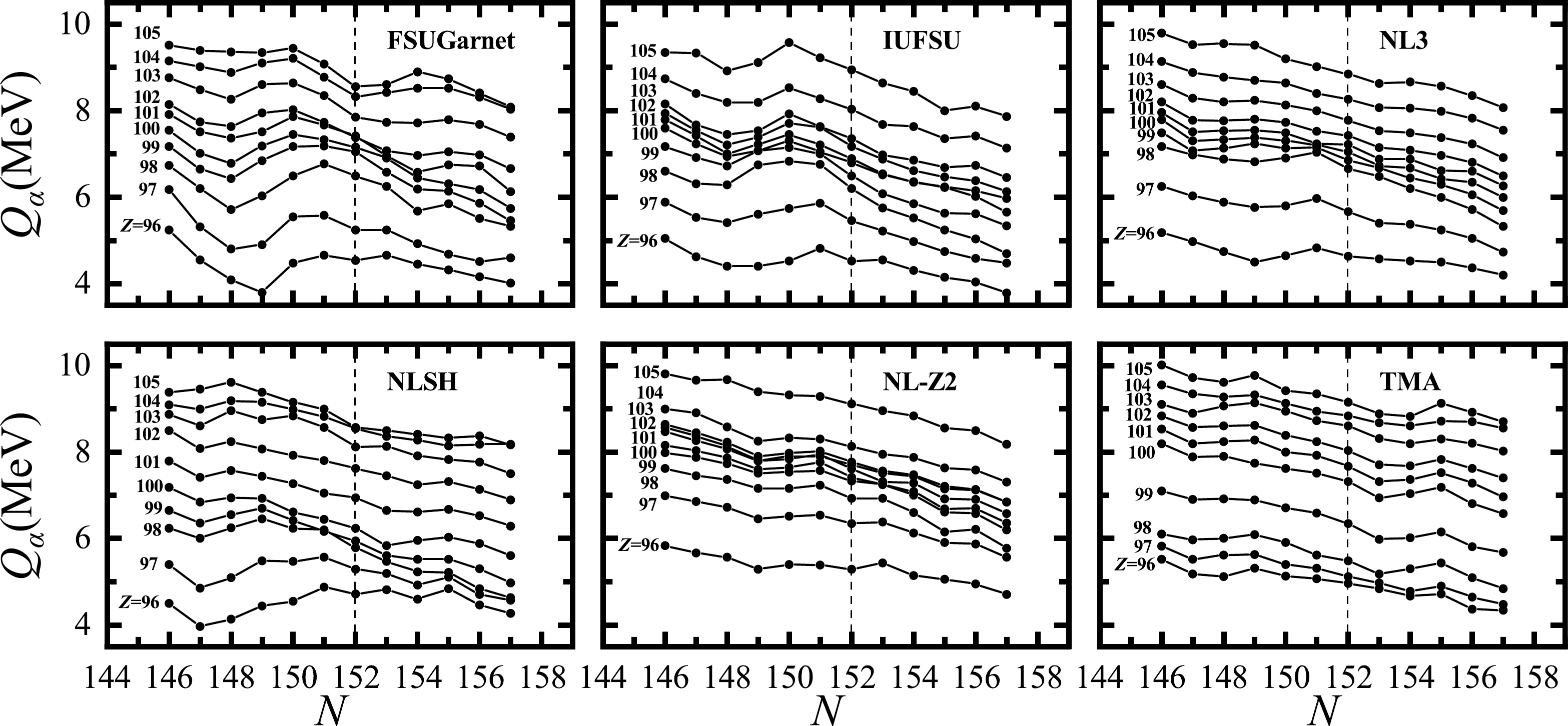
Figure 4. Calculated α-decay energy
$ Q_{\alpha} $ for the isotopic chains with proton numbers$ Z=96 $ to 105 as a function of neutron number N. The calculations are performed by employing RMF+BCS method with six parameters: FSUGarnet [50], IUFSU [51], NL3 [52], NLSH [53], NL-Z2 [21], and TMA [54].In summary, we investigated the robustness of
$ N=152 $ and$ Z=108 $ shell closures. Although the center of the 'island of stability' for superheavy nuclei (SHN) has not yet been reached, and the spherical magic numbers in the superheavy region remain unidentified,$ N=152 $ neutron shell in$ Z=102,103 $ isotopes and$ Z=108 $ proton shell in$ ^{270}\text{Hs} $ ($ N=162 $ ) has been confirmed based on experimental measurements. The stability of$ N=152 $ and$ Z=108 $ shell closures in other nuclei is examined based on alpha decay energy$ Q_{\alpha} $ via two different methods. A method involves investigating the irregular behavior of$ Q_{\alpha} $ along isotopic chains and isotonic chains, but it exhibits evident drawback for probing proton shells. The other method, as an alternative strategy, recognizes shell closures and shell evolution by comparing the experimental and theoretical α-decay energy$ Q_{\alpha} $ , where the theoretical one is based on the analytic formulas to calculate the$ Q_{\alpha} $ value with the aid of experimentally measured$ Q_{\alpha} $ values of its neighbors. The robust$ N=152 $ shell is identified in$Z=101-105$ isotopes, and$ Z=108 $ proton shell appears in Hs isotopes with$ N=159,160 $ . A weakening of$ N=152 $ ($ Z=108 $ ) shell stabilization in$ Z=96-100 $ isotopes ($ ^{265}\text{Hs} $ ) is suggested. Whether$ N=152 $ or$ Z=108 $ is a magic number or not for other nuclei remains unknown due to insufficient experimental data. Additionally, the$ Q_{\alpha} $ values of these SHN have been computed by applying the RMF+BCS approach, and the shell of$ N=152 $ cannot be reproduced generally. Therefore, the conclusions drawn in this study could serve as crucial calibrations for reliable construction of effective interactions applied in superheavy mass region in nuclear many-body approaches. The present study provides a valid strategy to explore the locations of shell closures in superheavy region with the mere information about measured α-decay energies.
Robustness of N=152 and Z=108 shell closures in superheavy mass region
- Received Date: 2024-09-26
- Accepted Date: 2024-10-24
- Available Online: 2025-01-15
Abstract: The neutron shell gap at






 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF























 DownLoad:
DownLoad: