Revisiting the alpha-decay reduced width of the lightest uranium isotope ²¹⁴U

Alpha decay is a transformation that occurs when a nucleus spontaneously emits an α particle. It is one of the main decay modes of heavy and superheavy nuclei (SHN). In the 1920s, Gamow [1] and Gurney and Condon [2] independently used quantum mechanics to describe α-decay, the process by which a preformed α-particle tunnels through a barrier created by the α-particle and residual nucleus. Since then, many theoretical models have been proposed to describe the nuclear interaction potential between the α-particle and daughter nucleus and to calculate the penetration probability (P) in α-decay [3−11]. The Wentzel-Kramers-Brillouin (WKB) approximation is a quasi-classical approximation for solving the one-dimensional Schrödinger equation proposed by Wenzel, Kramers, and Brillouin. It has been very widely used to calculate the penetration probability of a particle across potential barriers in quantum tunneling processes, such as α-decay and heavy-ion fusion reactions in nuclear physics. The transfer matrix (TM) approach uses multistep functions (multistep potential approximation) to approximate an arbitrary potential; subsequently, the wave function in each region can be calculated analytically, and the penetration probability is calculated by connecting momentum eigenfunctions [12]. The TM method can accurately calculate the penetration probability across arbitrary potential barriers when the number of segment is sufficiently large. For α-decay, the penetration penetrability obtained using the WKB approximation is about 30%−40% smaller than the accurate result obtained using the TM approach [13].

The synthesis of SHN has attracted much interest in the field of nuclear physics for a long time in the search for the possible existence of "island of superheavy nuclei". The α-decay of SHN is of central interest in both experimental and theoretical studies, not only because α-decay is the main means of identifying SHN experimentally, but also because the α-decay reduced width $ \delta^{2} $, which is deduced from the measured half-life and the calculated penetration probability, is considered to encode rich information on the structure of nuclei.

Uranium is the heaviest element in nature. $ {\rm^{234}U} $, $ {\rm^{235}U} $, and $ {\rm^{238}U} $ are the three isotopes of uranium that can be found in nature. Recently, the lightest uranium isotope $ {\rm^{214}U} $ has been produced at the Heavy Ion Research Facility in Lanzhou, China, which has attracted widespread interest in the nuclear physics community [14]. With the Rasmussen method, the $ \delta^{2} $ of $ {\rm^{214,216}U} $ is found to be significantly enhanced by a factor of 2 compared with other nuclei in the $ N_pN_n $ systematics [14]. As the extraction of $ \delta^{2} $ is model dependent, it relates to the choice of the α-core potential and the method of obtaining the penetration probability. Therefore, we must investigate whether these two factors affect the conclusion that the $ \delta^{2} $ of $ {\rm^{214,216}U} $ is significantly larger than those of the surrounding nuclei. Hence, four different phenomenological α-core potentials and two microscopic double-folding potentials, together with the WKB approximation and TM approach, are used to obtain the penetration probability. The α-decay reduced width can be derived from the α-preformation probability. Various effects (e.g., nuclear deformation, isospin asymmetry, nuclear shells and pairing) on the α-preformation probability have been extensively studied, see e.g., Refs. [15−18]. In this work, we focus on the influences of nuclear deformation and the α-core potential on the α-decay reduced width in the $ N_{p}N_{n} $ systematics.

The remainder of this paper is organized as follows: In Sect. II, the WKB approximation, TM approach, and α-core potentials are introduced. In Sect. III, the ratios of the $ \delta^{2} $ of $ {\rm^{214}U} $ to those of the surrounding nuclei for different methods of calculating P and different α-core potentials are given and compared. The summary is given in Sect. IV

The α-decay reduced width $ \delta^{2} $ is expressed as [19]

where $ T_{1/2} $ is the experimental half-life for α-decay, and P is the penetration probability. In this work, the half-life are obtained from Refs. [14, 20].

Using the WKB approximation, the probability of penetration of a particle penetrating a barrier V(r) at an incident energy of Q can be obtained:

where μ is the mass of the particle. The turning points $ r_{2} $ and $ r_{3} $ are determined from the equation $ V(r_{2})= V(r_{3})=Q $. This method of calculating the barrier penetration probability has certain limitations. The WKB approximation is considered to better calculate the penetration probability at energies significantly below the barrier peak and for the potentials that are slowly varying [21].

The TM approach assumes that an arbitrary potential barrier can be split into segments. When the number of segments is sufficiently large, this method can reasonably describe the arbitrary potential barrier. Assuming that the barrier is equally divided into N segments, the potential energy of each segment is expressed as

with $ r_{j-1} $ < r < $ r_{j}\ (j=0, 1, 2,... N, N+1) $. The wave function of a particle with energy Q in the jth region is given by

Here, $ k_{j} $=$ \sqrt{2\mu(Q-V_{j})}/{\hbar} $ is the wave number. The coefficients $ A_{j} $ and $ B_{j} $ can be obtained from the continuity of ψ(r) and its derivative at each boundary. By setting $ A_{0} $ = 1 and $ B_{N+1} $ = 0, we can calculate the penetration probability as follows:

where $ A_{n+1} $=$ \dfrac{k_{0}}{k_{n+1}} $$ \dfrac{1}{M_{22}} $. $ {M_{22}} $ is given by

where

The accuracy of the TM approach can be validated with the $ V(x) = V_{0}\cosh^{-2}(x/a) $ potential, for which the exact analytic transmission probability is known [22, 23]. Thus, the penetration probability obtained using the TM approach is quoted as the exact value throughout the paper.

Often, the α-core potential is composed of nuclear, Coulomb, and centrifugal terms:

In this paper, the following six α-core potentials are considered.

(1) In a cluster model proposed by Buck et al. in Ref. [24], a cosh form of nuclear potential was used. It is given by

where $ V_{0} $ is the depth, a is the diffuseness parameter, and R is the radius. A Langer modified centrifugal barrier $\dfrac{\hbar^{2} }{2\mu }\dfrac{(L+{1}/{2})^2}{r^2}$ is used instead of $ \dfrac{\hbar^{2} }{2\mu }\dfrac{L(L+1)}{r^2} $. This modification is necessary when moving from the one-dimensional problem to three-dimensional problems [25, 26]. The Coulomb potential $ V_{C}(r) $ is taken as a form appropriate to a point α-particle interacting with a uniformly charged spherical core of radius R:

R in the above two equations is determined by the Bohr-Sommerfeld quantization condition

Here, G is the global quantum number, and $ Q_{\alpha} $ is the α-decay energy. The classic turning points ($ {r_{1}} $, $ {r_{2}} $, and $ {r_{3}} $ in order of increasing distance from the origin) are solved using $ V(r)=Q_{\alpha} $. The three free parameters are set to $ V_{0}=162.3 $ MeV, a = 0.40 fm, $ G=20 $ (for N$ \le126 $), G = 22 (for N$ \ge126 $). They are determined using a best fit to the available data [24]. This potential is termed Pot1.

(2) In our recent work, an isospin-dependent depth parameter $ V_{0} $=152.5(1+0.2 $ \dfrac{N-Z}{N+Z} $) and an adjusted diffuseness parameter $ a=0.39 $ fm in Eq.(10) are obtained by fitting the experimental half-lives of uranium isotopes [27]. This potential is termed Pot2.

(3) In Ref. [13], a mass and charge number dependent α-core potential was obtained using the following expression:

with $ U_{0} =[53-33(N-Z)/A] $ MeV, $ R_{0}=1.27A^{1/3} $ fm, $ a= 0.67 $ fm. $ A_{1} $ is the mass number of the emitted particle. N, Z, and A are the neutron, proton, and mass numbers of the parent nucleus, respectively. The Coulomb potential is given by Eq. (11) with radius $R=1.28A^{1/3}- 0.76+0.8A^{-1/3}$ fm. This potential is termed Pot3.

(4) In the Rasmussen method, the α-core potential is obtained from the real part of a potential deduced by Igo to fit alpha elastic-scattering data [28, 29]. It is expressed as

where A is the mass number of the parent nucleus. This potential is termed Pot4.

(5) Unlike the previous phenomenological α-core potentials, the double-folding potential uses the microscopic nuclear and realistic Coulomb potentials [30]:

where λ is the renormalized factor. In this work, λ=0.6 is used according to the values in Ref. [30]. In some studies, the value of λ is determined using the Bohr-Sommerfeld quantization condition, see e.g., [15, 31], whereas others use a constant value of λ, e.g., Refs. [6, 30, 32]. As discussed in Ref. [32], the variation in λ is small in both spherical and deformed cases for different nuclei. We have checked that the extracted $ \delta^{2} $ is influenced when varying λ; however, its effect on the α-decay reduced width ratio is weak. Therefore, a constant value of λ is used in this paper for simplicity. $ \boldsymbol{r } $ is the distance between the mass centers of the α-particle and core. $\boldsymbol{r_{1} }$ and $ \boldsymbol{r_{2} } $ are the nucleon coordinates belonging to the α -particle and daughter nucleus, respectively. The quantity $ \left | \boldsymbol{s } \right | $ is the distance between a nucleon in the core and a nucleon in the α-particle. $ \rho_{1} $ and $ \rho_{2} $ are the mass density distributions of the α-particle and core.

where $ \rho_{0} $ is fixed by the mass numbers of the daughter nucleus ($ A_{d} $), $ c=1.07A_{d}^{1/3} $, and $ a=0.54 $ fm.

$ \rho'_{1} $ and $ \rho'_{2} $ in Eq. (16) are the charge density distributions of the α-particle and daughter nucleus, respectively.

The value of $ \rho'_{0} $ is fixed by the charge numbers of the α-particle and daughter nucleus.

$ g(E,\left | \boldsymbol{s } \right |) $ in Eq. (15) is the microscopic M3Y nucleon-nucleon interaction potential:

$ E_{\alpha} $ and $ A_{\alpha} $ denote the energy and mass number of the cluster, respectively. This potential is termed Pot5.

(6) The majority of all known atomic nuclei have varying degrees of deformation. The deformed double-folding potential considers the axial deformation of the daughter nuclei [32]. The α-core potential is

where β is the orientation angle of the α-particle relative to the symmetry axis of the daughter nucleus.

For the deformed residual nucleus, its density distribution is related to the deformation parameters:

where the half-density radius $ R(\theta) $ is given by

Here, $ R_{0} = 1.07A_{d}^{1/3} $ fm and $ a = 0.54 $ fm. In this work, only the contribution of $ \beta_2 $ is considered for simplicity. This potential is termed Pot6.

In Pot6, the P of α-decay is

where the values of $ r_{2}(\beta) $ and $ r_{3}(\beta) $ can be calculated using $ Q_{\alpha} = V(r,\beta) $. The total penetration factor P is obtained by

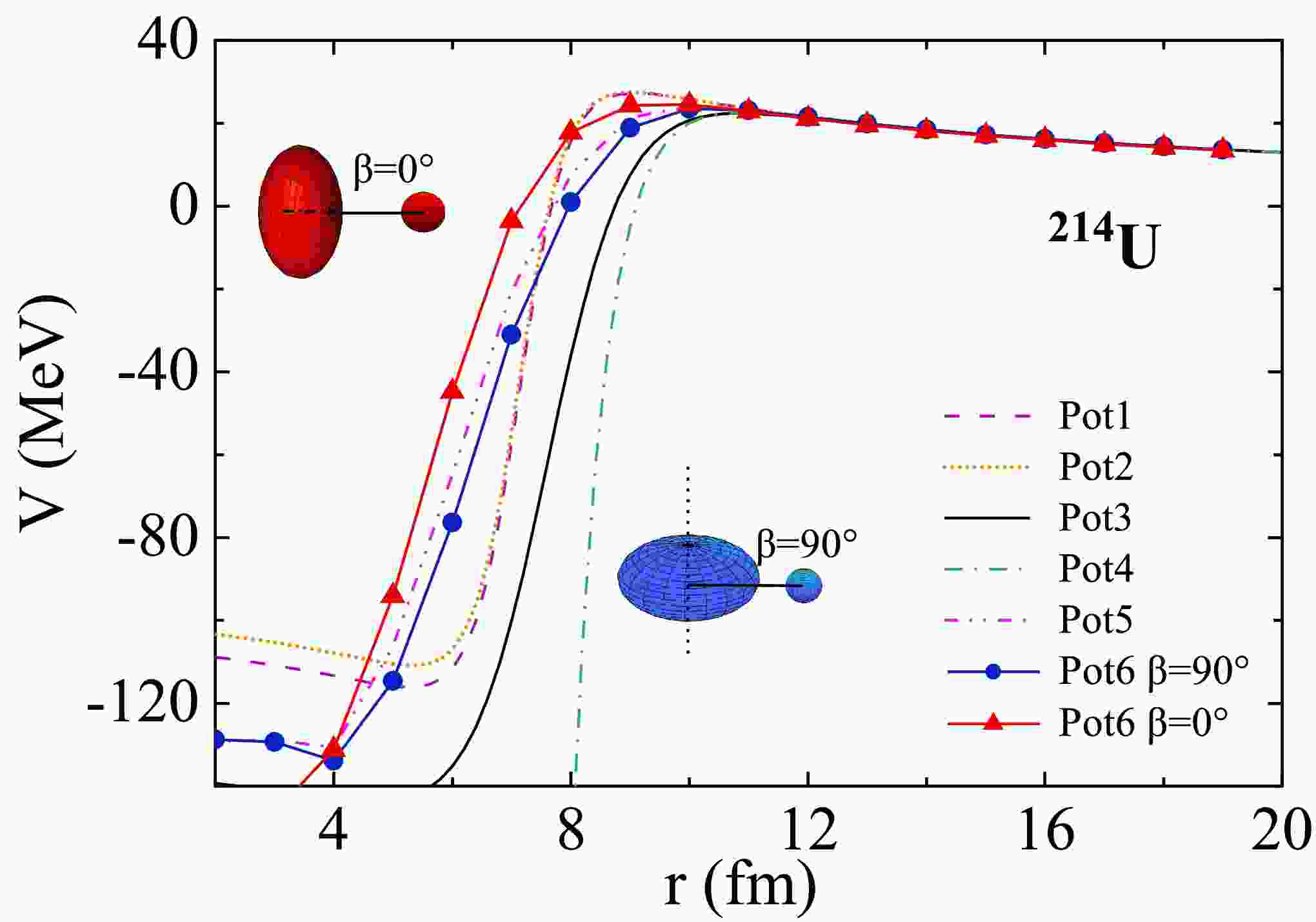
The six potential barriers for the α-decay of $ {\rm^{214}U} $ are shown in Fig. 1. The potentials for two different orientations β=0° and 90° in Pot6 are shown as red solid lines with triangles and blue solid lines with circles, respectively. These potentials are significantly different, which can lead to a large difference in the penetration probability P of the α-particle through each potential because P depends strongly on the height and width of the potential barrier. Consequently, the α-decay reduced width $ \delta^{2} $ can be considerably different correspondingly.

Figure 1.  (color online) Six α-core potentials of $ {\rm^{214}U} $. $ \beta = 0^{\circ} $ and $ \beta = 90^{\circ} $ are two different orientations of the $ \alpha+{\rm^{210}Th} $ system under Pot6.

The WKB approximation is more reliable with a gentle variation in potential, i.e., only slightly change over the de Broglie wavelength. Its computational accuracy in studies on heavy-ion fusion, alpha decay, and proton and cluster radioactivity has been discussed [13, 33, 34]. Ref. [13], for Pot3, found that P of the α-decay obtained using the WKB approximation is about 30%−40% smaller than the exact one obtained with the TM approach. In this work, the accuracy of the WKB approximation to calculate P is examined using different α-core potentials. The relative deviation of the WKB approximation can be examined using $RD=(P_{\mathrm{WKB}}-P_{\rm TM})/ P_{\rm TM}\times$100%. $P_{\rm WKB}$ and $P_{\rm TM}$ denote the penetration probabilities obtained using the WKB approximation and TM approach, respectively. In this work, the α-decay of nuclei around $ {\rm^{214}U} $ is considered. The experimental $ Q_{\alpha} $ values are obtained from Refs. [14, 35]. The RD values obtained with different α-core potentials are plotted as a function the mass number of parent nuclei in Fig. 2. For Pot1 and Pot2, the RD values are almost constant (about –40%), whereas for Pot3 and Pot4, the RD values vary from about –20% to –40%. This is because both Pot3 and Pot4 are dependent on the mass number, whereas Pot1 and Pot2 are not.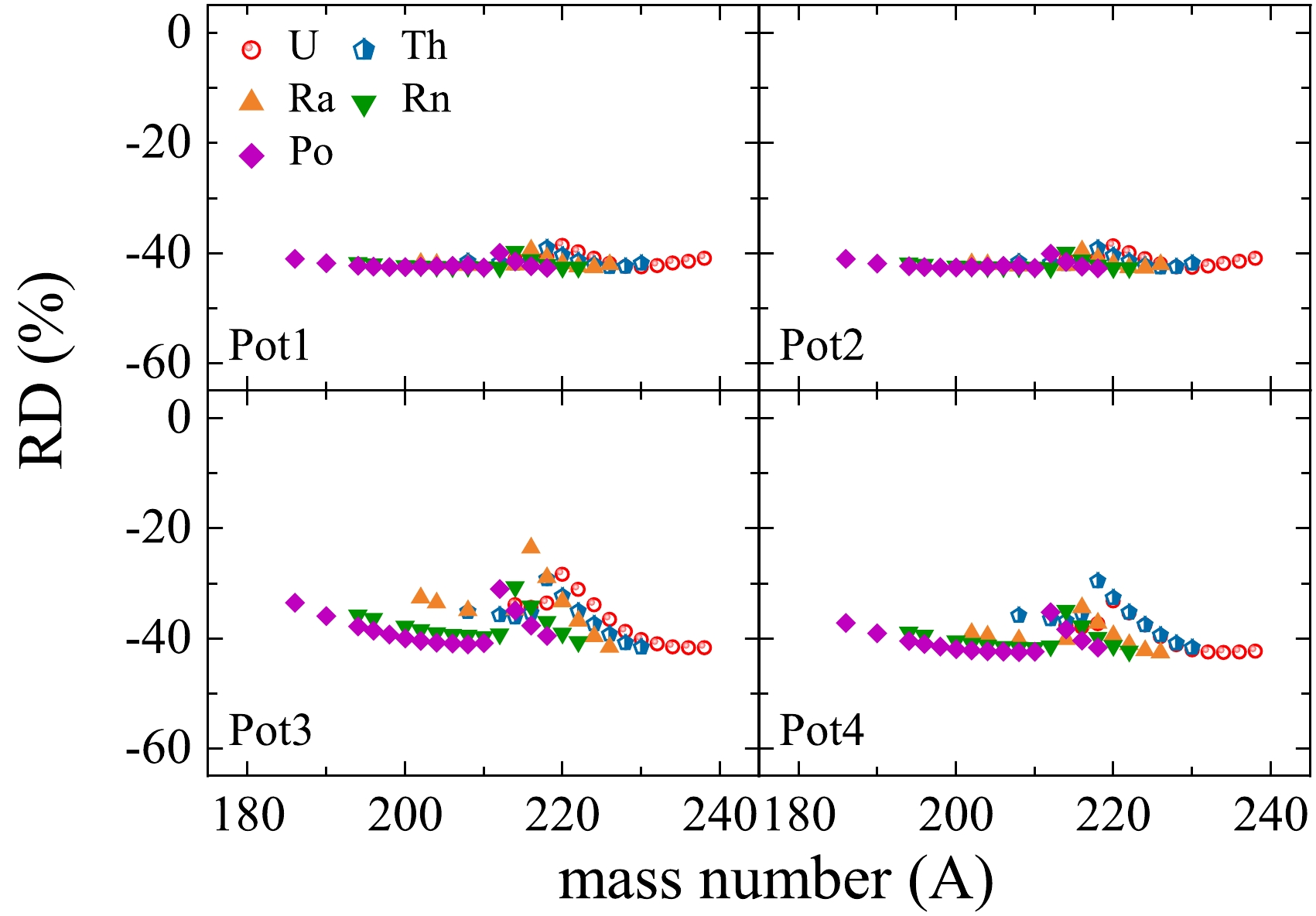

Figure 2.  (color online) Relative deviation in the penetration probability caused by the WKB approximation of α-decay for Pot1-Pot4.

Using the WKB approximation and TM approach, we extract$ \delta^{2} $ of the ground state to ground state decays of $ {\rm^{214}U} $ and the surrounding nuclei for Pot1-Pot4, which is shown in Fig. 3 as function of $ N_{p}N_{n} $. Here, $ N_{p} $ and $ N_{n} $ are the proton and neutron numbers relative to the nearest closed shell Z = 82 and N = 126, respectively. In this work, the error in $ \delta^{2} $ is caused only by the uncertainty of the experimental half-life [14]. Figure 3 clearly shows that$ \delta^{2} $ is model-dependent. Nevertheless, as discussed in Ref. [36], rich information about the structural properties of nuclei can be gained from the trend in $ \delta^{2} $ of different isotopes, rather than from $ \delta^{2} $ itself. Comparing the results in the left column of Fig. 3, we observe that the values of $ \delta^2 $ for different α-core potentials vary by almost three orders of magnitude, from $ 10^{2} $ to $ 10^{5} $. According to Eq. (1), for the same nucleus, the only factor that affects the value of the $ \delta^2 $ is P. In Fig. 1, Pot1 has the largest barrier width, resulting in a lower probability of the α-particle crossing this barrier. Therefore, the value of $ \delta^2 $ obtained with Pot1 is the largest. If we compare the results in the left and right panels, for the same α-core potential, we find that the values of $ \delta^2 $ obtained with the TM approach are smaller than those obtained with the WKB approximation. This is owing to the underestimation of P in the WKB approximation. In addition, in all cases, the $ \delta^2 $ of $ {\rm^{214,216}U} $ is larger than those of surrounding nuclei. This is consistent with the results presented in the experimental paper Ref. [14] in which Pot4 and the WKB approximation were used.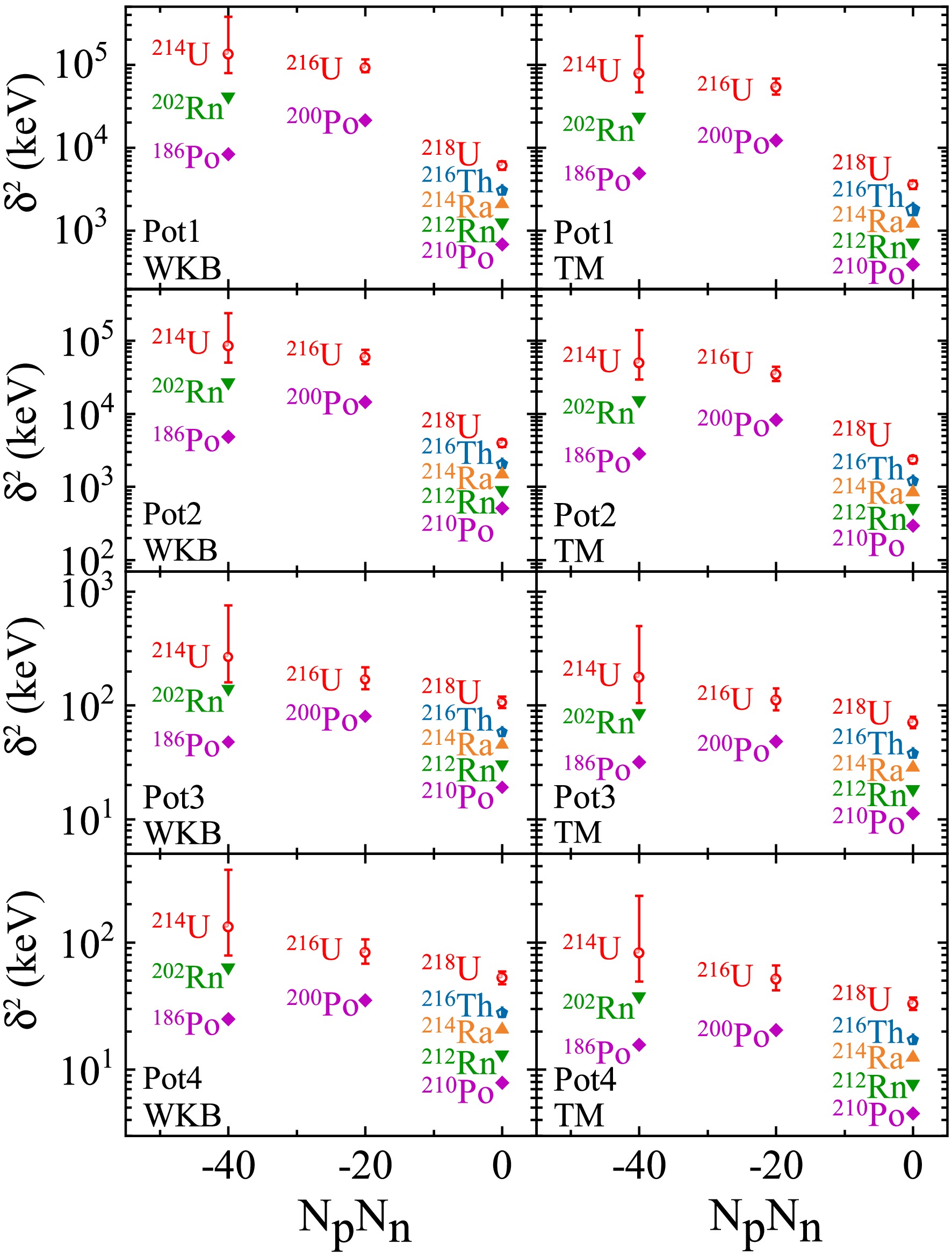

Figure 3.  (color online) α-decay reduced width $ \delta^{2} $ of $ {\rm^{214}U} $ and the surrounding nuclei obtained using the WKB approximation (left panels) and the TM method (right panels) with different α-core potentials. The $ N_{p} $ and $ N_{n} $ values are proton and neutron number relative to $ Z = 82 $ and $ N = 126 $ closed shells, respectively.

To quantitatively study whether $ \delta^2 $ of $ {\rm^{214,216}U} $ is significantly enhanced by a factor of 2 compared with those of the surrounding nuclei in the $ N_{p}N_{n} $ systematics, we list the ratios of $ \delta^2 $ of $ {\rm^{214,216,218}U} $ to those of surrounding nuclei with the same $ N_{p}N_{n} $ in Table 1. First, the $ \delta^2 $ ratio between $ {\rm^{214}U} $ ($ {\rm^{216}U} $) and $ {\rm^{202}Rn} $ ($ {\rm^{200}Po} $) is larger than 2 for Pot1-Pot4. In Pot1, the $ \delta^2 $ ratio of $ {\rm^{214}U} $ to $ {\rm^{202}Rn} $ and $ {\rm^{216}U} $ to $ {\rm^{200}Po} $ are the largest (3.29 and 4.33, respectively). The conclusion that $ \delta^2 $ of $ {\rm^{214,216}U} $ is enhanced appears to hold even if the value of $ \delta^2 $ may vary several orders of magnitude for different α-core potentials. Second, we find that the values of the $ \delta^2 $ ratio obtained using the WKB approximation are considerably close to those obtained with the TM approach, which means that both the WKB approximation and TM approach can be used to study the α-decay reduced width. By considering the fact that the TM approach is much more time consuming than the WKB approximation, in the following discussions, we focus on the results obtained using the WKB approximation.

α−core potential	$ N_{p}N_{n} $	$ \delta^2 $ ratio	WKB method	TM approach
Pot1	−40	$ {\rm^{214}U} $ / $ {\rm^{202}Rn} $	$ 3.22_{-1.30}^{+5.89} $	$ 3.29_{-1.33}^{+6.01} $
		$ {\rm^{214}U} $ / $ {\rm^{190}Po} $	$ 5.97_{-2.41}^{+10.91} $	$ 6.04_{-2.44}^{+11.04} $
		$ {\rm^{214}U} $ / $ {\rm^{186}Po} $	$ 15.96_{-6.44}^{+29.15} $	$ 15.92_{-2.44}^{+29.09} $
	−20	$ {\rm^{216}U} $ / $ {\rm^{200}Po} $	$ 4.24_{-0.44}^{+1.19} $	$ 4.33_{-0.77}^{+1.21} $
	0	$ {\rm^{218}U} $ / $ {\rm^{216}Th} $	$ 2.00_{-0.22}^{+0.24} $	$ 2.02_{-0.21}^{+0.25} $
		$ {\rm^{218}U} $ / $ {\rm^{214}Ra} $	$ 2.87_{-0.31}^{+0.35} $	$ 2.94_{-0.32}^{+0.36} $
		$ {\rm^{218}U} $ / $ {\rm^{212}Rn} $	$ 4.83_{-0.52}^{+0.59} $	$ 4.98_{-0.54}^{+0.61} $
		$ {\rm^{218}U} $ / $ {\rm^{210}Po} $	$ 8.82_{-0.95}^{+1.09} $	$9.10_{ - 0.98}^{ + 1.12}$
Pot2	−40	$ {\rm^{214}U} $ / $ {\rm^{202}Rn} $	$ 3.13_{-1.26}^{+5.71} $	$ 3.19_{-1.29}^{+5.84} $
		$ {\rm^{214}U} $ / $ {\rm^{190}Po} $	$ 6.25_{-2.52}^{+11.41} $	$ 6.32_{-2.55}^{+11.55} $
		$ {\rm^{214}U} $ / $ {\rm^{186}Po} $	$ 17.41_{-7.03}^{+31.82} $	$ 17.37_{-7.01}^{+31.73} $
	−20	$ {\rm^{216}U} $ / $ {\rm^{200}Po} $	$ 4.07_{-0.72}^{+1.14} $	$ 4.15_{-0.74}^{+1.16} $
	0	$ {\rm^{218}U} $ / $ {\rm^{216}Th} $	$ 1.94_{-0.21}^{+0.24} $	$ 1.96_{-0.21}^{+0.24} $
		$ {\rm^{218}U} $ / $ {\rm^{214}Ra} $	$ 2.69_{-0.29}^{+0.33} $	$ 2.75_{-0.30}^{+0.34} $
		$ {\rm^{218}U} $ / $ {\rm^{212}Rn} $	$ 4.37_{-0.47}^{+0.54} $	$ 4.51_{-0.49}^{+0.55} $
		$ {\rm^{218}U} $ / $ {\rm^{210}Po} $	$ 7.72_{-0.83}^{+0.95} $	$ 7.96_{-0.86}^{+0.98} $
Pot3	−40	$ {\rm^{214}U} $ / $ {\rm^{202}Rn} $	$ 1.90_{-0.77}^{+3.46} $	$ 2.04_{-0.83}^{+3.73} $
		$ {\rm^{214}U} $ / $ {\rm^{190}Po} $	$ 2.33_{-0.94}^{+4.26} $	$ 2.40_{-0.97}^{+4.39} $
		$ {\rm^{214}U} $ / $ {\rm^{186}Po} $	$ 5.55_{-2.24}^{+10.13} $	$ 5.51_{-2.23}^{+10.07} $
	−20	$ {\rm^{216}U} $ / $ {\rm^{200}Po} $	$ 2.10_{-0.37}^{+0.59} $	$ 2.29_{-0.41}^{+0.64} $
	0	$ {\rm^{218}U} $ / $ {\rm^{216}Th} $	$ 1.82_{-0.20}^{+0.22} $	$ 1.87_{-0.20}^{+0.23} $
		$ {\rm^{218}U} $ / $ {\rm^{214}Ra} $	$ 2.35_{-0.25}^{+0.29} $	$ 2.49_{-0.27}^{+0.31} $
		$ {\rm^{218}U} $ / $ {\rm^{212}Rn} $	$ 3.50_{-0.38}^{+0.43} $	$ 3.82_{-0.41}^{+0.47} $
		$ {\rm^{218}U} $ / $ {\rm^{210}Po} $	$ 5.58_{-0.60}^{+0.69} $	$ 6.27_{-0.67}^{+0.77} $
Pot4	−40	$ {\rm^{214}U} $ / $ {\rm^{202}Rn} $	$ 2.08_{-0.83}^{+3.79} $	$ 2.17_{-0.89}^{+4.00} $
		$ {\rm^{214}U} $ / $ {\rm^{190}Po} $	$ 2.35_{-0.95}^{+4.30} $	$ 2.41_{-0.97}^{+4.40} $
		$ {\rm^{214}U} $ / $ {\rm^{186}Po} $	$ 5.30_{-2.14}^{+9.68} $	$ 5.26_{-2.12}^{+9.61} $
	−20	$ {\rm^{216}U} $ / $ {\rm^{200}Po} $	$ 2.36_{-0.42}^{+0.66} $	$ 2.52_{-0.45}^{+0.70} $
	0	$ {\rm^{218}U} $ / $ {\rm^{216}Th} $	$ 1.89_{-0.20}^{+0.23} $	$ 1.93_{-0.21}^{+0.24} $
		$ {\rm^{218}U} $ / $ {\rm^{214}Ra} $	$ 2.54_{-0.27}^{+0.31} $	$ 2.66_{-0.29}^{+0.33} $
		$ {\rm^{218}U} $ / $ {\rm^{212}Rn} $	$ 3.98_{-0.43}^{+0.49} $	$ 4.26_{-0.46}^{+0.52} $
		$ {\rm^{218}U} $ / $ {\rm^{210}Po} $	$ 6.71_{-0.72}^{+0.83} $	$ 7.30_{-0.79}^{+0.90} $

Table 1.  Ratios of $ \delta^2 $ of $ {\rm^{214,216,218}U} $ to those of surrounding nuclei with the same $ N_{p}N_{n} $ systematics under different α-core potentials and different theoretical methods of obtaining the penetration probability.

The values of the quadrupole deformation $ \beta_2 $ of the daughter nuclei of $ {\rm^{214}U} $ and surrounding nuclei are listed in Table 2. These values are obtained from Ref. [37]. Clearly, the value of $ \beta_2 $ varies from positive to negative for different nuclei. We may expect that the $ \delta^{2} $ ratio between them can be influenced by the nuclear deformation, particularly for $ {\rm^{214}U} $ and $ {\rm^{202}Rn} $. The nuclear deformation effect can be considered within the double-folding potential. Moreover, the effects of nuclear deformation on α-decay also have been studied based on the nuclear proximity potential [38] and deformed Woods-Saxon type potential [39−41]. In this work, double-folding potentials without (Pot5) and with (Pot6) the deformation effect are considered. The $ \delta^{2} $ ratios calculated using the WKB approximation under Pot5 and Pot6 are listed in Table 3. Comparing the results obtained under Pot5 and Pot6, we observe that the ratio is reduced when the nuclear deformation effect is considered. The $ \delta^2 $ ratio between $ {\rm^{214}U} $ and $ {\rm^{202}Rn} $ is slightly below 2, implying that the nuclear deformation effect should be considered when studying the trend in the α-decay reduced width.

Parent nuclei	$ Q_{\alpha} $/MeV	Daughter nuclei	$ \beta_{2} $ [37]
$ {\rm^{186}Po} $	8.501	$ {\rm^{182}Pb} $	0.011
$ {\rm^{200}Po} $	5.9816	$ {\rm^{196}Pb} $	0
$ {\rm^{202}Rn} $	6.7738	$ {\rm^{198}Po} $	0.075
$ {\rm^{216}Th} $	8.072	$ {\rm^{212}Ra} $	−0.053
$ {\rm^{214}U} $	8.696	$ {\rm^{210}Th} $	−0.135
$ {\rm^{216}U} $	8.531	$ {\rm^{212}Th} $	−0.094
$ {\rm^{218}U} $	8.775	$ {\rm^{214}Th} $	−0.063

Table 2.  Quadrupole deformation $ \beta_2 $ for daughter nuclei is a theoretical value taken from Ref. [37], whereas the α-decay energy $ Q_{\alpha} $ is taken from experimental data [14, 20].

α−core potential	$ N_{p}N_{n} $	$ \delta^2 $ ratio	WKB method
Pot5	−40	$ {\rm^{214}U} $/$ {\rm^{202}Rn} $	$ 2.22_{-0.90}^{+4.05} $
		$ {\rm^{214}U} $/$ {\rm^{186}Po} $	$ 6.92_{-2.79}^{+12.64} $
	−20	$ {\rm^{216}U} $/$ {\rm^{200}Po} $	$ 2.59_{-0.46}^{+0.73} $
	0	$ {\rm^{218}U} $/$ {\rm^{216}Th} $	$ 1.92_{-0.21}^{+0.24} $
Pot6	−40	$ {\rm^{214}U} $/$ {\rm^{202}Rn} $	$1.78_{-0.72}^{+3.25}$
		$ {\rm^{214}U} $/$ {\rm^{186}Po} $	$ 5.35_{-2.16}^{+9.78} $
	−20	$ {\rm^{216}U} $/$ {\rm^{200}Po} $	$ 2.30_{-0.41}^{+0.64} $
	0	$ {\rm^{218}U} $/$ {\rm^{216}Th} $	$ 1.86_{-0.20}^{+0.23} $

Table 3.  Same as Table 1 but under Pot5 and Pot6.

We note that in Ref. [42], within the generalized liquid drop model, the $ \delta^2 $ ratio between $ {\rm^{214}U} $ and $ {\rm^{202}Rn} $ is smaller than 2. In Ref. [36], by considering a shell-dependent α-core potential, the $ \delta^2 $ ratio between $ {\rm^{214}U} $ and $ {\rm^{202}Rn} $ is found to be larger than 10. With other phenomenological α-core potentials, this ratio is typically smaller than 3, as listed in Table 1. The present analysis demonstrates that the influences of the α-core potential and nuclear deformation should be discussed when studying the α-decay reduced width. Studies (e.g., Refs. [15, 16]) on the α-preformation probability have shown that shell closures in both parent and daughter nuclei are very important; consequently, the reduced width is also closely related to shell closures. More detailed studies on the effects of shell closures are required to fully understand the α-decay reduced width in the $ N_{p}N_{n} $ systematics.

Using the WKB approximation and TM approach to obtain the penetration probability, we revisit the α-decay reduced width $ \delta^{2} $ of $ {\rm^{214,216}U} $ and their surrounding nuclei under four different phenomenological α-core potentials, as well as spherical and deformed double-folding potentials. We observe that $ \delta^{2} $ is very sensitive to the α-core potential; its value can vary by almost three orders of magnitude when different α-core potential is considered. The values of $ \delta^{2} $ obtained using the WKB approximation are about 20%−40% larger than those obtained with the TM approach because of the underestimation of penetration probability in the WKB approximation. This underestimation is found to be related to the choice of α-core potential, as well as the mass and charge number of parent nuclei. However, this underestimation only have a small effect on the ratio of the $ \delta^{2} $ of uranium isotopes to the surrounding nuclei under $ N_{p}N_{n} $ systematics. With spherical form for the α-core potentials, the $ \delta^{2} $ ratio is about twice as large as those of the surrounding nuclei. With the deformed double-folding potential, the ratio between $ \delta^{2} $ of $ {\rm^{214}U} $ and those of the surrounding nuclei is found slightly below 2. This present work indicates that the influences of the α-core potential and nuclear deformation should be discussed when studying the α-decay reduced width in the $ N_{p}N_{n} $ systematics.

The authors are grateful to the C3S2 computing center in Huzhou University for numerical calculation support. We acknowledge the fruitful discussions with Drs Qian Yibin, Wan Niu, Deng Jungang, and Zhang Zhiyuan.