Invariant Mass Spectroscopy of ¹⁵C

Nuclear clustering is a fascinating and important phenomenon in nuclear physics, where owing to many-body correlations, nucleons (protons and neutrons) within an atomic nucleus bunch up to form substructures or clusters. These clusters can exhibit properties similar to smaller nuclei, such as alpha particles, $ ^{12} {{\rm{C}}}$ or other light nuclei. Due to their strong binding energy, alpha particles are the most common building blocks of nuclear clustering. In fact, as early as 1931, Gamow proposed that 4n-nuclei such as $ ^8 {{\rm{Be}}}$, $ ^{12} {{\rm{C}}}$ and $ ^{16} {\rm{O}}$ were composed of alpha particles [1]. Such model of nucleus with alpha particles as the main constituents remained the mainstream [2, 3] for a long time after the discovery of neutron in 1932 [4], and notwithstanding the early emergence of the model for independent particle motion [5]. It was not until 1949 following the development of the more robust nuclear shell model [6, 7] that the independent-particle picture became the dominant nuclear model.

The prediction [8] and discovery [9] in the 1950s of the famous resonance Hoyle state – a triple-alpha clustering state – in $ ^{12} {{\rm{C}}}$ at 7.65 MeV reaffirmed the importance of alpha clustering. The introduction of the Ikeda diagram [10] a decade later provides useful guides for observation of cluster states in excited states of light nuclei, especially near their alpha-decay threshold. Many experiments have been carried out ever since to search for alpha-clustering states in stable [11−14] as well as in exotic nuclei, where excessive valence neutron(s) or proton(s) may lead to the formation of molecular states [15−23]. Because of the subtle interplay between many-body correlations and single-particle behavior which gives rise to competition between alpha-clustering and single-particle structure, it remains a challenge to experimentally identify the clustering states from a multitude of single-particle states.

Invariant mass spectroscopy is a powerful tool for studying nuclear resonance states in nuclear physics, especially under inverse kinematics condition using radioactive ion beams [20−22, 24−28]. These states are excited states of nuclei that typically decay rapidly (on the order of $ 10^{-22} $ to $ 10^{-15} $ seconds) by emitting particles such as alpha or gamma rays. By analyzing the invariant mass of the decay products, it is possible to determine the properties of these resonances, such as their mass, width and decay modes, providing insights on nuclear structure and the underlying nuclear forces, nuclear reaction dynamics, as well as the stellar nucleosynthesis. For a resonance state that decay by emitting a nuclear cluster such as alpha particle, measurements of the decay alpha particle and the residual nucleus provide access to the resonance parameters.

An ideal reaction for studying nuclear resonance states, particularly those involving clustering or other exotic structures, typically consists of two key steps: population of resonance states and resonant decay. However, in reality, prompt particles from direct breakup are always present, giving rise to physical backgrounds. Therefore, accurate estimates of the background from non-resonant components are essential to extract resonance parameters from invariant-mass spectra. Presently, there are no established methods to evaluate such contributions. One of the conventional methods to estimate the contribution is the event-mixing method. However, it is not at a satisfactory level to describe the spectrum. The result with such event mixing usually overestimates the non-resonant background, especially near the charged particle threshold, due to the difficulty of treating the long-range Coulomb final-state interactions, which are not included in the event-mixing analysis. To address this issue, a phenomenological weighted event-mixing method has recently been proposed [29].

In this article, we report on an invariant-mass spectroscopy employing the $ ^{208} {\rm{Pb}}$($ ^{15} {{\rm{C}}}$, $ ^{15} {{\rm{C}}}^*$ $ \rightarrow $ $ ^{11} {{\rm{Be}}}$+$ ^4 {{\rm{He}}}$) reaction to search for resonance state(s) in $ ^{15} {{\rm{C}}}$ at excitation energy above the alpha-decay threshold of 12.73 MeV [30]. Most of the studies of clustering effect in neutron-rich carbon isotopes have focused on the neighboring $ ^{14,16} {{\rm{C}}}$. Several spectroscopy studies on $ ^{15} {{\rm{C}}}$ have been reported [31−33] but the discussions focus mostly on single-particle resonances. In the present work, to address the issue of overestimation of the non-resonant background, we refer to Gamow theory of alpha decay [1] and introduce a new method that aims at a robust treatment of the Coulomb effect. Combined with the phenomenological weighted event-mixing method [29], our analysis results suggest possible resonant states in $ ^{15} {{\rm{C}}}$. A brief description of the experiment is given in Sec. 2. Next, detailed descriptions on the analysis procedures, which include non-resonant background estimation, spectrum fitting and a brief discussion on the results are given in Sec. 3. Finally, a summary is given in Sec. 4.

The experiment was performed at the Radioactive Ion Beam Line of the Heavy Ion Research Facility in Lanzhou (HIRFL-RIBLL)[34, 35]. Secondary beams were produced via the projectile fragmentation reaction using a 60-MeV/nucleon $ ^{18}{\rm{O}} $ primary beam incident on a 4.5-mm-thick $ ^{9}{{\rm{Be}}} $ target. Following subsequent magnetic-rigidity selection and purification by RIBLL, a secondary beam of $ ^{15} {{\rm{C}}}$ at about 27.9 MeV/nucleon with an average intensity of approximately 2.0$ \times 10^4 $ particles per second (pps) and a purity of about 81% was obtained. The beam particle identification was achieved by combining the magnetic rigidity ($ B_\rho $), time-of-flight (TOF) and energy loss ($ \Delta E $) on an event by event basis. TOF was provided by two 50-µm thick plastic scintillation detectors installed at the second (T1) and fourth (T2) achromatic focal planes of RIBLL, which are about 17 m apart. A large area silicon detector was installed at around the T2 focal plane to measure the energy loss. Three position-sensitive parallel plate avalanche counters (PPACs), with a typical position resolution of around 1 mm (FWHM), located upstream of the target, were used to track incident beam particles.

The $ ^{15} {{\rm{C}}}$ beam bombarded a 230-mg/cm²-thick $ ^{208} {\rm{Pb}}$ target with a size of 30 mm in diameter to induce inelastic scattering populating unbound cluster states in $ ^{15} {{\rm{C}}}$, namely $ ^{208} {\rm{Pb}}$($ ^{15} {{\rm{C}}}$, $ ^{15} {{\rm{C}}}$$ ^\star \rightarrow $ $ ^{11} {{\rm{Be}}}$ + $ ^4 {{\rm{He}}}$). A zero-degree telescope named T0, which forms one part of a larger detection system described in detail in Ref.[22, 36, 37], was employed to coincidentally detect the charged reaction products. The telescope was placed symmetrically with respect to the beam axis at a distance of about 170 mm downstream from the target, thus providing an approximate angular coverage range of $ \pm $10.7° as seen from the center of the $ ^{208} {\rm{Pb}}$ target. The T0 telescope consists of three 1000-µm-thick double-sided silicon strip detectors (DSSDs), three 1000-µm-thick segmented single-readout silicon detectors (SSDs), and four CsI(Tl) crystals arranged in a 2$ \times $2 matrix, and readout by photo-diodes. The DSSDs and SSDs have an active area of 64$ \times $64 mm²; each side of DSSD has 32 strips with a strip width of about 2 mm. In the present experiment, the charged reaction products, namely $ ^{11} {{\rm{Be}}}$ and alpha did not reach the CsI(Tl) detectors; the $ ^{11} {{\rm{Be}}}$ nuclei stopped in one of the DSSDs, while the alpha particles either stopped in the DSSDs, or penetrated the DSSDs, depositing a portion of their kinetic energies, before being stopped in one of the SSDs.

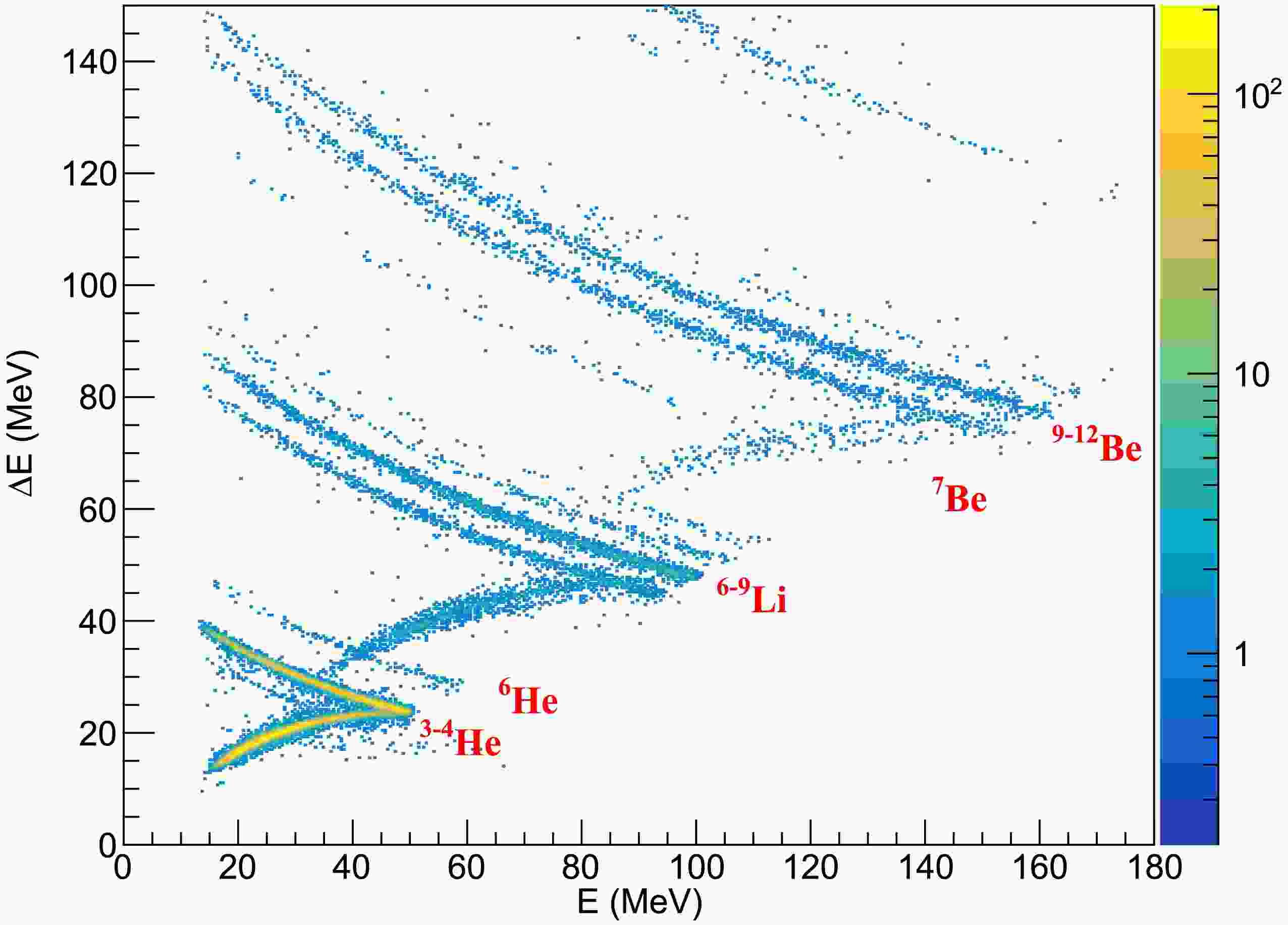
Energy calibration of the DSSDs was performed through a combination of normalization and calibration procedures. First, the signals of different strips on both sides were normalized using the method described in Ref.[38]. The absolute energy calibration was realized by combining the measurement with an $ ^{241} {\rm{Am}}$ standard alpha particle source, and the threshold energies of nuclei penetrating each DSSD. For SSD, only absolute energy calibration was performed. The average relative energy resolution of silicon detectors was around 1% at 5.486-MeV alpha-particle energy, obtained with the $ ^{241} {\rm{Am}}$ source. For the energy calibration of CsI(Tl) crystals, a linear formula for He isotopes was used, which is a good approximation as discussed in Refs. [39, 20, 21]. Un-reacted $ ^{15} {{\rm{C}}}$ Beam particles, which has several orders higher intensity than the reaction products, penetrated the first layer of the T0 telescope, and were stopped in the second layer of the DSSDs. To exclude accidentally coincident events due to these beam particles, timing signals of the DSSD strips on the both sides were considered. The horizontal (X) and vertical (Y) positions of two coincident particles on each DSSD were determined by matching their energy signals at the front and rear sides. Reaction particles produced by $ ^{15} {{\rm{C}}}$ beam impinging on DSSDs were mostly removed by considering correlating hits with consistent positions on the target and at least two adjacent DSSDs [20−22]. After implementing the above-mentioned analysis procedures, a clean particle-identification (PID) plot is obtained based on the energy loss versus residual energy ($ \Delta E $-E) technique, as shown in Fig. 1. Assuming that the reaction occurred at the center of the target, the kinetic energies of the reaction products, namely $ ^{11} {{\rm{Be}}}$ and alpha, right after the reaction were determined taking into account their energy losses in the target. The momenta of $ ^{11} {{\rm{Be}}}$ and alpha were then determined considering their scattering angles.

Figure 1.  (color online) $\Delta E$-E spectrum. $\Delta E$ and E represent the energy losses of charged particles in the first and second layers of the DSSDs of the T0 telescope, respectively.

The excitation energy spectrum of $ ^{15} {{\rm{C}}}$ was reconstructed using the momenta and rest masses of the coincidentally detected $ ^{11} {{\rm{Be}}}$ and alpha employing the invariant mass method. Since $ ^4 {{\rm{He}}}$ does not have bound excited state, while $ ^{11} {{\rm{Be}}}$ has only one with an excitation energy of 0.32 MeV, $ ^{11} {{\rm{Be}}}$ may be in its ground or bound excited state. Because the invariant-mass resolution of the present experiment does not allow separation of the ground and the excited state in $ ^{11} {{\rm{Be}}}$, in the following analysis, we assume that all $ ^{11} {{\rm{Be}}}$ particles measured coincidently with $ ^4 {{\rm{He}}}$ were in ground state.

For cluster decay following nuclear reaction $ ^{208} {\rm{Pb}}$($ ^{15} {{\rm{C}}}$, $ ^{15} {{\rm{C}}}^*$ → $ ^{11} {{\rm{Be}}}$ + $ ^{4} {{\rm{He}}}$), the excitation energy of $ ^{15} {{\rm{C}}}^*$ is expressed as follows:

where $ m_{^{15}{\rm{C}}} $ is the rest mass of $ ^{15} {{\rm{C}}}$, and $ m_{^{15}{\rm{C}}^*} $, $ E_{^{15}{\rm{C}}^*} $ and $ P_{^{15}{\rm{C}}^*} $ are the rest mass, total energy and momentum of the resonant nucleus $ ^{15} {{\rm{C}}}$$ ^* $, respectively. Here, we have adopted the natural unit for the speed of light, i.e. $ c = 1 $. According to the conservation of energy and momentum during the decay process, $ E_{^{15}{\rm{C}}^*} $ and $ P_{^{15}{\rm{C}}^*} $ can be calculated by:

For each breakup fragment, the total energy, momentum and kinetic energy are related as follows:

where i represents $ ^{11} {{\rm{Be}}}$ or $ ^{4} {{\rm{He}}}$, and $ P_i $, $ T_i $ represents their momentum and kinetic energy, respectively. Combining Eqs. (2), (3), (4), (5) and (6), $ m_{^{15}{\rm{C}}^*} $ can be deduced by:

The kinetic energies and opening angle (θ) of the two fragments produced from $ ^{15}{\rm{C}}^* $ were measured in the experiment, while the ground-state rest masses are taken from Ref. [40]. Hence, $ m_{^{15}{\rm{C}}^*} $ can be reconstructed using experimental kinetic energies ($ T_{^{11}{\rm{Be}}} $ and $ T_{^{4}{\rm{He}}} $) and angular information of the two coincident products. The excitation energy can also be expressed as:

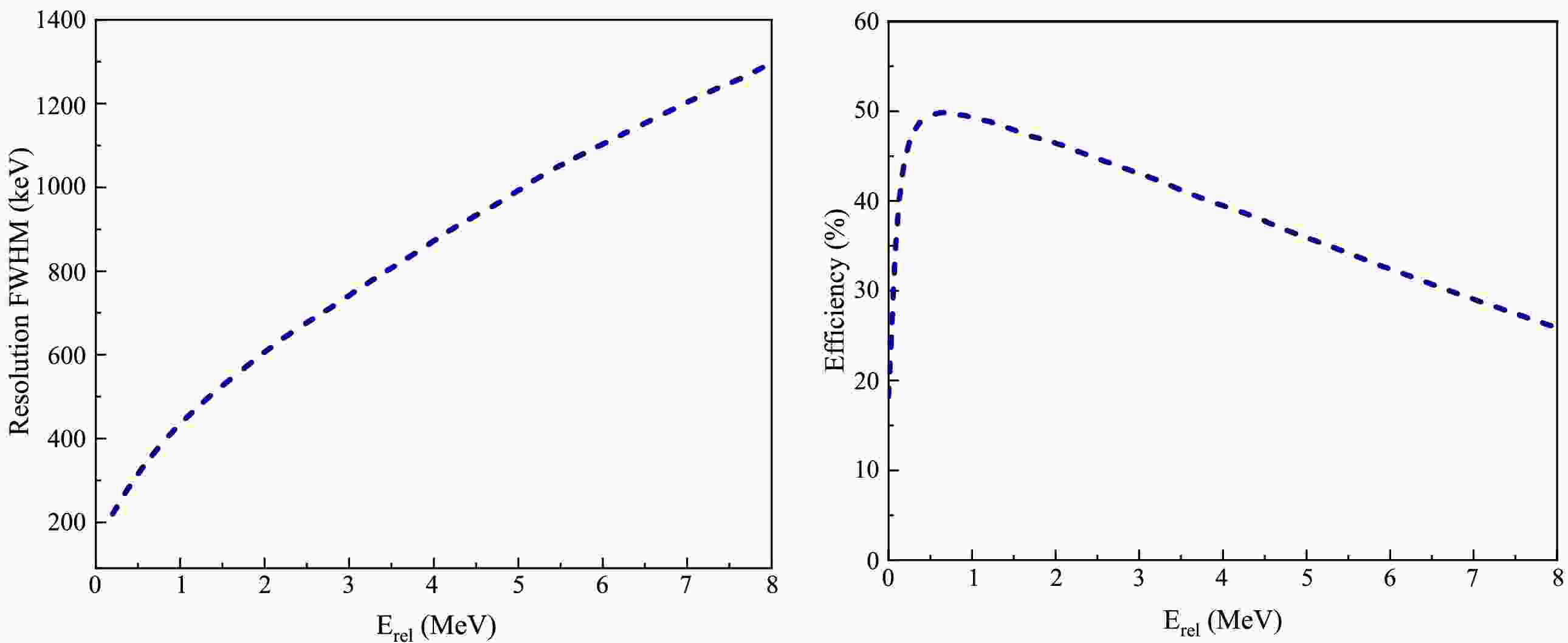
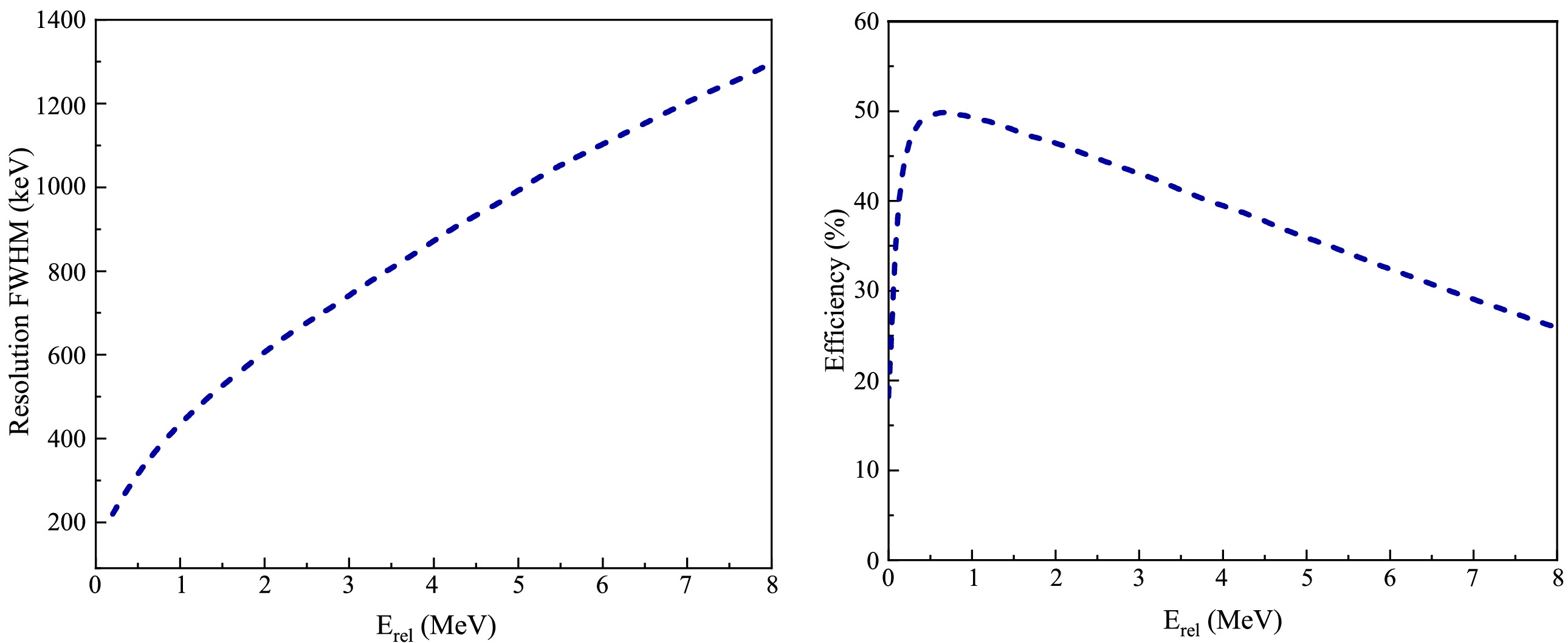
where $ E_{\rm{th}} $ is the threshold energy of the decay.

Monte-Carlo simulation was conducted to evaluate the detection efficiency and invariant-mass resolution, taking into account (i) the geometry and performances of the detectors, which include the active area of the DSSDs, strip width and energy resolution; (ii) the energy and angular spread of the beam; and (iii) the reaction position and energy loss of the beam and fragments in the target. Figure 2 shows detection resolution and efficiency as functions of the relative energy for $ ^{15} {{\rm{C}}}$ decaying into $ ^{11} {{\rm{Be}}}$(g.s.) + $ ^{4} {{\rm{He}}}$.

Figure 2.  (color online) Simulated detection resolution (left) and efficiency (right) as functions of the relative energy for ¹⁵C decaying into ¹¹Be(g.s.) + ⁴He, respectively.

As in most invariant-mass spectroscopy involving unbound resonance states, reconstructed spectra are likely to include non-resonant contributions in addition to resonant states. To estimate the non-resonant contribution, we applied the event-mixing method. Following the prescription proposed in Ref [29], we employed the following functional form to estimate the reduction factor:

where $ c_1 $ and $ c_2 $ are parameters to determine each final state, and $ E_R $ is the relative energy for the two-cluster system. For example, $ c_1 = -38.4 $ MeV and $ c_2 $ = +1.19 MeV were used for the p+$ ^8 {\rm{B}}$ channel [29].

In this paper, we introduce another method that focuses on a robust treatment of the Coulomb effect, which is usually applied to describe alpha-decay processes [1]. The penetrability P of alpha particles in a Coulomb barrier is formulated as follows:

where $ Z_1 $ and $ Z_2 $ are the atomic number of the two clusters, µ and E are the reduced mass of the clusters and the total decay energy of a resonance, respectively. R is the radius of the nuclear potential, and b is the outer radius of the Coulomb barrier at energy E. The penetrability is a function of the total decay energy, and is introduced as a reduction factor in the event-mixing method. Since $ E_R $ is equal to E in the present case, $ P(E) $ is taken as equal to 1+R(E) by definition here. It is analytically solved using the sharp-cut Coulomb barrier method, namely

The above $ B_{\rm{C}} $ is the energy height of the Coulomb barrier at the radius R. Conventionally, R is taken as the sum of radii of the two clusters:

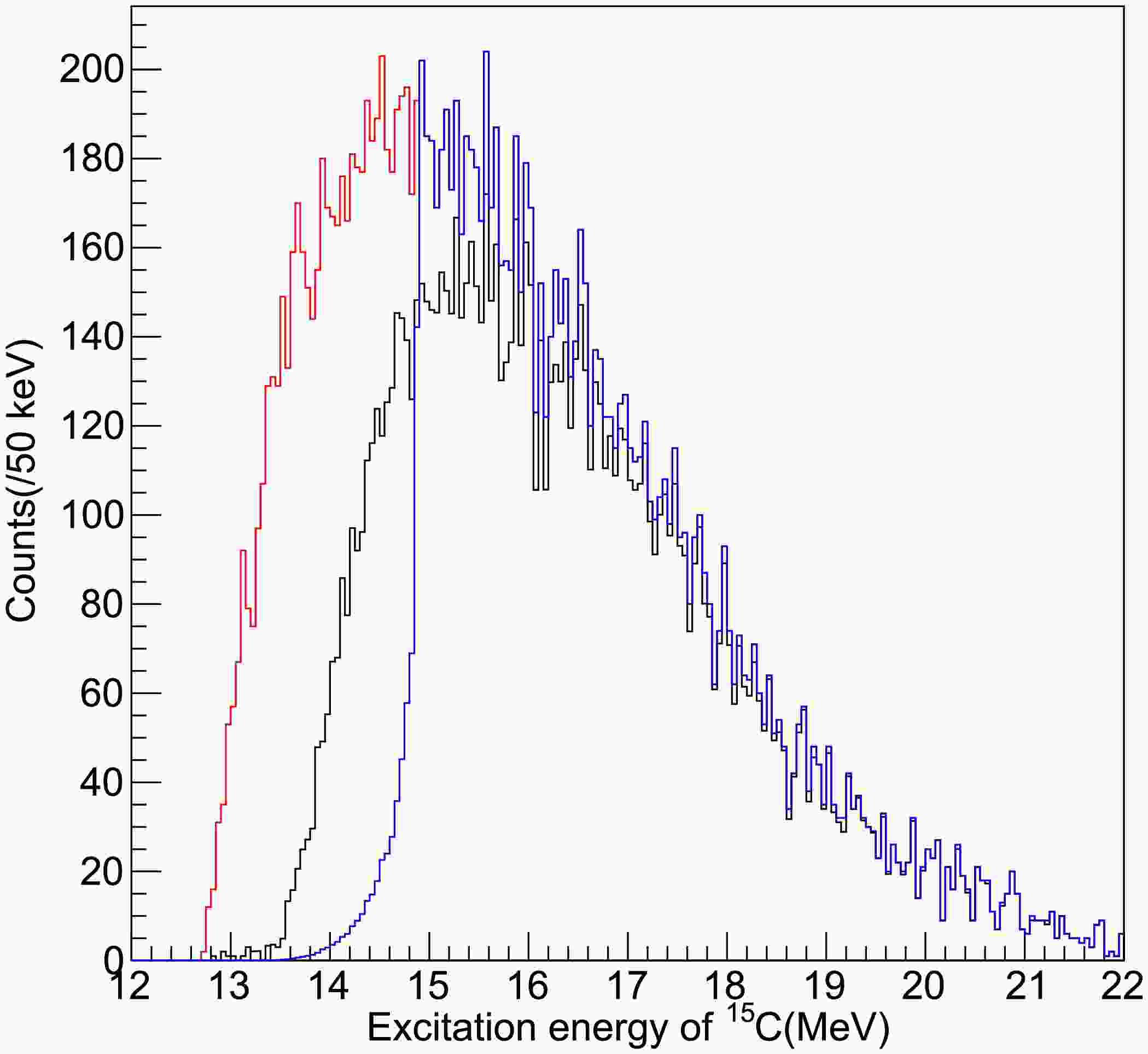
where $ A_1 $ = 11, $ A_2 $ = 4 and $ r_0 $ typically takes a value of 1.40 fm. Although the event-mixing method can explain the spectrum at larger decay-energy region, we found that the penetrability with the conventional R with $ r_0 = $1.40 fm underestimated the spectrum near the threshold. Hence, we introduce additional degrees of freedom to reproduce the shape of the spectrum. The parameter R is randomly determined assuming a normal distribution with its mean value and width as the new free parameters. Typically, around 50 % larger mean value than the usual radius R is necessary. In the present $ ^4 {{\rm{He}}}$+$ ^{11} {{\rm{Be}}}$ channel, a 2.0 fm larger value compared to the conventional R and a width of 3.5 fm (in σ) were adopted. In Fig. 3, we compare the mixed-event distribution without (red histogram) and with reduction correction obtained with different methods. The blue and black histograms are the weighted mixed events corrected with the sharp-cut and effective Coulomb barrier methods, respectively.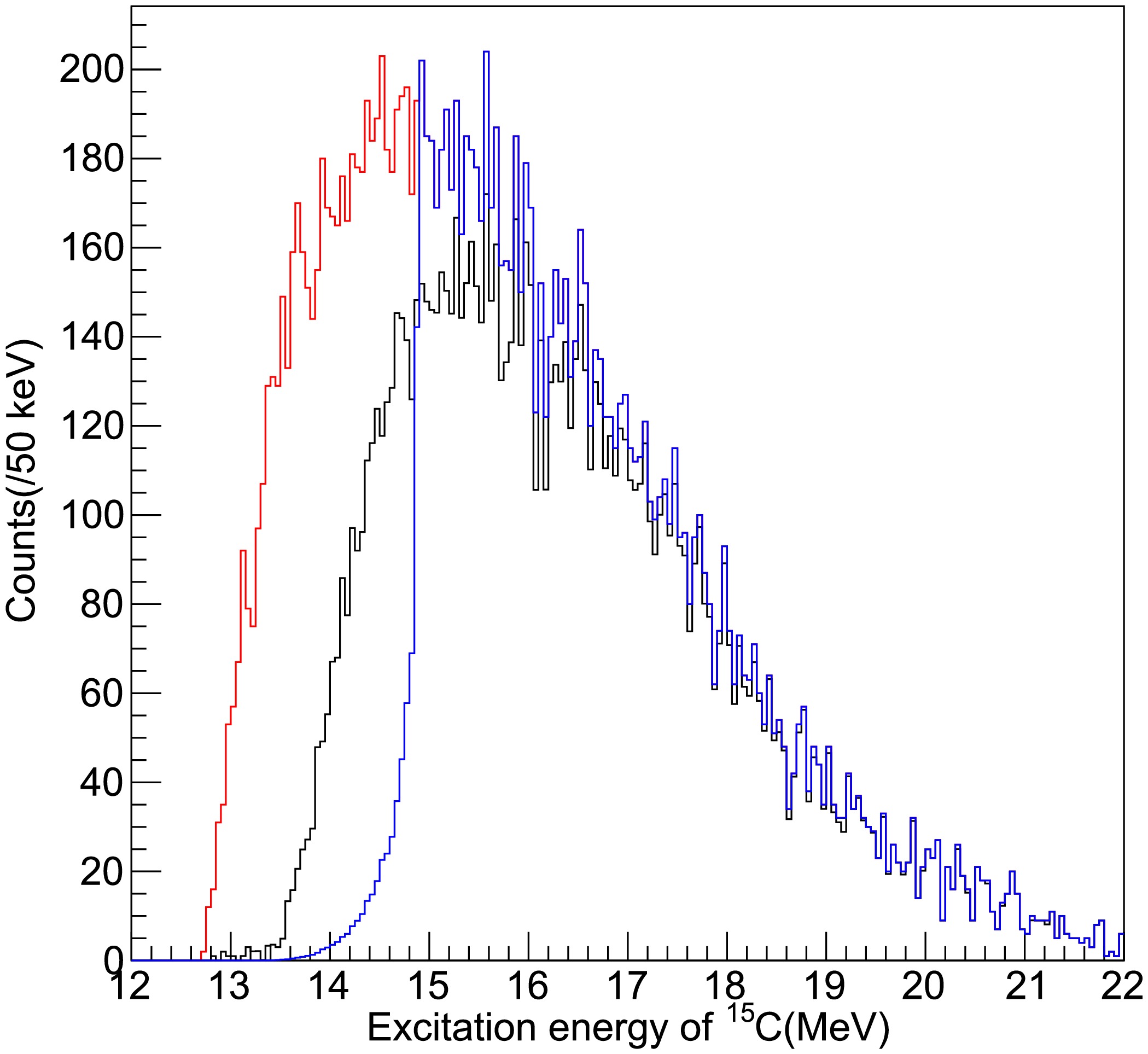

Figure 3.  (color online) Excitation energy spectrum with the background distribution produced by the event-mixing analysis coupled to the reduction factors deduced using two different methods. The red histogram represents the mixed-event distribution without any reduction correction, while the blue and black histograms are the weighted mixed events corrected with the sharp-cut and effective Coulomb barrier methods, respectively.

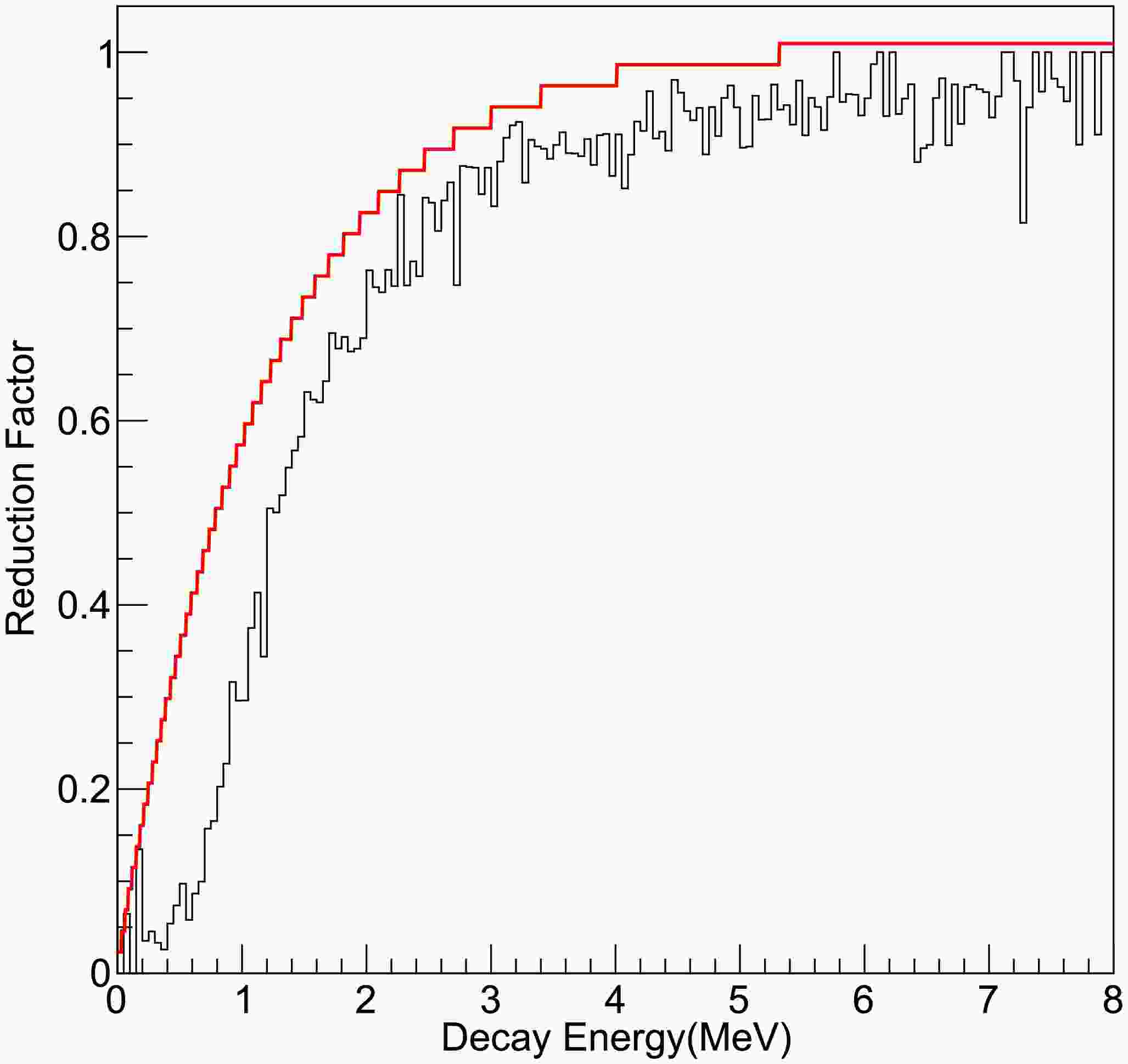
Figure 4 shows the reduction factor as a function of the total decay energy obtained with the present effective Coulomb barrier method. For comparison, the reduction factor obtained with the phenomenological method in Ref. [29] but for the case of p+$ ^8 {\rm{B}}$ is also shown as the red histogram. Both distributions show a similar trend moving towards the particle threshold, starting to deviate at around a few MeV above the threshold, and gradually decreases to zero. Since the statistics are very limited in the present work, it is challenging to follow the recipes in Ref. [29] to determine the parameters $ c_1 $ and $ c_2 $ using the present data. For practical reason, we analyzed the “1+R” correlation function data for the $ ^3 {{\rm{He}}}$+ $ ^8 {\rm{B}}$ channel in Ref. [29] whose Coulomb barrier is close to the present $ ^4 {{\rm{He}}}$+$ ^{11} {{\rm{Be}}}$ channel, and obtained $ c_1 $ = +2.017 MeV and $ c_2 $ = +0.482 MeV for the reduction factor. The reduction factor was then adopted to estimate the non-resonant background.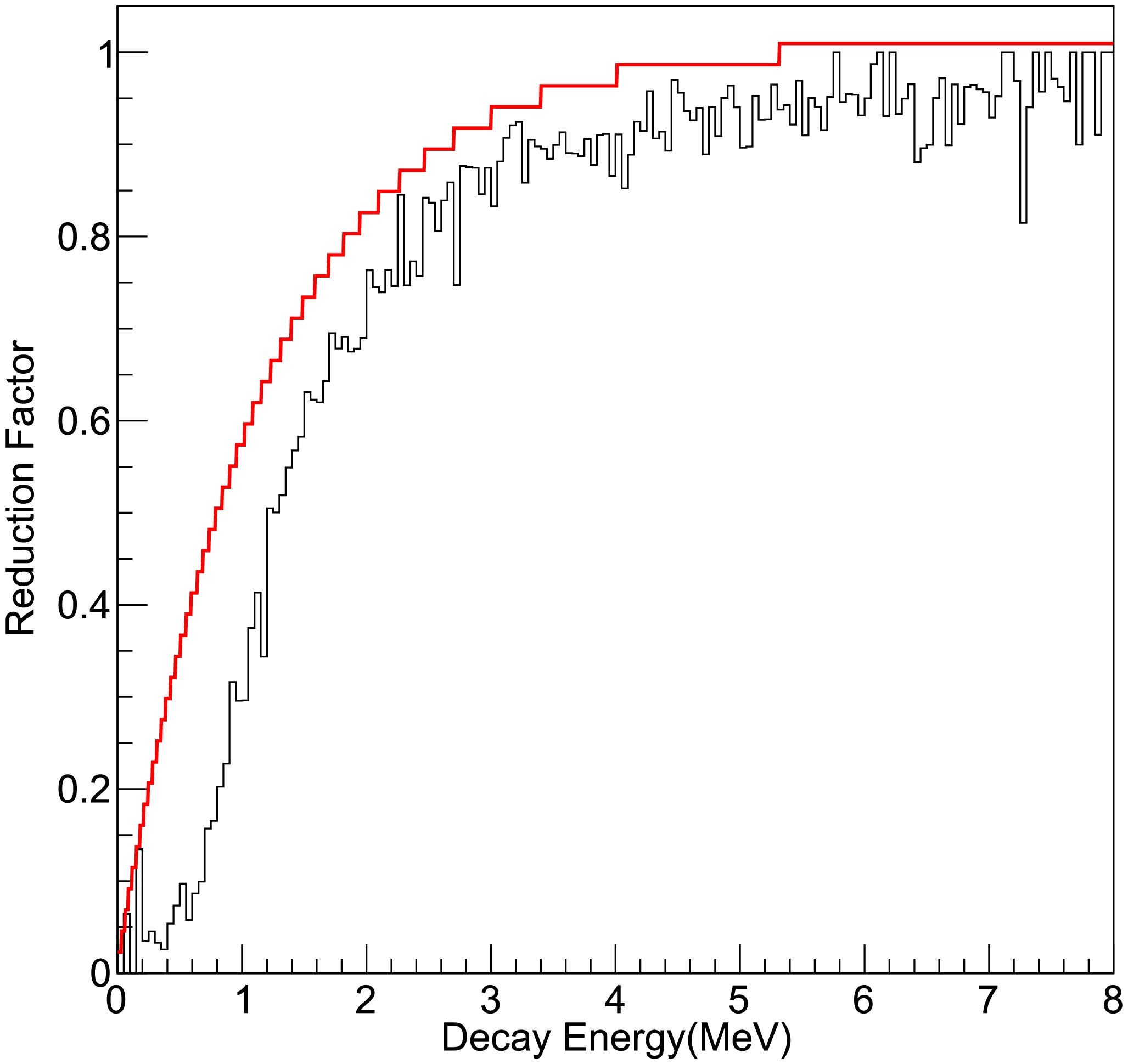

Figure 4.  (color online) Reduction factor as a function of the total decay energy. The black and red histograms represent the results of the present method for ⁴He+ ¹¹Be and the method from Ref [29] for the p+ ⁸B channel, respectively. Both distributions show a similar trend moving towards the particle threshold, starting to deviate at around a few MeV above the threshold, and gradually decreases to zero.

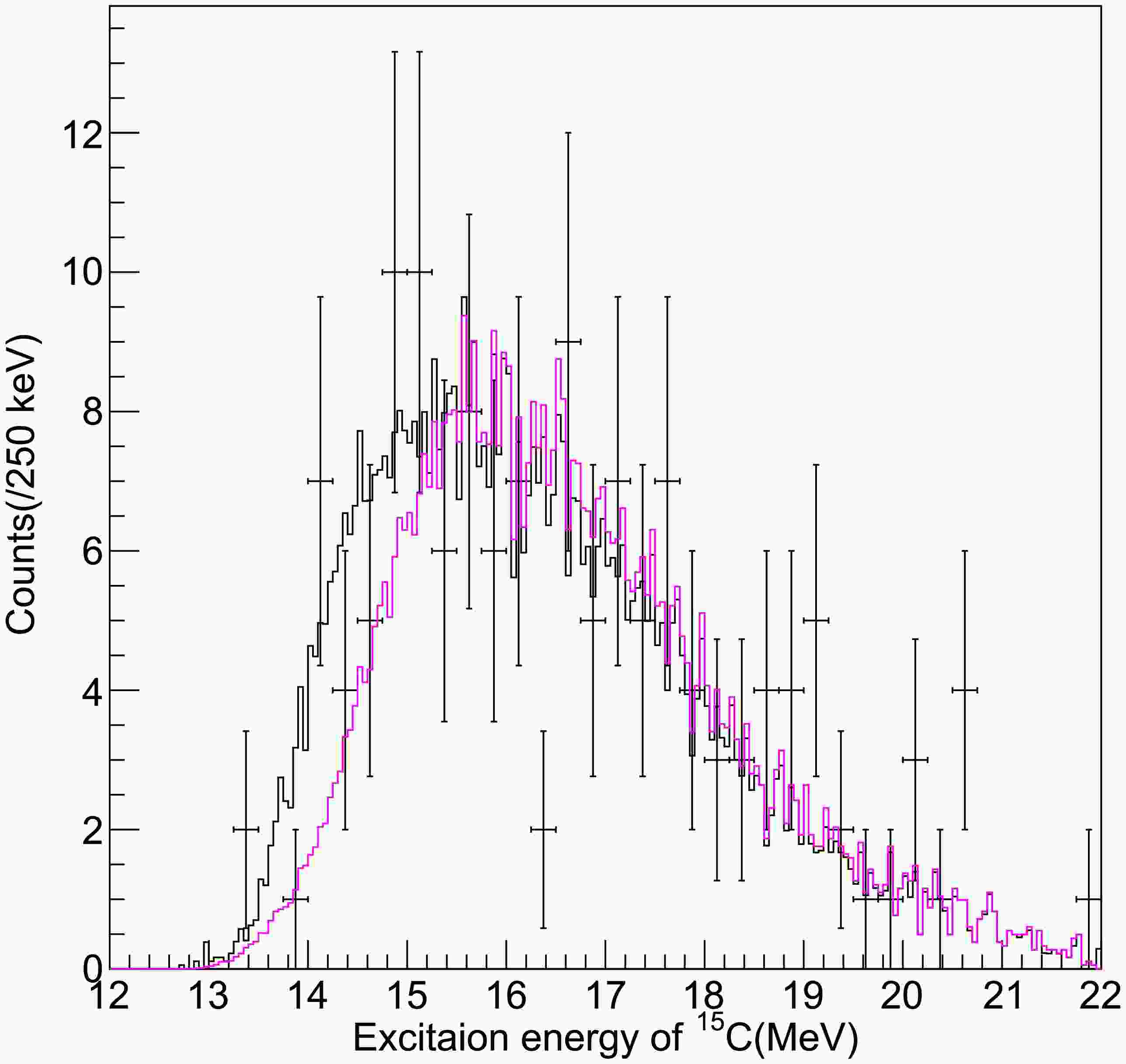
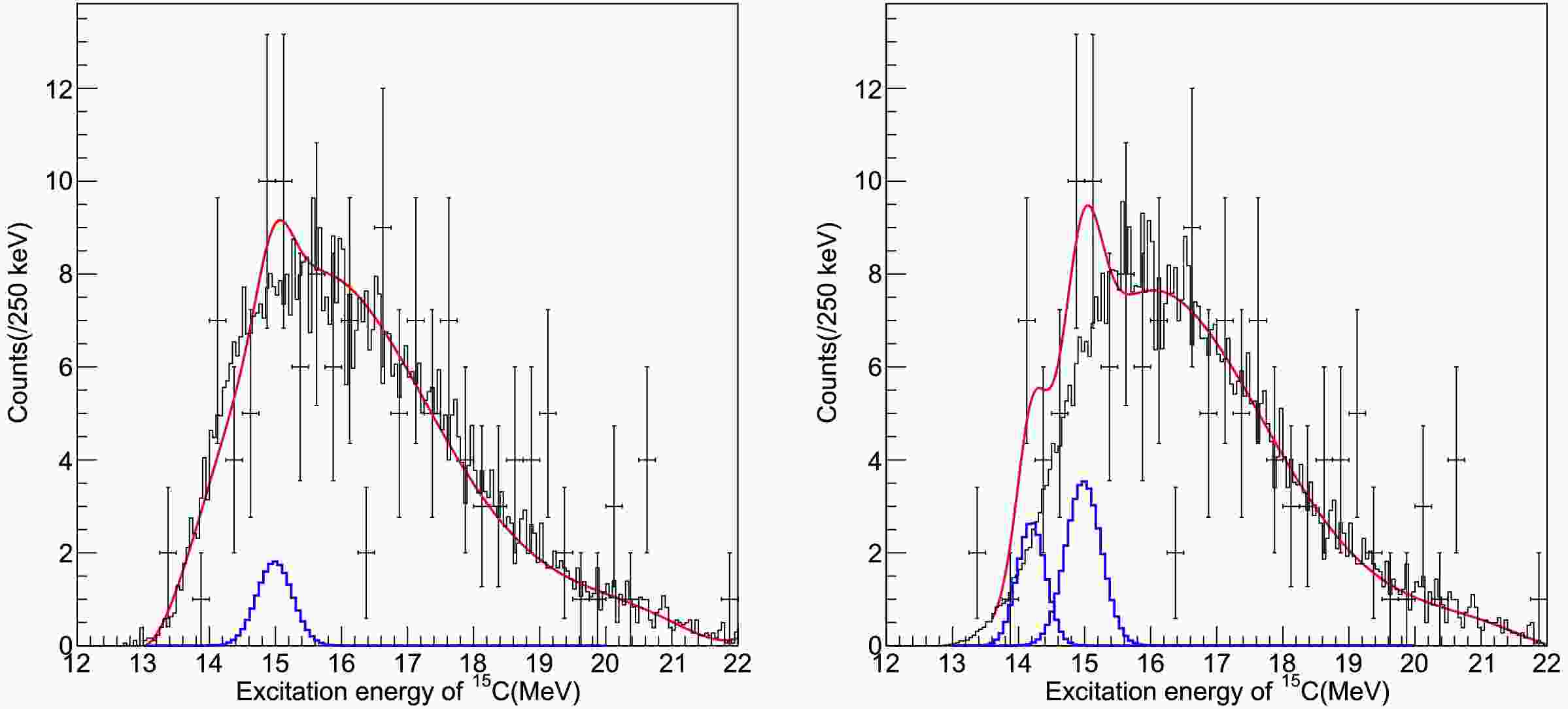
We describe the background distribution using the event-mixing data obtained in the present work coupled with the decay-energy dependent reduction factor determined with two different methods. For simplicity, we fitted the event-mixing data using a sixth-order polynomial function. The amplitude of the distributions was then determined to reproduce the events at the excitation energy above 17 MeV in the excitation-energy spectrum. The resonance state can be described by a convoluted function of a Breit-Wigner distribution with the decay energy-dependent experimental resolution function in the Gaussian form. This was achieved by using the Voigt function provided by the ROOT library [?]. Since the typical resolution at 2 MeV decay energy is 250 keV in σ, and is much larger than that expected for a resonance state, the spectrum shape of the resonance states is expected to be nearly Gaussian, and their widths are mainly determined by the experimental resolution described above. Figure 5 shows the excitation energy spectrum, along with the weighted event-mixing background distributions obtained with the above-mentioned two different methods. The black background, obtained with the effective Coulomb barrier method reproduces the experimental data well with a reduced chi-square $ \chi^2 $/ndf = 43.3/35. The second type of background – the magenta histogram, obtained with the mixed events incorporating the reduction factor (Eq. 9) with $ c_1 $ = +2.017 MeV and $ c_2 $ = +0.482 MeV, shows a reduction in the lower energy region. We note that a small energy shift of around 100 keV has been introduced to the distribution to compensate for the difference in the Coulomb barrier between the $ ^4 {{\rm{He}}}$+$ ^{11} {{\rm{Be}}}$ and $ ^3 {{\rm{He}}}$+$ ^{8} {\rm{B}}$ channels. For convenience, we referred to the weighted event-mixing background obtained with this latter method as “phenomenologically-corrected background” (labelled simply as “bg2”); the background obtained using the effective Coulomb barrier is referred to as “Coulomb-corrected background”, (labelled simply as “bg1”) hereinafter.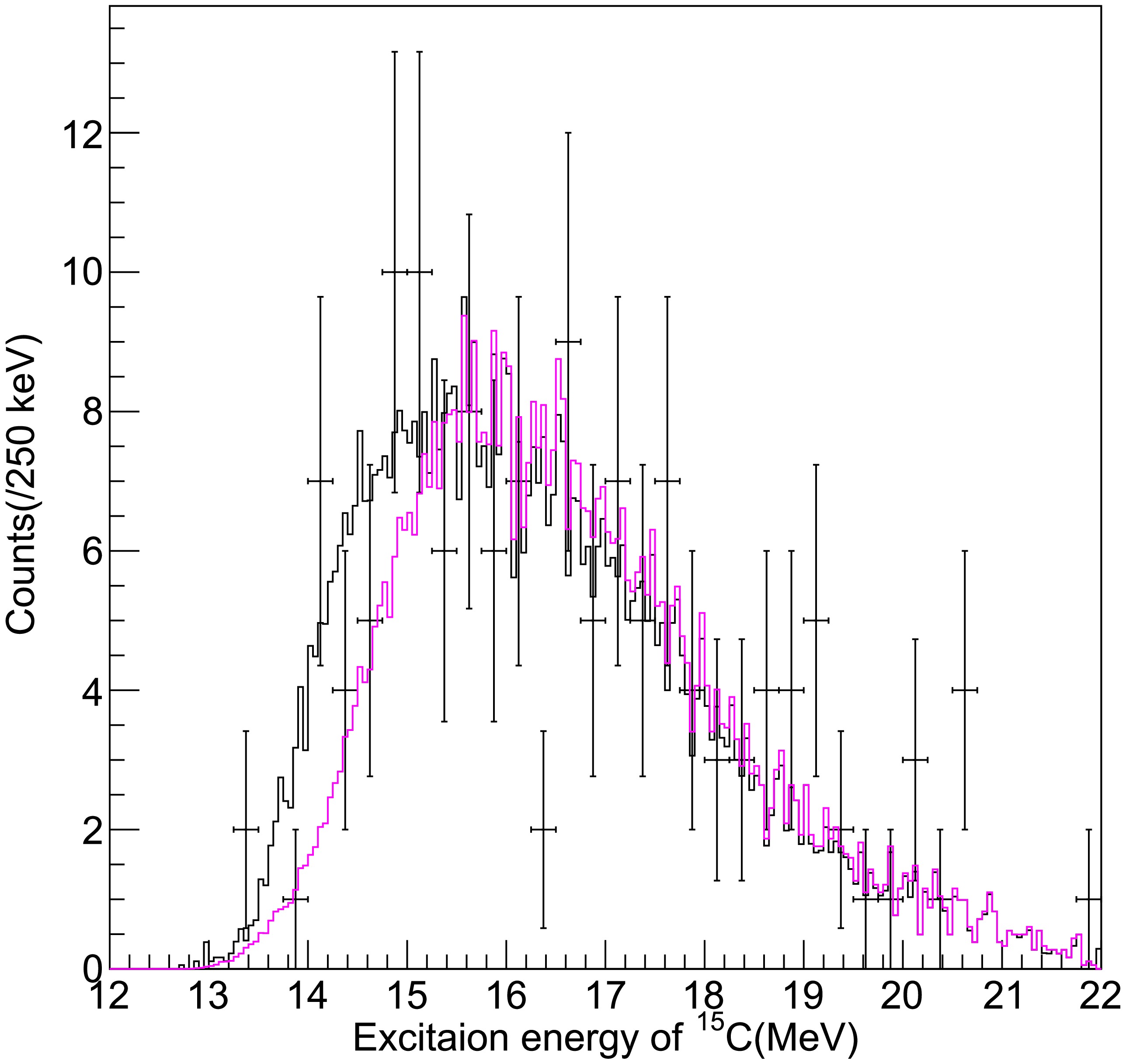

Figure 5.  (color online) Excitation energy spectrum with the background distribution produced by the event-mixing analysis coupled to the reduction factors deduced using two different methods. The black histogram represents the background obtained with the effective Coulomb barrier method, while the magenta histogram is the background obtained with the mixed events incorporating the reduction factor given by Eq. 9 with $c_1$=+2.017 MeV and $c_2$=+0.482 MeV.

A few events can be seen on top of the estimated non-resonant background, especially. We consider possible resonance state(s) with signal function(s) of the Voigt form. Figure 6 shows the fitting results (red lines) with possible resonance state candidate(s) (blue lines) plus the two different backgrounds (black histograms). Although the fit with the Coulomb-corrected background describes the experimental data well, a resonance state candidate is suggested around 15 MeV. Fitting with the phenomenologically-corrected background, on the other hand, implies possible observation of two resonance state candidates.

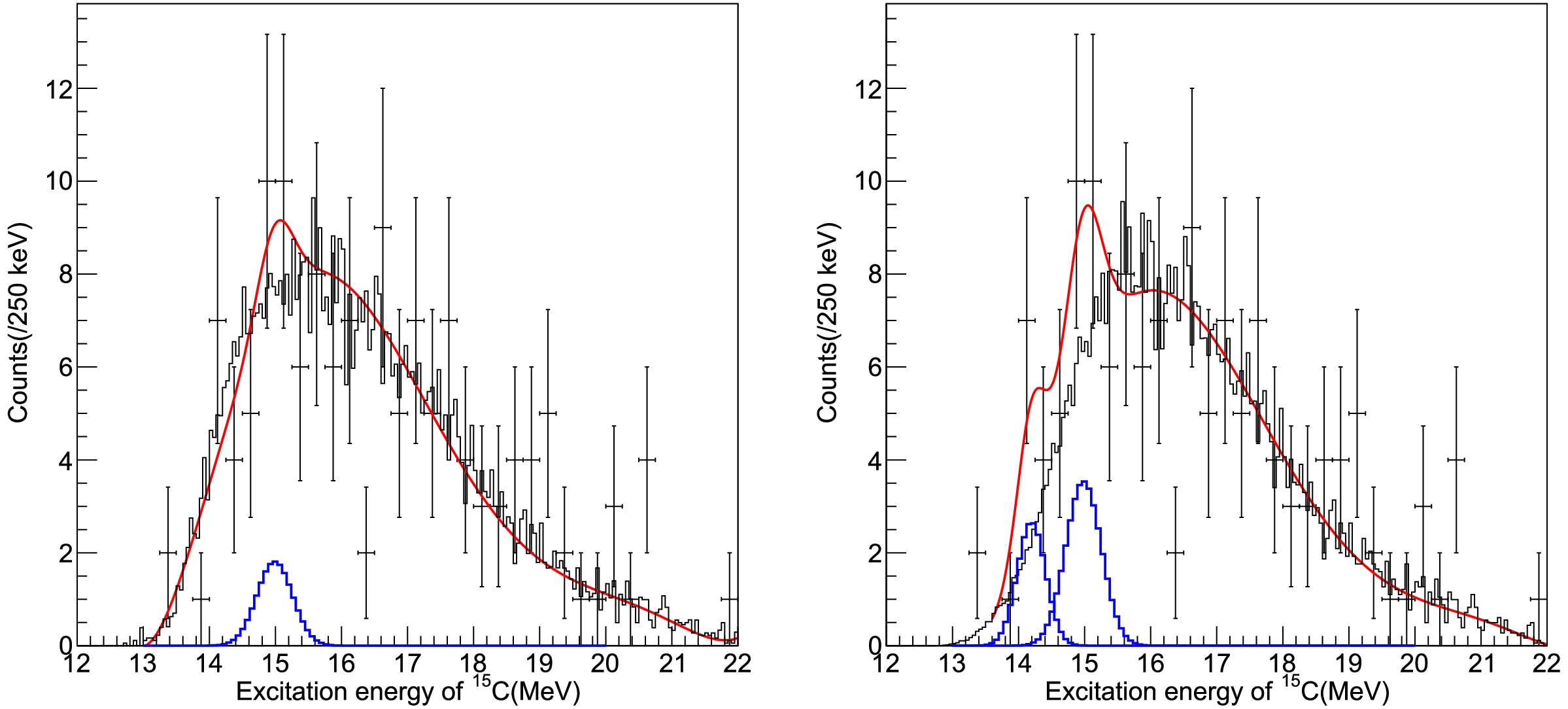
Figure 6.  (color online) Results of spectral fitting (red lines) assuming one (left) and two (right) resonance state(s) (blue lines) plus the event-mixing backgrounds (black histograms) obtained with the effective Coulomb barrier method and the method proposed in Ref. [29], respectively. The experimental resolutions have been assumed for the resonance state candidates.

Further optimization was made applying the unbinned maximum likelihood analysis. We define the total probability density function ($ pdf $) consisting of several sub-$ pdf $'s. The log-likelihood function is defined as:

where $ N_f = 3 $ correspond to two signal functions and one background distribution considered in the analysis, and n represents the number of data. $ \vec{p}_j $'s are arrays with dimensions equal to the number of parameters for the respective functions $ f_j $, i.e. dimension = 2 for the signal functions and 7 for the background, although some of the background parameters were fixed during the analysis process. All of the parameters $ \vec{p}_j $ and the integral of each sub-$ pdf $ $ \mu_j $ were optimized to maximize the log-likelihood function $ lnL $. The values extracted from the present data are summarized in Table 1. Small differences in the table were observed, potentially due to the binning bias. It is difficult to avoid the effect of the finite binning size due to very limited statistics.

		$\mu_1$	$E_1$	$\Gamma_1$	$\mu_2$	$E_2$	$\Gamma_2$
bg1	binned	4.9(54)	14.99(13)	<0.01	–	–	–
	unbinned	18.3(61)	15.06(15)	<0.01	–	–	–
bg2	binned	9.8(55)	14.98(16)	<0.01	5.7(36)	14.19(12)	<0.01
	unbinned	17.4(60)	15.05(14)	<0.01	8.1(40)	14.22(13)	<0.01

Table 1.  Summary of the extracted values for the two resonant-state candidates. The amplitude $\mu_i$ ($i=1,2$) is in unit count, while the mean $E_i$ and width $\Gamma_i$ are in unit MeV.

The fitting results with both background models indicate possible structure around 15 MeV, although the statistical significance are not high, namely 0.8 σ and 1.8 σ, respectively. Estimated widths Γ of the resonance states are very small, less than one-tenth as compared to the experimental resolutions. Therefore, the sensitivity of the natural width in the present analysis is not high. We set the upper limit for the width as 50 keV. The possible states with the second background model have more significance. In addition to the resonance state at 15 MeV, a smaller component around 14 MeV is also suggested.

There are very limited existing data for $ ^{15} {{\rm{C}}}$ at excitation energy above the alpha particle threshold. Bohlen et al. have reported results from a multi-nucleon transfer reaction at Hahn-Meitner Institute populating excited states in $ ^{15} {{\rm{C}}}$ up to above the alpha threshold [33]. Several discrete states were observed in the continuum region. Of particular interest is a state with marked intensity at 14.6 MeV, which was assigned a high spin and parity of 11/2⁺. However, this state is not observed in the present analysis. One possible reason for its absence is the difficulty to populate high-spin state by the inelastic scattering. The same reason applies to the other states suggested in that work. The lead target is usually used to study electromagnetic $ E1 $ strength through the Coulomb excitation induced by virtual photons. The reaction is well described by a semi-classical theory [41], according to which the number of virtual photons decreases rapidly as a function of the beam energy. It is therefore unlikely to populate high excited states in $ ^{15} {{\rm{C}}}$ via the Coulomb excitation. It might be possible, however, to reach such high excited states via nuclear excitation with the lead target acting as an isoscalar probe. Since the spin-parity of the ground state of $ ^{15} {{\rm{C}}}$ is 1/2⁺, one can intuitively regard the two resonance state candidates observed in this work as clustering states with spin-parities 1/2⁺ and 3/2⁺, populated through $ L = 0 $ or 2 transition.

Recently, a systematic study on cluster states in $ ^{14} {{\rm{C}}}$ were reported using the same experimental technique, but with coincident detection of alpha and $ ^{10} {{\rm{Be}}}$ [22]. Several states above the threshold were identified as members of a cluster band, and assigned spin-parities of 0⁺, 2⁺, and 4⁺. Although our statistics are limited and the significance is low, the “peak” positions of our observed candidates are closed to the peak positions of those states in $ ^{14} {{\rm{C}}}$. Naively, the simplest and plausible configurations are that of one neutron in the $ {\rm{s}}_{1/2} $ orbit coupled to those states in $ ^{14} {{\rm{C}}}$. The fact that these states were very weakly populated in the present experiment may be attributed to competition from neutron-decay channels and the energy landscape of the nuclei involved. In the present case of $ ^{15} {{\rm{C}}}$ and its alpha-decay residual $ ^{11} {{\rm{Be}}}$ – a neutron-halo nucleus, the relatively small neutron separation energies ($ S_n $ = 1218.1 keV and 501.64 keV [30], respectively) may play a significant role in hindering the population of these resonance states. It will be interesting to perform a further experiment using a light isoscalar target such as $ ^4 {{\rm{He}}}$ or $ {\rm{CD}}_2 $ with about 10 times more statistics, and/or perform a theoretical calculation to confirm the resonance states.

We have performed an invariant-mass spectroscopy at HIRFL-RIBLL using the $ ^{208} {\rm{Pb}}$($ ^{15} {{\rm{C}}}$, $ ^{15} {{\rm{C}}}$$ ^\star \rightarrow $ $ ^{11} {{\rm{Be}}}$ + $ ^4 {{\rm{He}}}$) reaction to search for possible resonance states in $ ^{15} {{\rm{C}}}$. By detecting the decay alpha particle and residual $ ^{11} {{\rm{Be}}}$ nucleus in coincidence, the excitation energy spectrum for $ ^{15} {{\rm{C}}}$ was reconstructed. To estimate physical background from non-resonant prompt alpha particles, the event-mixing method was applied. Since the prompt alpha's contribution is known to decrease at around the alpha-decay threshold due to the Coulomb final-state interactions, two methods were employed to determine the reduction factor. The first method employed the recipe proposed in Ref. [29]. To provide a robust treatment of the Coulomb effect, in the second method, we determined the reduction factor based on Gamow theory of alpha decay. Fitting the spectrum using the background estimated with these two methods, one and two resonance state candidates have been proposed. A further experiment with a light isoscalar target and with improved statistics, as well as theoretical calculations are called for to confirm these resonance states.

The authors thank the accelerator operators for the stable beams throughout the experiment. S.T. appreciates the fruitful discussion on the Coulomb final-state interactions with S. Koyama.