-
Fission in heavy and superheavy elements can occur either spontaneously or be induced by various particles and photons. Neutron-induced fission has been extensively studied due to its role in nuclear energy generation[1−4]. Proton-induced fission has also been well-documented by Deppman, Ayyad, and Gikal et al. [5−7]. Additionally, heavy energetic nuclei can induced fission through fusion-fission reactions, as demonstrated in various studies [8−11]. Photon-induced fission is another significant area of fission, with contributions from several researchers [12−14].
Since the discovery of fission and its application for nuclear energy production in the late 1940s, nuclear scientists primarily focused on studying binary fission. However, the ternary fission in which a heavy nucleus splits into three fragments emerged as an intriguing source of high-energy alpha particles. This rare type of the nuclear reaction was first identified by Chinese and French scientists, including San-Tsiang, Chastel, and Zah-Wei [15−19]. The cold ternary fission refers to as the disintegrating of an unstable heavy or superheavy nucleus into three fission fragments, neglecting neutrons and other types of radiations [20−31]. This process effectively reveals the behavior of nuclear systems at the scission point.
True ternary fission, where the parent nucleus splits into three fragments with comparable masses, is a rare phenomenon observed in the decay of a certain heavy and superheavy nuclei with high fissility parameters [32, 33]. Although this type of ternary fission has been extensively studied [34−42], its theoretical characteristics are not fully understood yet. True ternary fission typically occurs in heavy nuclei with high fissility parameter (
$Z^2/A > 31$ ) [32].According to the
$ \check{a} $ Sndulescu et al. [43, 44], the cold ternary fission is similar to cluster radioactivity [45, 46], representing a low energy rearrangement of nucleons from the ground state of the initial nucleus to the ground states of three final fragments. Delion et al. [47] provided a quantum description of the cold ternary fission of$ {}^{252}\text{Cf} $ isotope within a stationary scattering formalism, calculating the preformation amplitude for$ ^4\text{He} $ and$ {}^{10}\text{Be} $ light charged particle accompanied formed during the process [44, 48, 49] employed a double-folding potential and$ \text{M3Y} $ nucleon-nucleon forces to study the isotopic yields in the cold ternary fission of$ {}^{248}\text{Cm} $ isotope, without including preformation factors.The recently proposed
$ \text{TCM} $ by Balasubramaniam and Manimaran [50] builds on the preformed cluster model ($ \text{PCM} $ ) introduced by Gupta et al. [53]. This approach has been widely applied to investigate the various aspects of the ternary fission in isotopes of the Californium ($ \text{Cf} $ ), the Uranium ($ \text{U} $ ), the Plutonium ($ \text{Pu} $ ), and the Curium ($ \text{Cm} $ ) [51, 52, 55−58].The competition between the binary and the ternary fission with α-decay in the heavy and the superheavy isotopes has garnered considerable theoretical and experimental interest [46, 47]. A three-body equatorial model was introduced to examine the cold ternary fission of
$ ^{252}\text{Cf} $ isotope, accompanied by α-particles, using an approach including double-folding nuclear potential [43]. Rosen and Hudson [21] provided experimental evidence that the probability of the true ternary fission is significantly lower than the binary fission "approximately$ 6.7 \pm 3 $ per$ 10^6 $ symmetric binary fissions" in the thermal-neutron-induced fission of$ ^{235}\text{U} $ . Green and Livesey [70, 71] utilized photographic plate techniques to measure particle emissions during fission, finding that around 1% of events involved emission of light particle, such as α.Farwell, Segre, and Wiegand [72] conducted detailed studies using coincidence counting method to determine the frequency of the long-range charged particles emission during fission, predominantly α. Manimaran and Balasubramaniam [51, 52] investigated the influence of deformation and orientation on the cold α-particles and
$ {}^{10}\text{B} $ accompanied ternary fission of$ ^{252}\text{Cf} $ isotope. They applied the liquid-drop formalism combined with the Yukawa-plus-exponential nuclear potential and nuclear shape parametrization to determine the ternary-to-binary fission probability ratio using the equatorial geometry [59].Recent observations have identified various isotopes of
$ \text{He} $ ,$ \text{Li} $ ,$ \text{Be} $ , B, and C as light charged particles ($ \text{LCPs} $ ) in the spontaneous ternary fission of$ {}^{252}\text{Cf} $ [55, 56].Building on our previous studies [60, 61], which examined the ternary fission of
$ ^{248}\text{Cf} $ accompanied by$ ^{4}\text{He} $ ,$ ^{10}\text{Be} $ ,$ ^{14}\text{C} $ ,$ ^{14}\text{N} $ , and$ ^{16}\text{O} $ in the equatorial geometry, and the comparison of the results in equatorial and the collinear geometries for the ternary fission of$ ^{244}\text{Fm} $ isotope accompanied by$ ^{8}\text{Be} $ ,$ ^{28}\text{Si} $ ,$ ^{58}\text{Ni} $ , and$ ^{90}\text{Zr} $ , here we explore the fission dynamics Specifically by comparing the equatorial and the collinear geometries, focusing on accompanying heavy fragments with comparable masses in the true ternary fission of the$ ^{248}\text{Cf} $ isotope.The synthesized of superheavy isotopes has been one of the active area of nuclear physics [62]. Californium nucleus is discovered and synthesized in 1950 at Lawrence Berkeley National Laboratory (
$ \text{LBNL} $ ) by bombarding Curium nucleus with α-particles. Its name was after the California state University located at U.S.A. This nucleus has 20 known isotopes that most of them synthesized through successive neutron bombardment and β-decay.$ ^{248}\text{Cf} $ isotope is produced through$ \beta^{-} $ decay of$ ^{248\ast}\text{Bk} $ isotope.The remaining of this paper is organized as follows: Section II describes the theoretical method used to calculate the ternary fission process, focusing on the employed approach. Section III presents the results and provides an in-depth discussion of them. Finally, Section IV concludes the paper and briefly summarized the findings.
-
The ternary fission process closely resembles binary fission, differing primarily in the emission of
$ \text{LCPs} $ during the final stage of the scission process. Study of the ternary fission provides insights not only into fission dynamics but also nuclear structure. Theoretical frameworks have been developed to track$ \text{LCP} $ trajectories, offering tools to analyze statistical and dynamical characteristics of fission especially scission criteria [63, 64].The energy released in the ternary fission (Q-value) is calculated using
$ Q = M - \sum\limits_{i = 1}^{3} m_{i}, $

(1) where M is the mass excess of the parent nucleus, and
$ m_i $ ($ i = 1, 2, 3 $ ) are the mass excess of three fragments in$ \text{MeV} $ unit [65]. A positive Q-value is required for fission to occur, which is shared as kinetic energies of the fragments.The interaction potential between fragments consists of the Coulomb and the nuclear proximity potentials [66, 67]
$ V = \sum\limits_{i = 1}^{3} \sum\limits_{j>i} \left( V^{ij}_c + V^{ij}_p \right). $

(2) The Coulomb potential for interaction of spherical fragments is simply defined as follows,
$ V^{ij}_c = \frac{Z_i Z_j e^2}{R_{ij}}, $

(3) where
$ Z_i $ and$ Z_j $ are atomic numbers, and$ R_{ij} $ is the center-to-center distance between interacting fragments. The effective Radius$ R_x $ for each fragment is defined as,$ R_x = 1.28A_x^{1/3} - 0.76 + 0.8A_x^{-1/3}, $

(4) with
$ A_x $ as the mass number of a fragment labeled by x.The nuclear proximity potential
$ V_p(s) $ is defined by [68]$ V_p(s) = 4\pi \gamma R_\delta \Phi\left(\frac{s}{b}\right), $

(5) where
$ \Phi(\xi) $ depends on the separation$ s/b $ between fragments and is given by [69]$ \Phi(\xi) = \begin{cases} -\dfrac{1}{2}(\xi - 2.54)^2 - 0.0852(\xi - 2.54)^3, & \xi < 1.2511, \\ -3.437 \exp(-\xi/0.75), & \xi \geq 1.2511. \end{cases} $

(6) In the context of the ternary fission, the dimensionless parameter
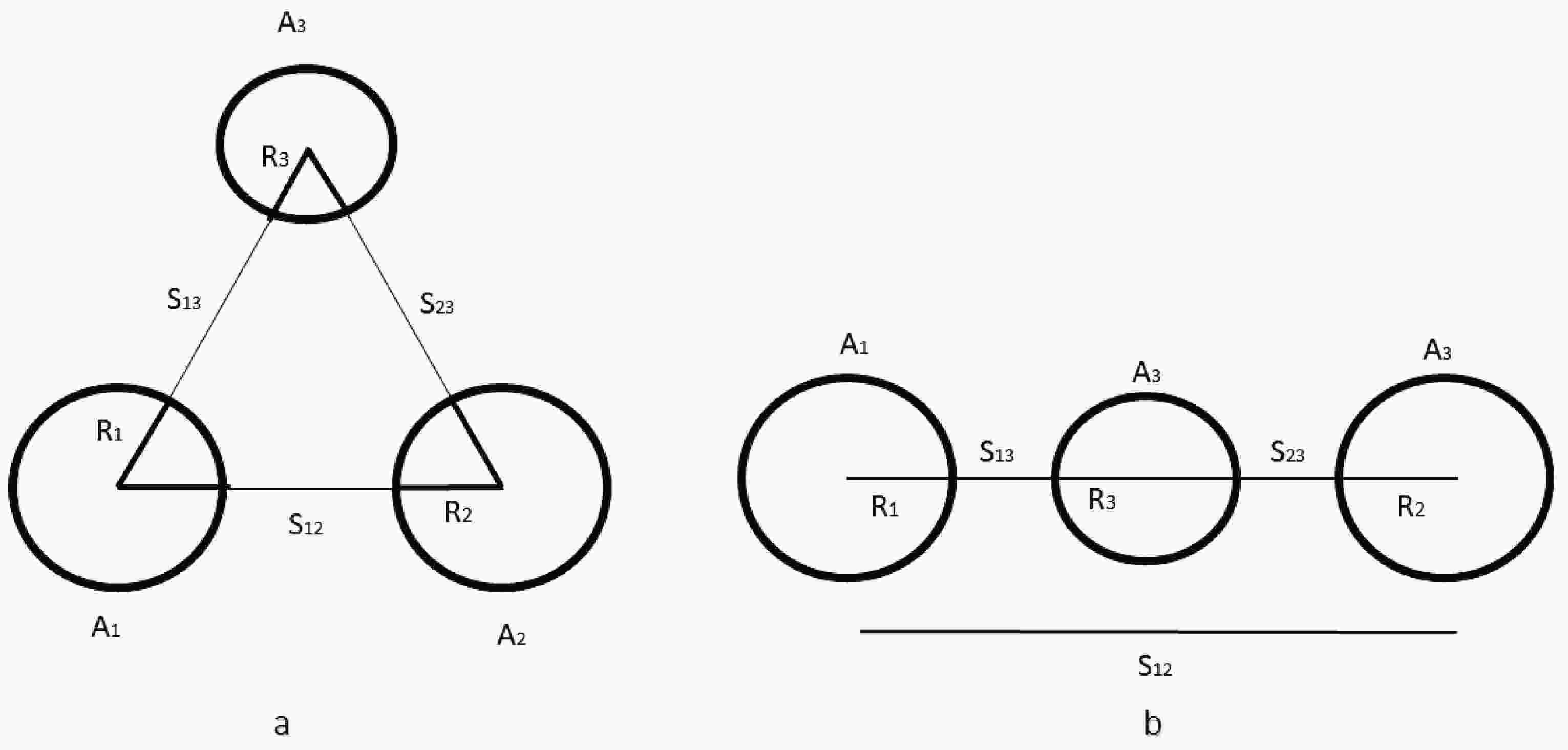
$ \xi = \dfrac{s}{b} $ represents the scaled distance between interacting fragments. For simplicity, it is often assumed that one of three fragments remains fixed during analysis.Geometrical configurations of fragments are key to understand penetration probabilities and fission half-lives. Typically, two types of geometrical configurations of fragments are considered:
● The collinear: Fragments align along a straight line joining the centers of three fragments.
● The equatorial: The fixed
$ LCP $ is ejected perpendicularly to the separation line of other two fragments.These geometrical arrangements of fragments significantly influence the energy distribution among the fragments, their angular momentum, and neutron emission characteristics. In the collinear configuration, the fragments move along a straight line connecting their centers. In contrast, the equatorial configuration involves the fixed light fragment being ejected perpendicular to the line connecting the centers of other two fragments. Figure 1 illustrates the tripartition of fragments in both the equatorial and the collinear geometries, with a separation distance
$ s > 0 $ indicating a moment after separation of fragments.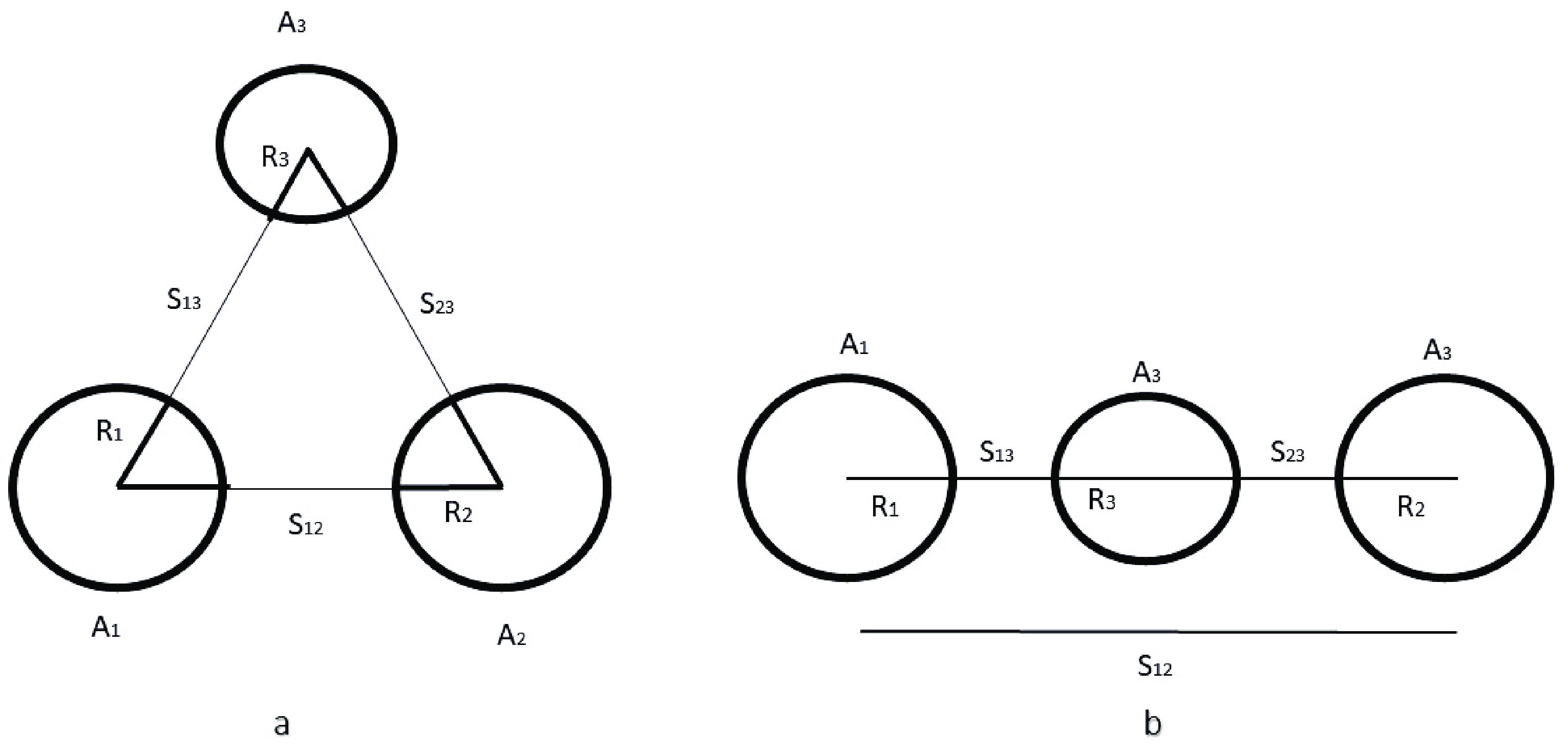
Figure 1. (color online) Schematic diagram of fragment geometries for the ternary fission: The equatorial (a) and the collinear (b) arrangement of fragments just a moment after separation of fragments in output channel (
$s > 0$ ).In the equatorial configuration, a symmetric separation is often assumed for simplicity. Also, it is considered that the fragments disperse with identical speeds and equal separation distances (
$ s = s_{12} = s_{13} = s_{23} $ ). However, this assumption does not account for differences in fragment velocities caused by repulsive Coulomb forces, particularly affecting the lightest fragment ($ A_3 $ , also referred to as the$ \text{LCP} $ , which moves faster than the heavier ones due to conservation of energy principle. To address this discrepancy, an initial relationship$ k \times s_{12} = s_{13} = s_{23} $ can be considered, where k varies within the interval$ 0 < k < 1 $ [50]. Notably, studies indicate that trends in relative yields and fragmentation potential barriers remain largely unaffected by the choice of$ k<1 $ . Therefore, the assumption of$ k = 1 $ not affects considerably on the final results.The parameter S further describes the fragment geometry:
$ S = 0 $ represents a touching configuration,$ S < 0 $ denotes overlapping fragments, and$ S > 0 $ indicates separated fragments configurations. In the collinear geometry, with$ A_3 $ positioned between the other two fragments, the distance between the surfaces of fragments 1 and 3, or 2 and 3, is denoted by$ s = s_{13} = s_{23} $ . The center-to-center distance between fragments 1 and 2 is then given as:$ s_{12} = 2(R_3 + s). $

(7) The penetration probability P is calculated using the
$ \text{WKB} $ approximation$ P = \exp\left(-\frac{2}{\hbar} \int_{S_{\text{in}}}^{S_{\text{out}}} \sqrt{2\mu(V - Q)} \, dS\right), $

(8) where
$ S_{\text{in}} $ and$ S_{\text{out}} $ are the inner and the outer turning points, and μ is the reduced mass of three fragments that obtained using$ \mu = m \frac{A_1 A_2 A_3}{A_1 A_2 + A_1 A_3 + A_2 A_3}. $

(9) Half-life of the ternary fission is evaluated using
$ T_{1/2} = \frac{\ln 2}{\lambda}, \quad \lambda = \nu P, $

(10) where ν is the assault frequency that is simply obtained by the following relation [50],
$ \nu = \frac{\omega}{2\pi} = \frac{2E_\nu}{h}. $

(11) The vibrational energy
$ E_\nu $ is then obtained as follows [?]$ E_\nu = Q \left[0.056 + 0.039\exp\left(\frac{4 - A_{\text{LCP}}}{2.5}\right)\right] \, \text{MeV}. $

(12) Finally, the relative yield
$ Y(A_i, Z_i) $ for each fragmentation with a given geometry is determined using$ Y(A_i, Z_i) = \frac{P(A_i, Z_i)}{\sum P(A_i, Z_i)}. $

(13) The resultant relative yields are used to evaluate the decay constant and the relative half-life.
It is also important to check the neutrons emission in the ternary fission process, which is considered in this research to compare the obtained results with no neutrons emission case of each selected combination.
-
In this study, the ternary fission of
$ ^{248}\text{Cf} $ isotope is analyzed focusing on fragment combinations and their geometries. For the collinear geometries, we systematically examined the driving potential (V) for each fragment configuration where$ A_1 $ ,$ A_2 $ , or$ A_3 $ occupy the middle position in the fragments moving line. Reaction Q-value, penetration probability ($ \text{P} $ ), and decay constant were evaluated for each combination constructed at given geometry for one neutron emission and no neutron emission to highlight the influence of fragment placement and neutrons emission. Among 128 unique sets of$ Z_1 $ ,$ Z_2 $ , and$ Z_3 $ combination, 40 sets of them with the higher penetration probabilities are selected that are tabulated in Table I. The relationship between the Q-value and the atomic numbers$Z_1$ ,$Z_2$ , and$Z_3$ is depicted in Figure 2.Combination Q
(MeV)${\bf{V}} _{e} $ 

(MeV)${\bf{V}} _{A3} $ 

(MeV)${\bf{V}} _{A2} $ 

(MeV)${\bf{V}} _{A1} $ 

(MeV)${\bf{P}} _{e} $ 

${\bf{P}} _{A3} $ 

${\bf{P}} _{A2} $ 

${\bf{P}} _{A1} $ 

ν $ \times 10^{21} $ 

$ \lambda_{\text{e}} $ 

$ \lambda_{\text{A3}} $ 

$ \lambda_{\text{A2}} $ 

$ \lambda_{\text{A1}} $ 

$ ^{50} {\rm{Ca}}$ +

$ ^{64} {\rm{Fe}}$ +

$ ^{134} {\rm{Te}}$ 

244.33 75.40 24.48 4.05 1.24 6.74e-51 2.31e-31 1.41e-21 5.13e-16 6.62 4.5e-29 1.5e-09 9.4e+00 3.4e+06 $ ^{48} {\rm{Ca}}$ +

$ ^{68} {\rm{Ni}}$ +

$ ^{132} {\rm{Sn}}$ 

251.47 75.46 21.12 5.02 0.01 1.63e-49 4.11e-29 2.17e-21 8.37e-15 6.81 1.1e-27 2.8e-07 1.5e+01 5.7e+07 $ ^{48} {\rm{Ca}}$ +

$ ^{67} {\rm{Co}}$ +

$ ^{133} {\rm{Sb}}$ 

245.71 77.92 25.23 6.44 3.73 1.59e-51 2.12e-31 1.98e-22 1.04e-16 6.65 1.1e-29 1.4e-09 1.3e+00 6.9e+05 $ ^{54} {\rm{Ti}}$ +

$ ^{60} {\rm{Cr}}$ +

$ ^{134} {\rm{Te}}$ 

242.42 78.73 27.14 2.67 8.44 5.31e-53 6.32e-33 3.82e-21 1.54e-19 6.57 3.5e-31 4.1e-11 2.5e+01 1.0e+03 $ ^{51} {\rm{Sc}}$ +

$ ^{64} {\rm{Fe}}$ +

$ ^{133} {\rm{Sb}}$ 

244.38 80.14 27.01 6.83 7.04 3.91e-53 1.43e-32 8.96e-23 1.55e-18 6.62 2.6e-31 9.4e-11 5.9e-01 1.0e+04 $ ^{53} {\rm{Sc}}$ +

$ ^{61} {\rm{Mn}}$ +

$ ^{134} {\rm{Te}}$ 

240.28 80.28 28.96 7.10 7.35 4.39e-54 5.80e-34 3.37e-23 3.53e-19 6.51 2.9e-32 3.8e-12 2.2e-01 2.3e+03 $ ^{54} {\rm{Ti}}$ +

$ ^{62} {\rm{Fe}}$ +

$ ^{132} {\rm{Sn}}$ 

248.41 80.42 25.16 6.43 7.11 7.66e-53 1.49e-31 2.68e-22 1.61e-18 6.73 5.2e-31 1.0e-09 1.8e+00 1.1e+04 $ ^{48} {\rm{Ca}}$ +

$ ^{76} {\rm{Zn}}$ +

$ ^{124} {\rm{Cd}}$ 

250.47 80.61 24.78 10.09 3.54 6.09e-53 2.75e-31 2.46e-24 1.06e-16 6.78 4.1e-31 1.9e-09 1.7e-02 7.2e+05 $ ^{48} {\rm{Ca}}$ +

$ ^{73} {\rm{Cu}}$ +

$ ^{127} {\rm{In}}$ 

247.33 81.49 26.68 10.60 5.29 1.36e-53 2.78e-32 1.38e-24 1.53e-17 6.70 9.1e-32 1.9e-10 9.3e-03 1.0e+05 $ ^{54} {\rm{Ti}}$ +

$ ^{61} {\rm{Mn}}$ +

$ ^{133} {\rm{Sb}}$ 

243.65 81.50 28.06 6.46 9.63 4.10e-54 2.86e-33 1.13e-22 5.65e-20 6.60 2.7e-32 1.9e-11 7.5e-01 3.7e+02 $ ^{51} {\rm{Sc}}$ +

$ ^{65} {\rm{Co}}$ +

$ ^{132} {\rm{Sn}}$ 

246.22 81.80 26.93 9.52 7.34 1.28e-53 2.11e-32 9.20e-24 1.55e-18 6.67 8.5e-32 1.4e-10 6.1e-02 1.0e+04 $ ^{48} {\rm{Ca}}$ +

$ ^{82} {\rm{Ge}}$ +

$ ^{118} {\rm{Pd}}$ 

252.27 82.63 24.86 12.97 4.09 5.01e-54 2.30e-31 8.51e-26 5.46e-17 6.83 3.4e-32 1.6e-09 5.8e-04 3.7e+05 $ ^{57} {\rm{V}}$ +

$ ^{58} {\rm{Cr}}$ +

$ ^{133} {\rm{Sb}}$ 

242.59 82.89 29.30 6.26 12.40 4.84e-55 5.33e-34 1.39e-22 2.02e-21 6.57 3.2e-33 3.5e-12 9.1e-01 1.3e+01 $ ^{56} {\rm{Cr}}$ +

$ ^{60} {\rm{Cr}}$ +

$ ^{132} {\rm{Sn}}$ 

245.98 83.64 28.03 4.16 15.44 8.65e-55 4.13e-33 1.56e-21 2.54e-22 6.66 5.7e-33 2.7e-11 1.0e+01 1.7e+00 $ ^{51} {\rm{Sc}}$ +

$ ^{68} {\rm{Ni}}$ +

$ ^{129} {\rm{In}}$ 

246.79 83.78 27.80 11.78 8.32 7.26e-55 5.55e-33 5.52e-25 4.20e-19 6.68 4.8e-33 3.7e-11 3.7e-03 2.8e+03 $ ^{50} {\rm{Ca}}$ +

$ ^{84} {\rm{Se}}$ +

$ ^{114} {\rm{Ru}}$ 

253.00 84.50 24.51 17.49 3.40 1.69e-55 1.37e-31 5.19e-28 4.66e-17 6.85 1.2e-33 9.4e-10 3.6e-06 3.2e+05 $ ^{48} {\rm{Ca}}$ +

$ ^{79} {\rm{Ga}}$ +

$ ^{121} {\rm{Ag}}$ 

248.41 84.69 27.87 14.57 6.84 1.97e-55 5.56e-33 1.27e-26 2.36e-18 6.73 1.3e-33 3.7e-11 8.5e-05 1.6e+04 $ ^{55} {\rm{V}}$ +

$ ^{61} {\rm{Mn}}$ +

$ ^{132} {\rm{Sn}}$ 

244.65 84.76 29.23 8.00 13.98 1.60e-55 8.96e-34 2.93e-23 8.90e-22 6.63 1.1e-33 5.9e-12 1.9e-01 5.9e+00 $ ^{80} {\rm{Ge}}$ +

$ ^{82} {\rm{Ge}}$ +

$ ^{86} {\rm{Se}}$ 

272.69 84.78 8.24 5.25 15.00 8.64e-56 2.43e-24 5.49e-23 2.75e-23 7.38 6.4e-34 1.8e-02 4.1e-01 2.0e-01 $ ^{55} {\rm{V}}$ +

$ ^{59} {\rm{V}}$ +

$ ^{134} {\rm{Te}}$ 

236.51 84.86 33.18 5.96 17.26 5.30e-57 2.31e-36 4.95e-23 7.89e-24 6.40 3.4e-35 1.5e-14 3.2e-01 5.1e-02 $ ^{50} {\rm{Ca}}$ +

$ ^{83} {\rm{As}}$ +

$ ^{115} {\rm{Rh}}$ 

250.73 85.21 26.68 17.00 4.71 3.25e-56 9.67e-33 5.00e-28 8.95e-18 6.79 2.2e-34 6.6e-11 3.4e-06 6.1e+04 $ ^{54} {\rm{Ti}}$ +

$ ^{68} {\rm{Ni}}$ +

$ ^{126} {\rm{Cd}}$ 

248.70 85.26 27.68 11.81 9.88 6.12e-56 3.59e-33 3.32e-25 4.10e-20 6.74 4.1e-34 2.4e-11 2.2e-03 2.8e+02 $ ^{78} {\rm{Zn}}$ +

$ ^{84} {\rm{Se}}$ +

$ ^{86} {\rm{Se}}$ 

271.17 85.66 9.39 10.23 11.92 1.93e-56 5.91e-25 3.16e-25 4.37e-22 7.34 1.4e-34 4.3e-03 2.3e-03 3.2e+00 $ ^{76} {\rm{Zn}}$ +

$ ^{82} {\rm{Ge}}$ +

$ ^{90} {\rm{Kr}}$ 

269.92 86.39 13.00 7.35 13.85 6.79e-57 1.31e-26 4.60e-24 7.02e-23 7.31 5.0e-35 9.6e-05 3.4e-02 5.1e-01 $ ^{50} {\rm{Ca}}$ +

$ ^{90} {\rm{Kr}}$ +

$ ^{108} $ Mo

252.54 86.77 25.10 20.88 4.96 6.19e-57 5.38e-32 8.07e-30 7.04e-18 6.84 4.2e-35 3.7e-10 5.5e-08 4.8e+04 $ ^{54} {\rm{Ti}}$ +

$ ^{82} {\rm{Ge}}$ +

$ ^{112} {\rm{Ru}}$ 

254.03 87.12 25.94 14.68 8.88 2.60e-57 1.53e-32 3.52e-27 6.76e-20 6.88 1.8e-35 1.0e-10 2.4e-05 4.6e+02 $ ^{79} {\rm{Ga}}$ +

$ ^{82} {\rm{Ge}}$ +

$ ^{87} {\rm{Br}}$ 

269.09 87.90 13.33 8.57 16.27 7.60e-58 8.26e-27 1.05e-24 4.01e-24 7.29 5.5e-36 6.0e-05 7.7e-03 2.9e-02 $ ^{80} {\rm{Ge}}$ +

$ ^{83} {\rm{As}}$ +

$ ^{85} {\rm{As}}$ 

269.63 88.00 9.50 10.37 18.16 8.23e-58 3.81e-25 1.99e-25 6.81e-25 7.30 6.0e-36 2.8e-03 1.5e-03 5.0e-03 $ ^{72} {\rm{Ni}}$ +

$ ^{86} {\rm{Se}}$ +

$ ^{90} {\rm{Kr}}$ 

266.93 88.17 15.30 12.65 12.57 4.03e-58 8.39e-28 1.35e-26 2.07e-22 7.23 2.9e-36 6.1e-06 9.7e-05 1.5e+00 $ ^{72} {\rm{Ni}}$ +

$ ^{82} {\rm{Ge}}$ +

$ ^{94} {\rm{Sr}}$ 

265.72 88.21 18.15 10.19 13.10 2.71e-58 3.66e-29 1.51e-25 1.04e-22 7.20 2.0e-36 2.6e-07 1.1e-03 7.5e-01 $ ^{78} {\rm{Zn}}$ +

$ ^{83} {\rm{As}}$ +

$ ^{87} {\rm{Br}}$ 

268.28 88.39 13.96 11.17 14.72 3.26e-58 3.76e-27 6.96e-26 1.57e-23 7.27 2.4e-36 2.7e-05 5.1e-04 1.1e-01 $ ^{79} {\rm{Ga}}$ +

$ ^{84} {\rm{Se}}$ +

$ ^{85} {\rm{As}}$ 

268.92 88.39 10.03 10.45 16.61 4.13e-58 1.96e-25 1.44e-25 2.72e-24 7.28 3.0e-36 1.4e-03 1.0e-03 2.0e-02 $ ^{76} {\rm{Zn}}$ +

$ ^{78} {\rm{Zn}}$ +

$ ^{94} {\rm{Sr}}$ 

265.87 88.66 18.34 7.05 16.88 1.43e-58 2.79e-29 3.81e-24 1.82e-24 7.20 1.0e-36 2.0e-07 2.7e-02 1.3e-02 $ ^{50} {\rm{Ca}}$ +

$ ^{94} {\rm{Sr}}$ +

$ ^{104} {\rm{Zr}}$ 

251.39 88.91 25.07 24.91 6.72 2.98e-58 3.79e-32 8.57e-32 9.20e-19 6.81 2.0e-36 2.6e-10 5.8e-10 6.3e+03 $ ^{75} {\rm{Cu}}$ +

$ ^{83} {\rm{As}}$ +

$ ^{90} {\rm{Kr}}$ 

266.34 89.47 16.30 12.62 14.89 5.77e-59 2.35e-28 1.27e-26 1.28e-23 7.21 4.2e-37 1.7e-06 9.2e-05 9.3e-02 $ ^{77} {\rm{Cu}}$ +

$ ^{85} {\rm{Br}}$ +

$ ^{86} {\rm{Se}}$ 

265.18 90.84 14.94 15.35 15.16 6.06e-60 7.19e-28 5.27e-28 5.49e-24 7.18 4.3e-38 5.2e-06 3.8e-06 3.9e-02 $ ^{72} {\rm{Ni}}$ +

$ ^{83} {\rm{As}}$ +

$ ^{93} {\rm{Rb}}$ 

263.75 90.95 19.08 14.57 15.51 5.90e-60 8.74e-30 1.32e-27 6.48e-24 7.14 4.2e-38 6.2e-08 9.4e-06 4.6e-02 $ ^{72} {\rm{Ni}}$ +

$ ^{87} {\rm{Br}}$ +

$ ^{89} {\rm{Br}}$ 

263.63 91.62 17.01 17.80 15.95 2.34e-60 7.22e-29 4.01e-29 3.82e-24 7.14 1.7e-38 5.2e-07 2.9e-07 2.7e-02 $ ^{66} {\rm{Fe}}$ +

$ ^{70} {\rm{Ni}}$ +

$ ^{112} {\rm{Ru}}$ 

252.15 92.45 29.65 13.45 18.48 2.66e-61 4.18e-35 5.19e-27 3.12e-25 6.83 1.8e-39 2.9e-13 3.6e-05 2.1e-03 $ ^{70} {\rm{Ni}}$ +

$ ^{81} {\rm{Ga}}$ +

$ ^{97} {\rm{Y}}$ 

260.20 92.92 23.90 13.17 19.22 2.51e-61 3.51e-32 3.16e-27 1.33e-25 7.05 1.8e-39 2.5e-10 2.2e-05 9.4e-04 Table 1. Calculated quantities of the ternary fission for 248Cf isotope in different geometries and for selected combinations without neutron emission.
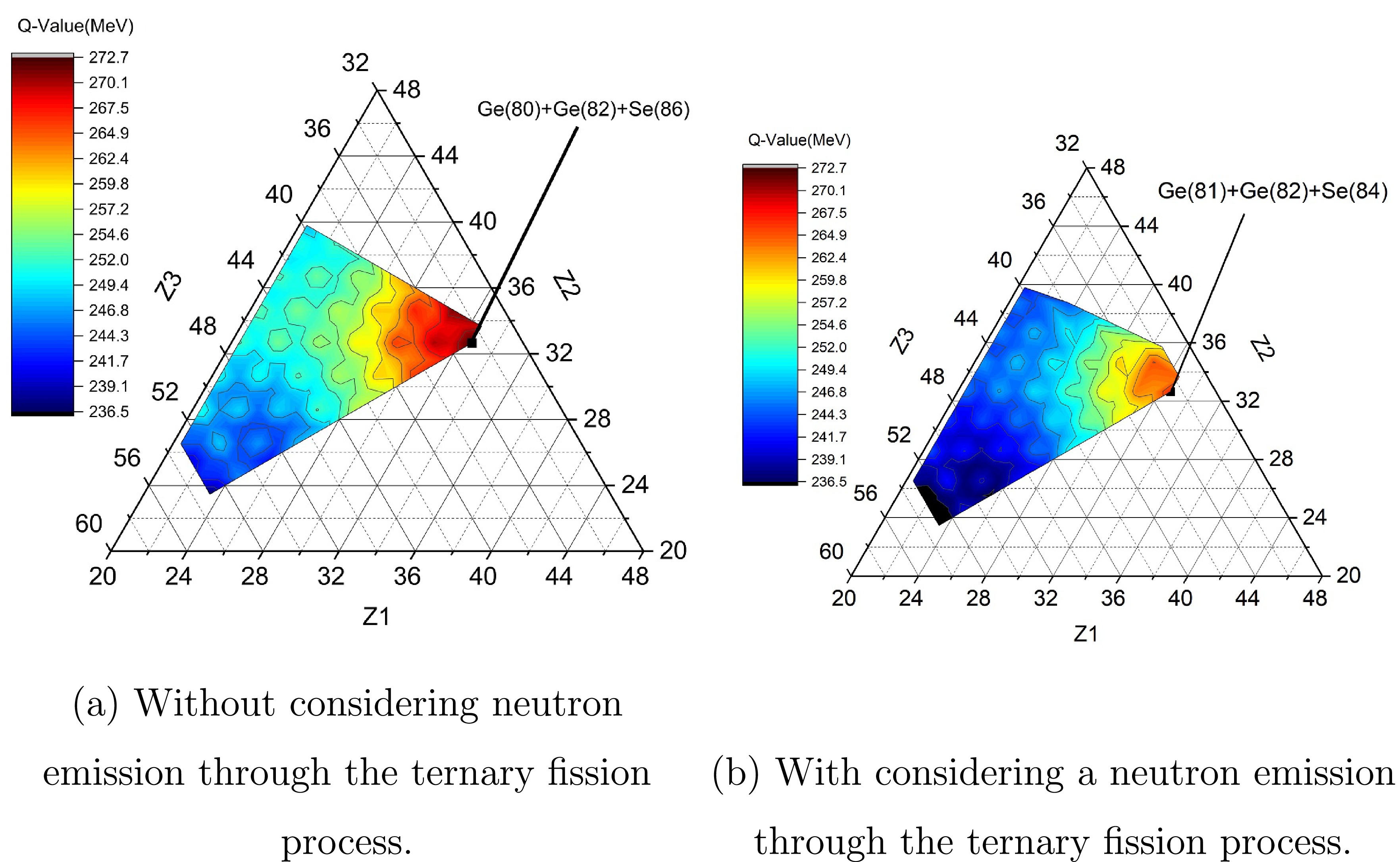
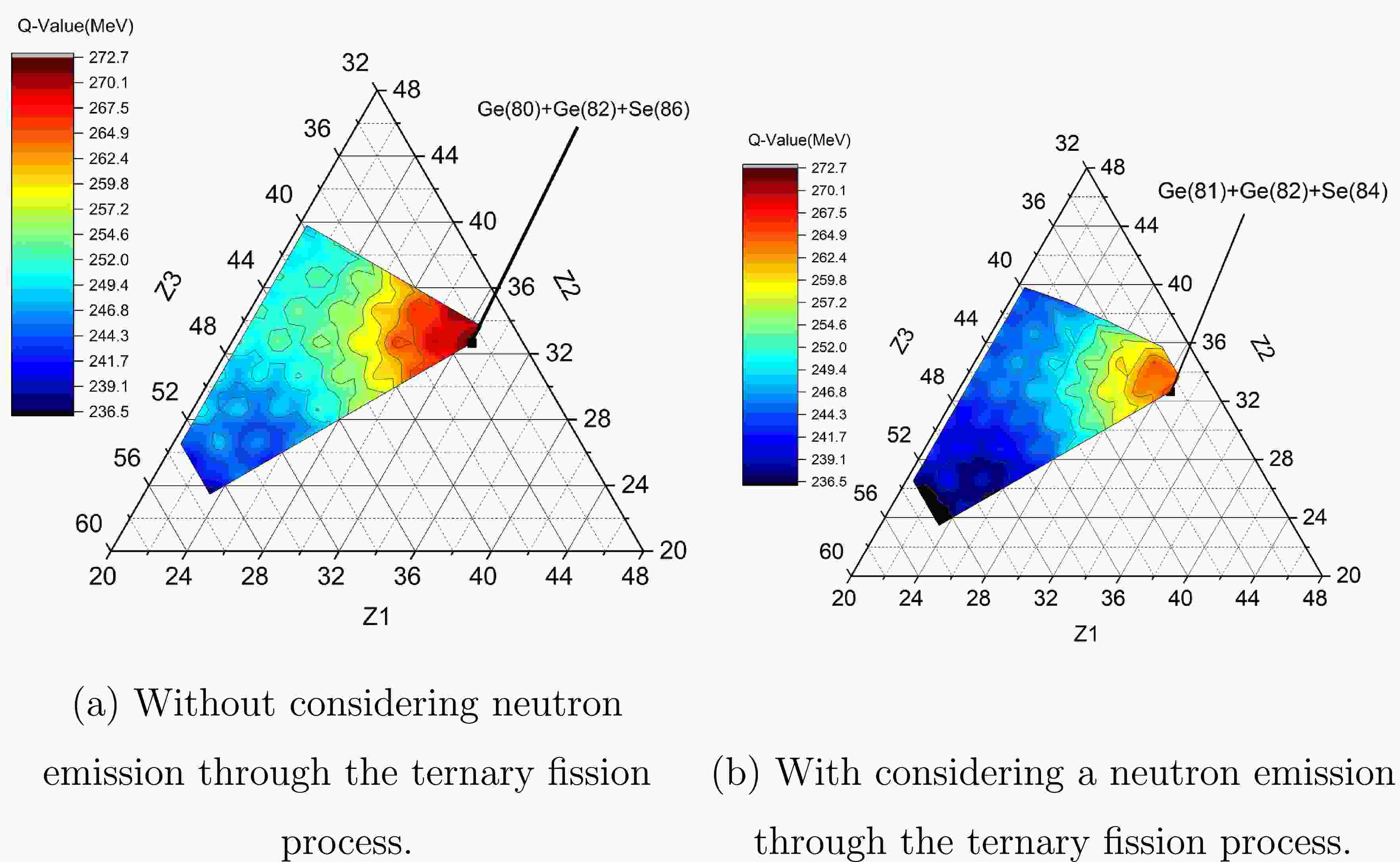
Figure 2. (color online) Q-Value via the fragments atomic numbers
$Z_1$ ,$Z_2$ , and$Z_3$ for the ternary fission of 248Cf isotope.In the collinear configurations with
$ A_3 $ positioned in the middle position of the two other fragments, the alignment of fragments reduces the Coulomb repulsion, resulting in lower driving potentials and higher penetration probabilities. For example, in the combination$ ^{80}\text{Ge} + ^{82}\text{Ge} + ^{86}\text{Se} $ , with a Q-value of 272.69 MeV,$ A_3 $ =$ ^{82}\text{Ge} $ provides significant stabilization due to magic neutron number$ (N = 50) $ . This combination, highlighted in Figure 2, which shows the Q-value as a function of atomic numbers$ Z_1 $ ,$ Z_2 $ , and$ Z_3 $ , exhibits the highest Q-value among all configurations. The calculated driving potential$ (V_{cA3} = 8.23 \, \text{MeV}) $ facilitates a penetration probability of$ 2.43 \times 10^{-24} $ . The even-even and nature of$ ^{80}\text{Ge} $ and$ ^{86}\text{Se} $ further enhance the stability of this configuration. This case is also illustrated in Figure 3c, which presents the collinear geometry with$ A_3 $ in the middle.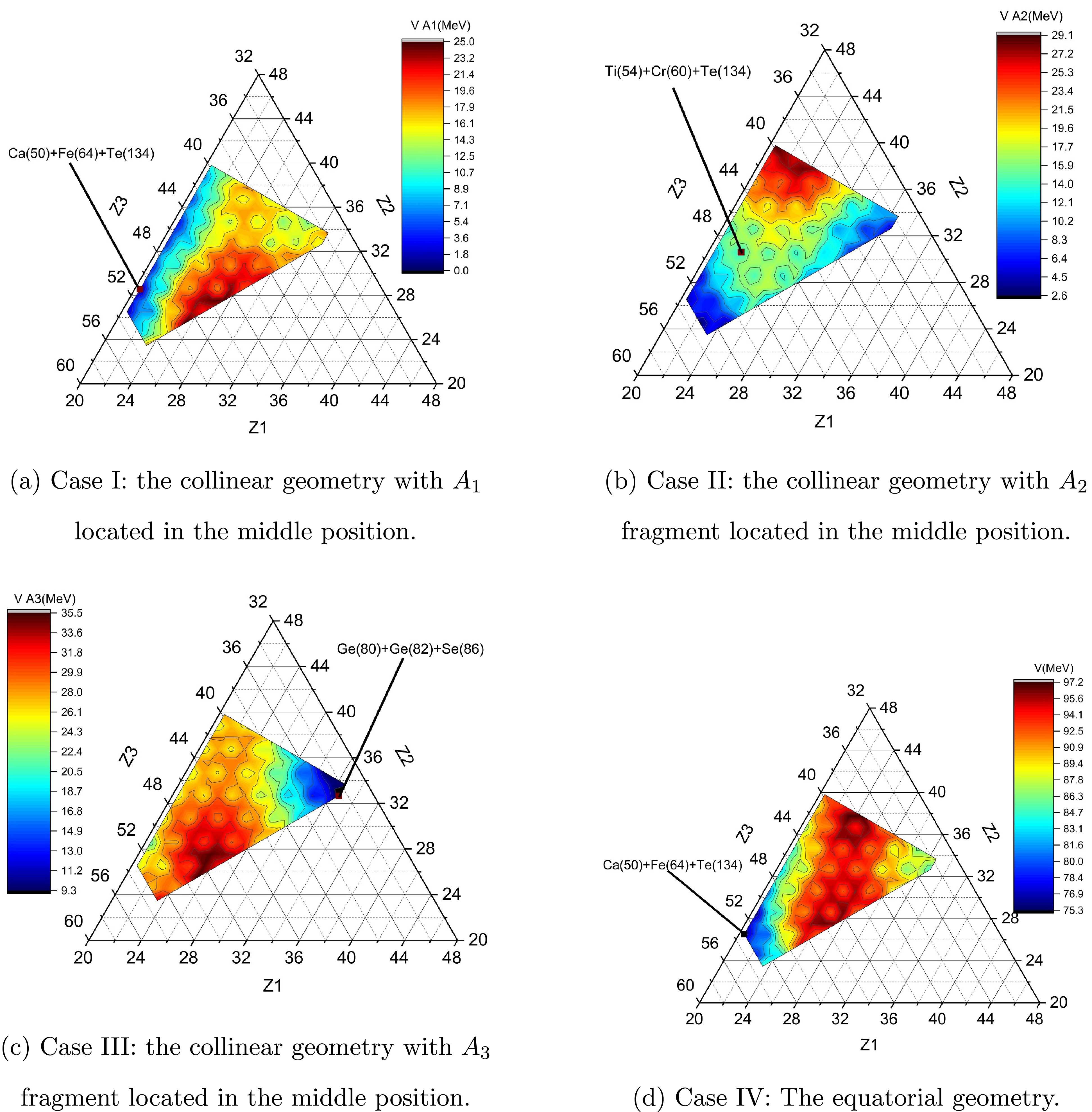
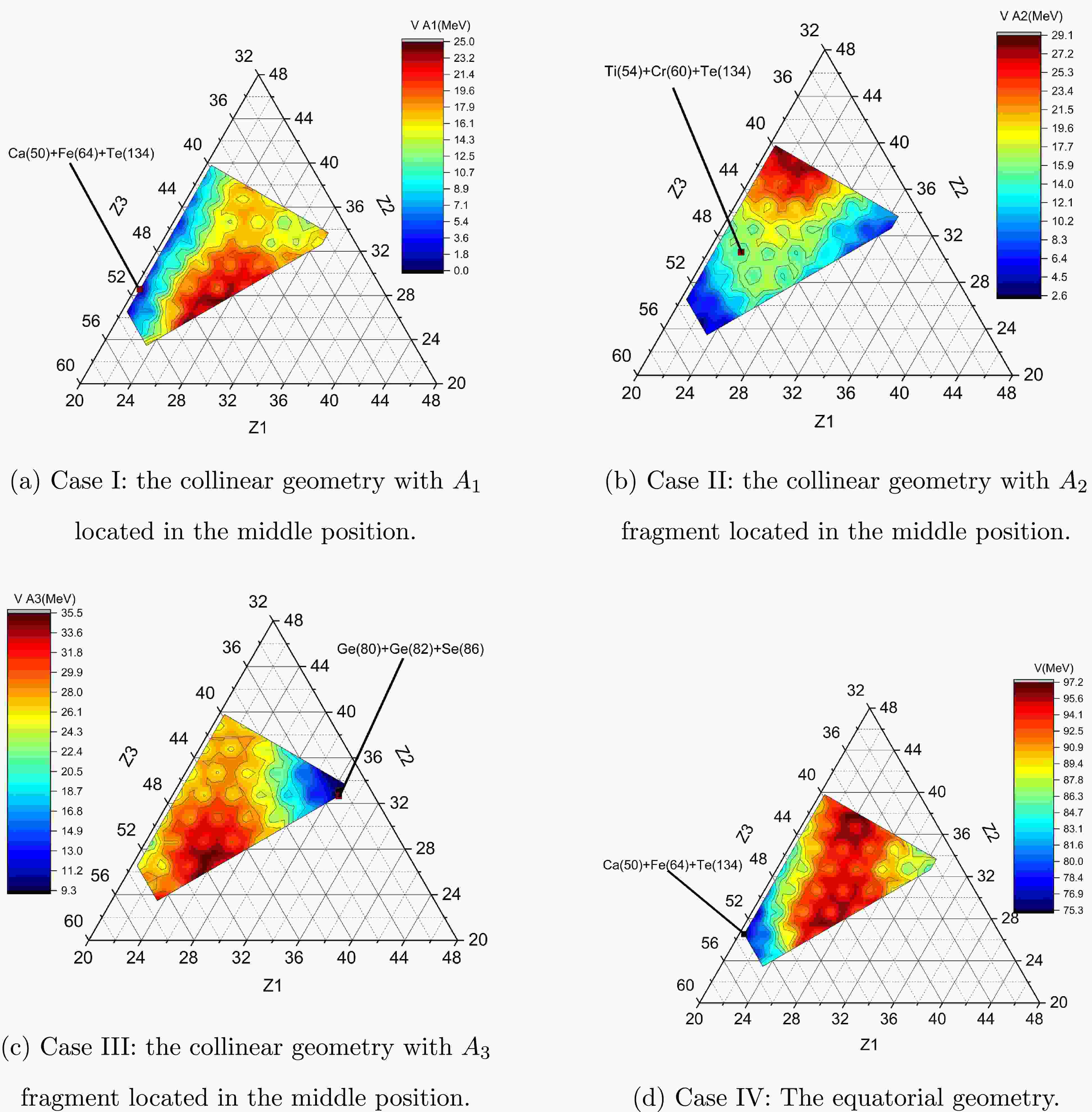
Figure 3. (color online) Driving potential via atomic numbers of the three fragments
$Z_1$ ,$Z_2$ , and$Z_3$ for various cases for the ternary fission of 248Cf isotope.Similarly, in
$ ^{76}\text{Zn} + ^{82}\text{Ge} + ^{90}\text{Kr} $ ,$ A_3 $ =$ ^{82}\text{Ge} $ stabilizes the configuration through magic and near-magic properties of its constituent fragments. The driving potential$ (V_{cA3} = 13.00 \, \text{MeV}) $ is among the lowest observed, leading to a penetration probability of$ 1.31 \times 10^{-26} $ for this combination.When the lightest fragment
$ A_1 $ occupies the middle location, the driving potential decreases due to the lower Coulomb interaction between other two heavier fragments positioned at the ends and lightest fragment occupied in the middle place. For instance, in the combination$ ^{48}\text{Ca} + ^{68}\text{Ni} + ^{132}\text{Sn} $ , where$ A_1 $ =$ ^{48}\text{Ca} $ is placed in the middle, the driving potential$ (V_{cA1} = 0.01 \, \text{MeV}) $ is relatively low. The resultant Q-value equal to 251.47$ \text{MeV} $ and penetration probability equal to$ 8.37 \times 10^{-15} $ are still favorable due to the double-magic nature of$ A_3 $ =$ ^{132}\text{Sn} $ and the even-even near-magic configuration of$ A_2 $ =$ ^{68}\text{Ni} $ . This combination is illustrated in Figure 3a, where$ ^{132}\text{Sn} $ is highlighted due to its double-magic structure.In the combination,
$ ^{50}\text{Ca} + ^{64}\text{Fe} + ^{134}\text{Te} $ , while$ A_1 $ =$ ^{50}\text{Ca} $ occupies the middle position, despite its stabilizing magic property$ (N = 50) $ , the overall driving potential$ (V_{cA1} = 1.24 \, \text{MeV}) $ remains very low due to the even-even nature of$ A_2 $ =$ ^{64}\text{Fe} $ and the magic nature$ N = 82 $ of$ A_3 $ =$ ^{134}\text{Te} $ . Consequently, the penetration probability$ (P = 5.13 \times 10^{-16}) $ is significantly high.When the intermediate-mass fragment
$ A_2 $ is placed in the middle, the driving potential is generally higher than combination when$ A_1 $ located in the middle but lower than when$ A_3 $ occupies middle position. For instance, in$ ^{54}\text{Ti} + ^{60}\text{Cr} + ^{134}\text{Te} $ combination when$ A_2 $ =$ ^{54}\text{Ti} $ positioned in the middle a driving potential$ (V_{cA2} = 2.66 \, \text{MeV}) $ is achived, which is lower than this combination when magic fragment$ A_3 = ^{134}\text{Te} $ is located in the middle. The penetration probability$ (P = 3.82 \times 10^{-21}) $ remains favorable due to the stabilizing effects of$ A_1 = ^{54}\text{Ti} $ and$ A_3 $ =$ ^{134}\text{Te} $ , because both of which are even-even nuclei and one of them contains magic neutron number$ N = 82 $ . This configuration is highlighted in Figure 3b, where the minimum driving potential of combination$ ^{54}\text{Ti} + ^{60}\text{Cr} + ^{134}\text{Te} $ is emphasized.As it is stated in the previous section, we also study one neutron emission in the ternary fission process. In order to check the effects of one neutron emission in the ternary fission process of
$ ^{248} {\rm{Cf}}$ isotope, 10 combinations are selected to make a better comparison of their results with no neutron emission case. The combinations have the same set of atomic numbers (Z), so the elements are the same, but their mass numbers are different. These combinations were chosen because they have the minimum driving potential and the highest penetrating probability among all selected fragments combinations, both with and without considering neutron emission. As it is obvious from the Table 2, the neutron emission reduces the Q-value of the fragments combination. This increase in the potential barrier leads to a lower penetration probability. As an example, for the combination with$ Z_1 = 20 $ ,$ Z_2 = 30 $ , and$ Z_3 = 38 $ , the favored combination is$ \mathrm{^{48}_{20}Ca} + \mathrm{^{76}_{30}Zn} + \mathrm{^{123}_{48}Cd} $ when considering neutron emission, and$ \mathrm{^{48}_{20}Ca} + \mathrm{^{76}_{30}Zn} + \mathrm{^{124}_{48}Cd} $ combination without neutron emission. Their penetrating probability for$ A_1 $ located in the middle is$ 1.62 \times 10^{-20} $ and$ 1.06 \times 10^{-16} $ , respectively. This trend is observed in other geometries and combinations as well.Combination Q
(MeV)${\bf{V}} _{A3} $ 

(MeV)${\bf{V}} _{A2} $ 

(MeV)${\bf{V}} _{A1} $ 

(MeV)${\bf{V}} _{e} $ 

(MeV)${\bf{P}} _{A3} $ 

${\bf{P}} _{A2} $ 

${\bf{P}} _{A1} $ 

${\bf{P}} _{e} $ 

Status $ ^{49} {\rm{Ca}}$ +

$ ^{64} {\rm{Fe}}$ +

$ ^{134} {\rm{Te}}$ 

237.97 31.66 11.15 7.20 82.84 6.35e-35 7.59e-25 1.50e-19 6.07e-55 Considering Neutron Emission $ ^{50} {\rm{Ca}}$ +

$ ^{64} {\rm{Fe}}$ +

$ ^{134} {\rm{Te}}$ 

244.33 24.48 4.05 1.24 75.40 2.31e-31 1.41e-21 5.13e-16 6.74e-51 Without Neutron Emission $ ^{48} {\rm{Ca}}$ +

$ ^{68} {\rm{Ni}}$ +

$ ^{131} {\rm{Sn}}$ 

244.12 29.18 12.83 5.94 83.45 3.16e-33 3.79e-25 1.72e-18 3.30e-54 Considering Neutron Emission $ ^{48} {\rm{Ca}}$ +

$ ^{68} {\rm{Ni}}$ +

$ ^{132} {\rm{Sn}}$ 

251.47 21.12 5.02 0.01 75.46 4.11e-29 2.17e-21 8.37e-15 1.63e-49 Without Neutron Emission $ ^{48} {\rm{Ca}}$ +

$ ^{76} {\rm{Zn}}$ +

$ ^{123} {\rm{Cd}}$ 

243.11 32.88 17.93 9.50 88.64 1.58e-35 3.12e-28 1.62e-20 8.10e-58 Considering Neutron Emission $ ^{48} {\rm{Ca}}$ +

$ ^{76} {\rm{Zn}}$ +

$ ^{124} {\rm{Cd}}$ 

250.47 24.78 10.09 3.54 80.61 2.75e-31 2.46e-24 1.06e-16 6.09e-53 Without Neutron Emission $ ^{50} {\rm{Sc}}$ +

$ ^{64} {\rm{Fe}}$ +

$ ^{133} {\rm{Sb}}$ 

237.60 34.62 14.36 13.42 88.02 2.03e-36 2.83e-26 2.91e-22 1.52e-57 Considering Neutron Emission $ ^{51} {\rm{Sc}}$ +

$ ^{64} {\rm{Fe}}$ +

$ ^{133} {\rm{Sb}}$ 

244.38 27.01 6.83 7.04 80.14 1.43e-32 8.96e-23 1.55e-18 3.91e-53 Without Neutron Emission $ ^{53} {\rm{Ti}}$ +

$ ^{62} {\rm{Fe}}$ +

$ ^{132} {\rm{Sn}}$ 

241.47 32.89 14.10 13.58 88.42 1.94e-35 7.64e-26 2.59e-22 2.54e-57 Considering Neutron Emission $ ^{54} {\rm{Ti}}$ +

$ ^{62} {\rm{Fe}}$ +

$ ^{132} {\rm{Sn}}$ 

248.41 25.16 6.43 7.11 80.42 1.49e-31 2.68e-22 1.61e-18 7.66e-53 Without Neutron Emission $ ^{54} {\rm{Ti}}$ +

$ ^{59} {\rm{Cr}}$ +

$ ^{134} {\rm{Te}}$ 

235.56 34.77 10.79 14.13 86.60 6.69e-37 6.63e-25 4.05e-23 1.48e-57 Considering Neutron Emission $ ^{54} {\rm{Ti}}$ +

$ ^{60} {\rm{Cr}}$ +

$ ^{134} {\rm{Te}}$ 

242.42 27.14 2.67 8.44 78.73 6.32e-33 3.82e-21 1.54e-19 5.31e-53 Without Neutron Emission $ ^{57} {\rm{Cr}}$ +

$ ^{58} {\rm{Cr}}$ +

$ ^{132} {\rm{Sn}}$ 

240.23 34.5005 11.767 12.38 90.36 2.07e-36 6.91e-25 4.42e-25 1.29e-57 Considering Neutron Emission $ ^{56} {\rm{Cr}}$ +

$ ^{60} {\rm{Cr}}$ +

$ ^{132} {\rm{Sn}}$ 

245.98 28.026 4.158 17.59 83.637 4.13e-33 1.57e-21 2.54e-22 8.65e-55 Without Neutron Emission $ ^{70} {\rm{Ni}}$ +

$ ^{81} {\rm{Ga}}$ +

$ ^{96} {\rm{Y}}$ 

254.34 30.70 19.59 23.79 99.58 1.13e-35 1.79e-30 8.57e-29 1.79e-65 Considering Neutron Emission $ ^{70} {\rm{Ni}}$ +

$ ^{81} {\rm{Ga}}$ +

$ ^{97} {\rm{Y}}$ 

260.20 23.90 13.17 19.22 92.92 3.51e-32 3.16e-27 1.33e-25 2.51e-61 Without Neutron Emission $ ^{70} {\rm{Ni}}$ +

$ ^{82} {\rm{Ge}}$ +

$ ^{95} {\rm{Sr}}$ 

258.91 25.35 17.72 19.84 96.08 8.74e-33 3.48e-29 1.20e-26 7.65e-63 Considering Neutron Emission $ ^{72} {\rm{Ni}}$ +

$ ^{82} {\rm{Ge}}$ +

$ ^{94} {\rm{Sr}}$ 

265.72 18.15 10.19 13.10 88.21 3.66e-29 1.51e-25 1.04e-22 2.71e-58 Without Neutron Emission $ ^{78} {\rm{Zn}}$ +

$ ^{84} {\rm{Se}}$ +

$ ^{85} {\rm{Se}}$ 

265.01 16.58 17.00 16.82 92.68 2.16e-28 1.58e-28 2.30e-25 1.52e-60 Considering Neutron Emission $ ^{78} {\rm{Zn}}$ +

$ ^{84} {\rm{Se}}$ +

$ ^{86} {\rm{Se}}$ 

271.17 9.39 10.23 11.92 85.66 5.91e-25 3.16e-25 4.37e-22 1.93e-56 Without Neutron Emission Table 2. Comparison of calculated quantities for the ternary fission of 248Cf isotope with considering a neutron emission in the fission process for the selected combinations.
As a second example, we consider the
$ \mathrm{Cr-Cr-Sn} $ combinations. The selected configurations are$ \mathrm{^{57}_{24}Cr} + \mathrm{^{58}_{24}Cr} + \mathrm{^{132}_{50}Sn} $ and$ \mathrm{^{56}_{24}Cr} + \mathrm{^{60}_{24}Cr} + \mathrm{^{132}_{50}Sn} $ , respectively for with neutron and without neutron emission. The driving potential is$ 34.50 $ $ \text{MeV} $ for$ A_3 $ placed in the middle, is$ 11.767 $ $ \text{MeV} $ for$ A_2 $ positioned in the middle, and equal to$ 12.38 $ $ \text{MeV} $ for$ A_1 $ occupied in the middle for neutron emission case. Respectively, they are$ 28.026 $ $ \text{MeV} $ ,$ 4.158 $ $ \text{MeV} $ , and$ 17.59 $ $ \text{MeV} $ for without neutron emission case. The double-magic$ \mathrm{^{132}_{50}Sn} $ isotope plays a crucial role in these combinations due to its neutron and proton closure shell, making them the most favorable set of combinations.The equatorial geometry, in contrast, shows consistently higher driving potentials and lower penetration probabilities due to the increased Coulomb repulsion among symmetrically placed fragments. For example, in
$ ^{50}\text{Ca} + ^{64}\text{Fe} + ^{134}\text{Te} $ combination, the driving potential in the equatorial geometry has value$ (V_{c} = 75.39 \, \text{MeV}) $ significantly higher than ones in the collinear geometry, reducing the penetration probability by several orders of magnitude. This behavior is summarized in Figure 3d, which highlights the magic combination$ ^{50}\text{Ca} + ^{64}\text{Fe} + ^{134}\text{Te} $ .Overall, in a given combination, the placement of fragments has a notable effect on driving potentials and penetration probabilities. While the collinear geometries are generally more favorable, the fixed fragment positioned in the middle point and its stability property influence the configuration likelihood of the ternary fission.
-
In this study, the influence of fragments geometry and nuclear structure on penetration probabilities, driving potentials, and stability in the ternary fission of the
$ ^{248} {\rm{Cf}}$ isotope are investigated. The results underscore the critical role of fragment placement in determining fission characteristics, particularly highlighting differences between the collinear and the equatorial fragments geometries.Across all configurations, the positioning of fragments significantly affects the driving potential and penetration probabilities. When the heaviest fragment,
$ A_3 $ , is placed in the middle position (Case III), the configurations exhibit the highest driving potential and the lowest penetration probability, resulting in greater stability and half-life. This is reflected in the notably longer half-life of$ 4.577 \times 10^{15} $ seconds, as shown in the Table 3. When we consider neutron emission during the ternary fission process, the half-life is even longer, at$ 4.81 \times 10^{19} $ seconds. Fragmentation channels involving magic or near-magic fragments, such as$ ^{80} {\rm{Ge}}$ +$ ^{82} {\rm{Ge}}$ +$ ^{86} {\rm{Se}}$ and$ ^{78} {\rm{Zn}}$ +$ ^{84} {\rm{Se}}$ +$ ^{86} {\rm{Se}}$ combinations, further support this enhancement of stability, as highlighted in Figure 4c.Geometry Half-life (s) (Without neutron emission) Half-life (s) (Considering a neutron emission) The equatorial $1.04 \times 10^{42}$ 

$4.18 \times 10^{40}$ 

The collinear ( $A_{1}$ placed in the middle)

$8.100 \times 10^{5}$ 

$3.58 \times 10^{9}$ 

The collinear ( $A_{2}$ placed in the middle)

$1.003 \times 10^{12}$ 

$1.43 \times 10^{16}$ 

The collinear ( $A_{3}$ placed in the middle)

$4.577 \times 10^{15}$ 

$4.81 \times 10^{19}$ 

Table 3. Calculated half-lives (in seconds) for the ternary fission of 248Cf isotope in different geometries with and without a neutron emission through fission process.
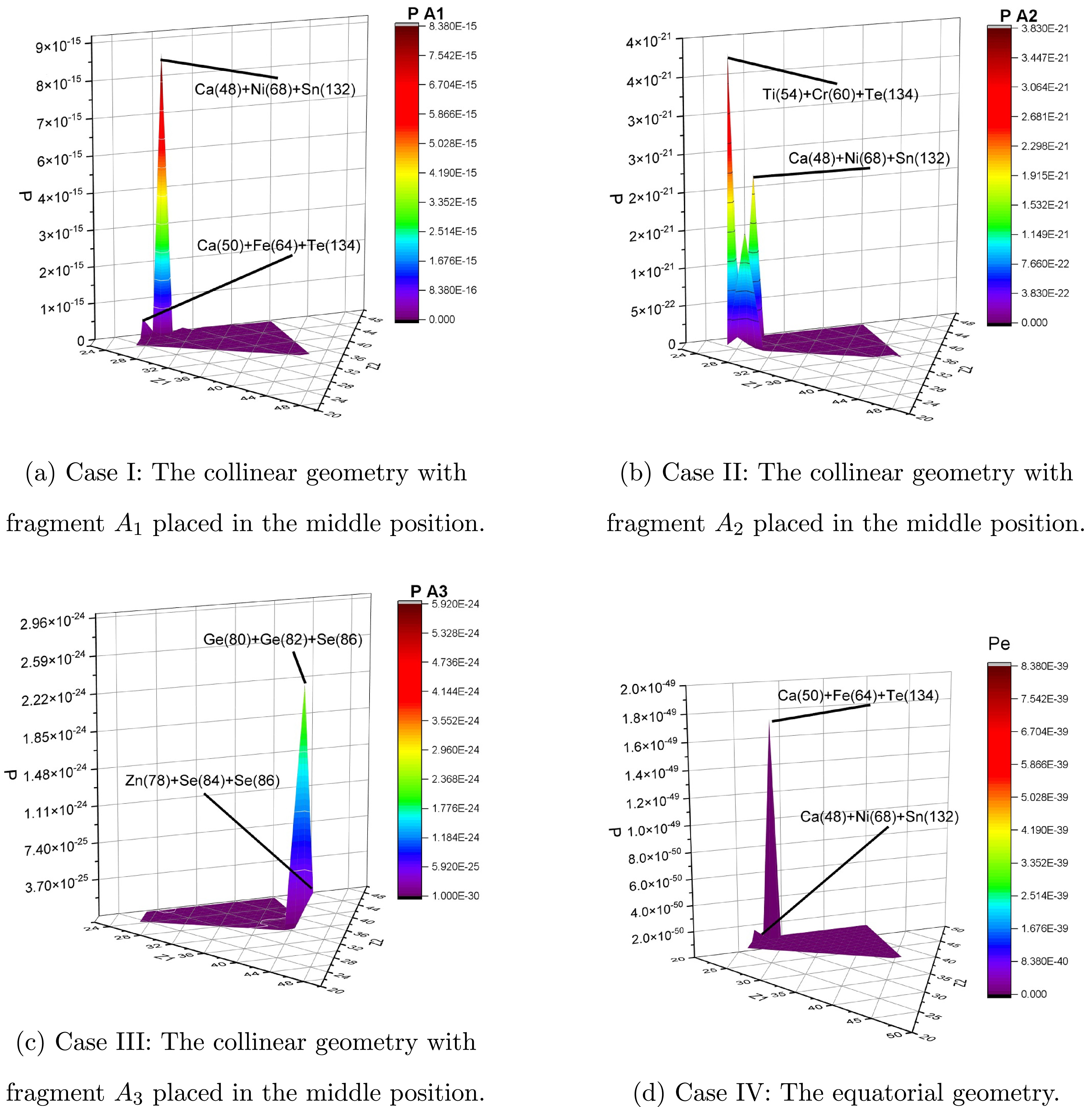
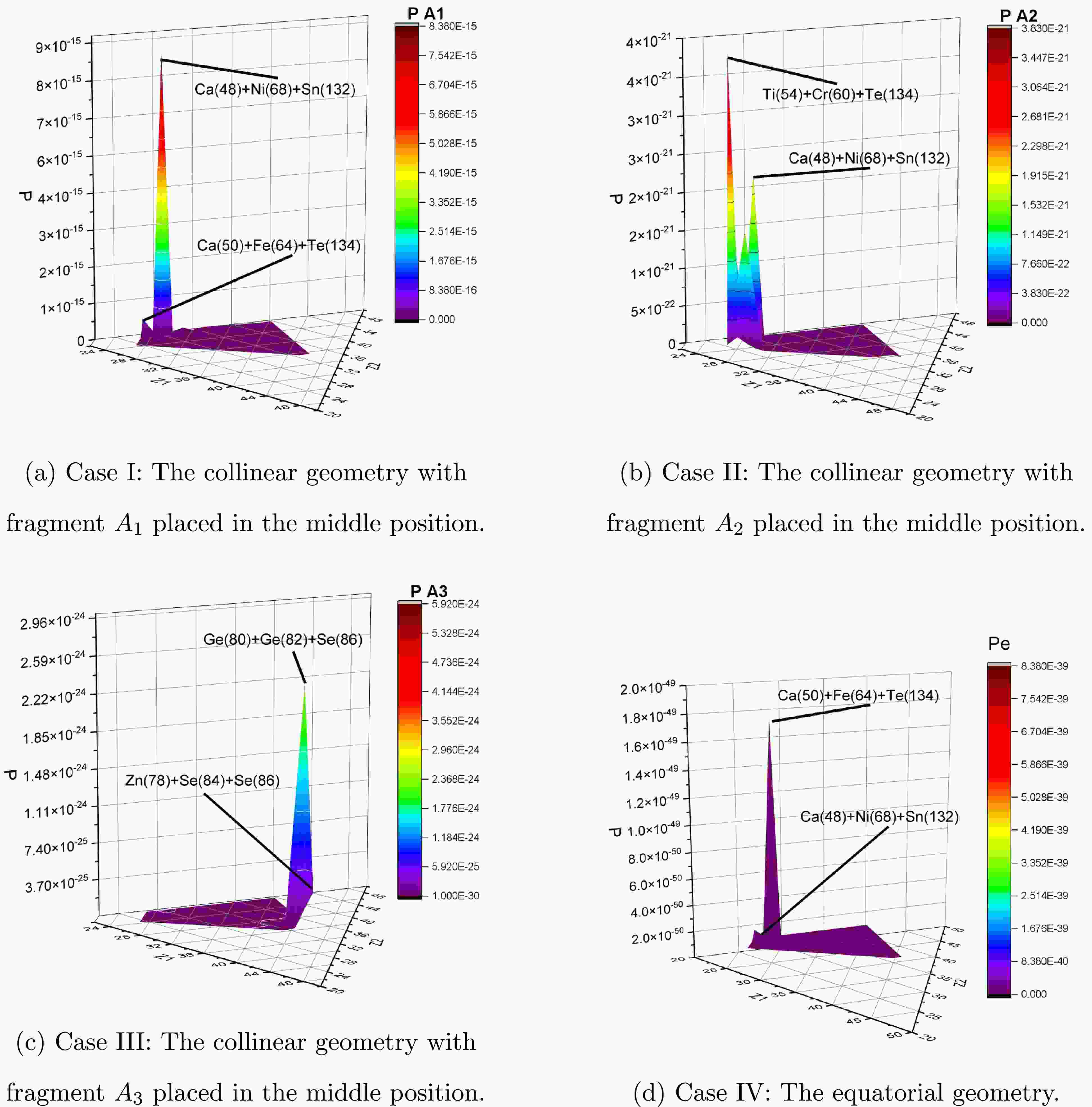
Figure 4. (color online) Penetrating probability via the atomic numbers of three fragments
$Z_1$ ,$Z_2$ , and$Z_3$ in various geometries for the ternary fission of 248Cf isotope.In contrast, placing the lightest fragment,
$A_1$ , in the middle position (Case I, Figure 4a) results in lower driving potentials, making these configurations more favorable. This is evidenced by their relatively short half-life of$8.100 \times 10^{5}$ seconds. When we consider neutron emission, the half-life increases to$ 3.58 \times 10^{9} $ seconds. Similar trends have been reported in previous studies, such as those by Vijayaraghavan et al. [33, 57] and Karpov et al. [73], where the central placement of the lightest fragment was shown to minimize the driving potential and maximize penetration probability due to the decrease in the Coulomb repulsion. These works serve as examples that corroborate our findings. Moreover, combinations such as$^{48}{\rm{Ca}}$ +$^{68}{\rm{Ni}}$ +$^{132}{\rm{Sn}}$ and$^{50}{\rm{Ca}}$ +$^{64}{\rm{Fe}}$ +$^{134}{\rm{Te}}$ presented in this study further exhibit high stability due to the presence of double-magic fragments like$^{132}{\rm{Sn}}$ .Configurations with
$ A_2 $ located in the middle (Case II, Figure 4b) exhibit intermediate behavior, with driving potentials and penetration probabilities influenced by the structural properties of the fragments. This is evidenced by its calculated half-life of$ 1.00339 \times 10^{12} $ seconds when considering neutron emission and$ 1.43 \times 10^{16} $ seconds when not considering neutron emission. Examples include$ ^{54}\text{Ti} $ +$ ^{60}\text{Cr} $ +$ ^{134}\text{Te} $ and$ ^{48}\text{Ca} $ +$ ^{68}\text{Ni} $ +$ ^{132}\text{Sn} $ combinations, illustrating the stabilizing effects due to magic, near-magic, and even-even structure of fragments.By considering neutron emission, the reduction of Q-value leading to an increase in the driving potential across all geometries. Consequently, the penetration probability decreases. Conversely, the geometry exhibits the same effect as when we do not consider neutron emission—the lighter the middle fragment, the higher the penetration probability. Additionally, the structural effects in the favored combinations, such as magic or near-magic shells, play a significant role in determining the most favorable sets of combinations. As we see in all collinear cases by considering neutron emission, the structural effects are critical in influencing the stability and penetrability of the configurations.
The equatorial geometry (Case IV, Figure 4d) displays distinct behavior, with the longest calculated half-life of
$ 1.04 \times 10^{42} $ seconds, indicating exceptional stability. Combinations such as$ ^{50}\text{Ca} $ +$ ^{64}\text{Fe} $ +$ ^{134}\text{Te} $ and$ ^{48}\text{Ca} $ +$ ^{68}\text{Ni} $ +$ ^{132}\text{Sn} $ , benefit from the presence of even-even, magic and double-magic fragments, which further enhance their stability.Overall, our analysis reveal that the collinear fragments geometry is consistently more favorable than the equatorial fragmentation for true ternary fission, particularly when the middle fragment exhibits magic or near-magic properties. The placement of fragments and their nuclear structure critically influence barrier characteristics, penetration probabilities, and overall fission dynamics. These insights provide a deeper understanding of the mechanisms governing the ternary fission and emphasize the importance of structural configurations in predicting the half-life of the ternary fission and its stability.
Study of the true ternary fission for 248Cf isotope in the equatorial and the collinear geometries
- Received Date: 2025-01-10
- Available Online: 2025-08-01
Abstract: This study presents a comprehensive investigation of the true ternary fission for





 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF


























 DownLoad:
DownLoad: